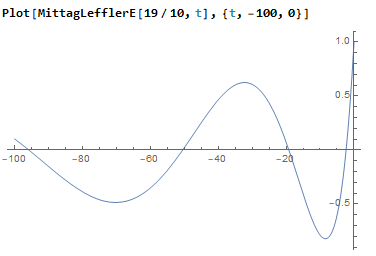
Let the Mittaq-Leffler function be defined by the expression $$ E_{\mu,\nu}(z) = \sum_{k=0}^{\infty} \frac{z^k}{\Gamma(k\mu+\nu)}\quad \text{$\mu>0$ and $\nu\in \mathbb R$}$$
Now let $n\in \mathbb N$, $\alpha \in (\frac{n-1}{n},1)$. My question is whether the Mittag-Leffler function $E_{n\alpha,1}(t)$ is non vanishing for all $t<0$ or not.
The answer is no. E.g., for $n=2$ and $\alpha=19/20$, we get the following:
The idea of this example is based on the identity $E_2(-z^2)=E_{2,1}(-z^2)=\cos z$ and the continuity of $E_{a,1}(t)$ in $a$.
More counterexamples can be obtained from the asymptotics $$E_{a,1}(t)\sim-\frac1{\Gamma(1-a)t}=-\frac{\Gamma(1+a)}{\pi at}\,\sin\pi a$$ as $t\to-\infty$ for $a\in\bigcup_{n=1}^\infty(n-1,n)$ and the equality $E_{a,1}(0)=1$.
