I am considering the ODE
$$y'(x) = \begin{pmatrix} 0 & 1-\sin(x-\frac{\pi}{4})\\ 1 - \sin(x+\frac{\pi}{4}) & 0 \end{pmatrix} y(x).$$
My question is: Can we find an explicit solution to this ODE? A solution clearly exists, but I am wondering if one can find it explicitly?
Partial answer: the system reduces to a Riccati equation.
We have $$ \begin{aligned} y_1'&=(1-\sin(x-\frac\pi4))y_2,\\ y_2'&=(1-\sin(x+\frac\pi4))y_1. \end{aligned} $$ The function $z=y_1/y_2$ satisfies $$ z'=y_1'/y_2-y_1y_2'/y_2^2=1-\sin(x-\frac\pi4)-(1-\sin(x+\frac\pi4))z^2. $$ Once $z$ is found $y_1$ and $y_2$ can be found from it in terms of antiderivatives: $$ \begin{aligned} (\log y_1)'&=(1-\sin(x-\frac\pi4))/z\\ (\log y_2)'&=(1-\sin(x+\frac\pi4))z. \end{aligned} $$ As for the Riccati equation, if desired, it can be made algebraic (in fact, with rational function coefficients): the substitution $\tan(\frac{x-\pi/4}2)=t$ gives $$ \frac{dz}{dt}=2 \left(\frac{1-t}{1+t^2}\right)^2-\left(\frac{2tz}{1+t^2}\right)^2 $$ It can be also turned into a second order linear equation. Beyond that, I don't know. I would say, it is sort of generic kind of a Riccati equation.
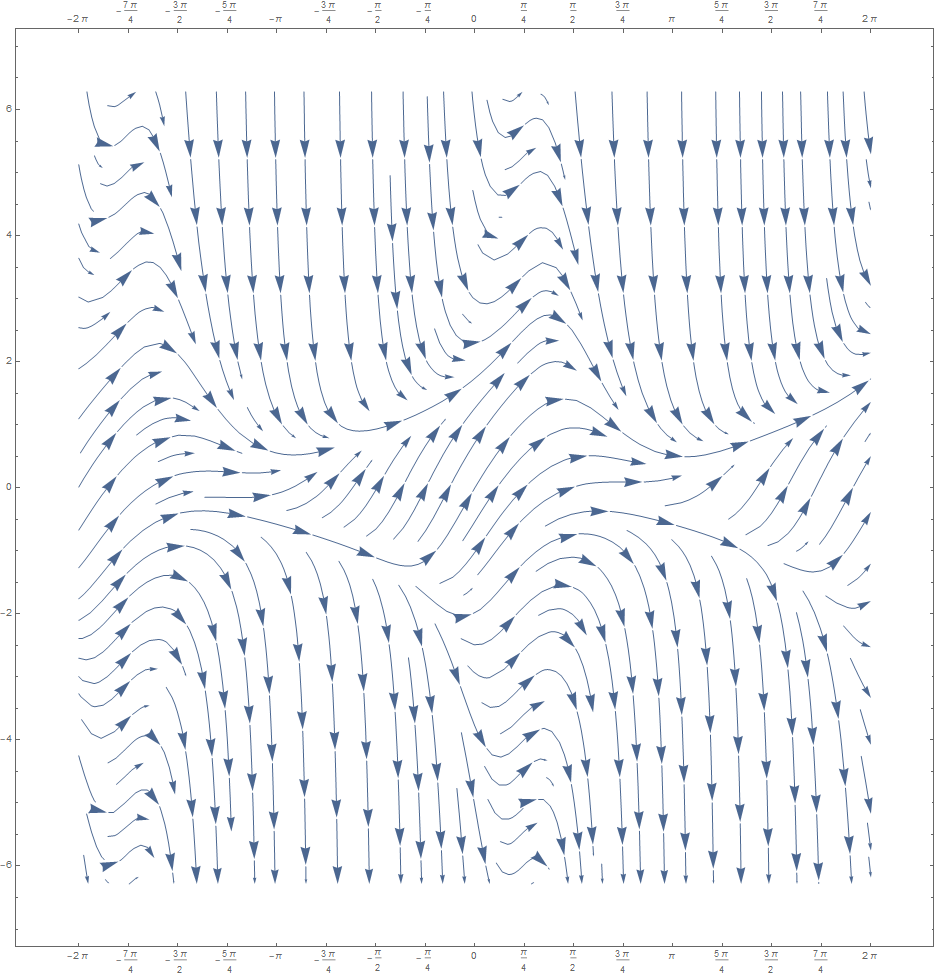
To look at the qualitative picture, here is a stream plot (from Mathematica) in the $(x,z)$-plane
