It is a simple fact, the granddaddy of correlation inequalities that if $f,g$ are monotone functions on $[0,1]$ then $$\int_0^1 f(x)g(x) dx \ge \int_0^1 f(x) dx \int_0^1 g(x) dx.$$ In their famous inequalities book, Hardy-Littlewood-Polya say this is due to Chebyshev and I even found an article calling this "Chebyshev's other inequality". But I haven't found a reference to a paper of Chebyshev where this appears. Does anyone know such a reference? (I want it because I am writing a book with a chapter on correlation inequalities). Many thanks.
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
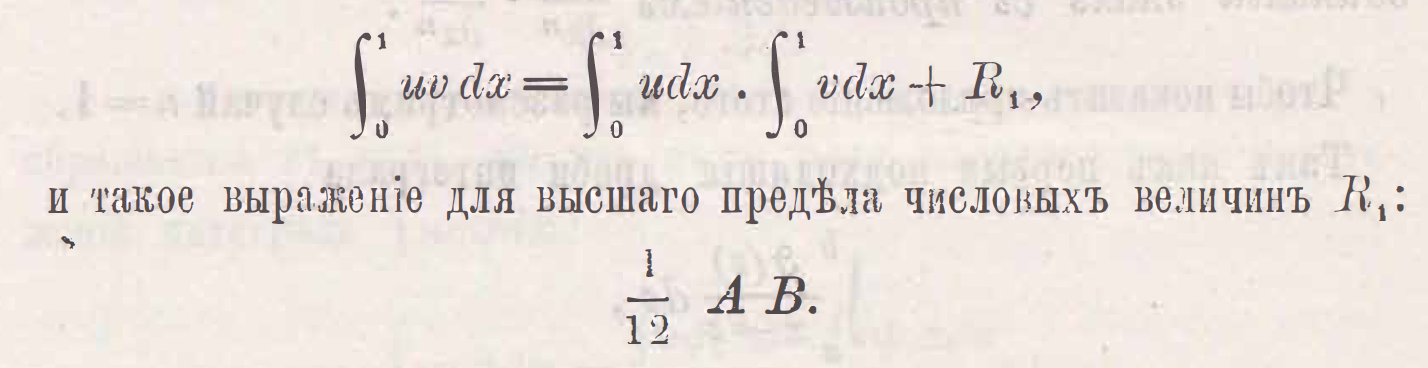$\endgroup$
3
The source for the inequality is
P.L. Chebyshev, On approximate expressions of some integrals in terms of others, taken within the same limits, Proc. Math. Soc. Kharkov 2, 93–98 (1882).
This article is online here. The relevant text is copied below, which presumably states $R_1>0$, hopefully someone who reads Russian can provide a translation.
Google translate suggests that $R_1=AB/12$ with $A,B$ the maximum of the derivatives of $du/dx$, $dv/dx$, which as noted by Emil Jeřábek cannot be quite correct.
answered Dec 13, 2020 at 12:52
-
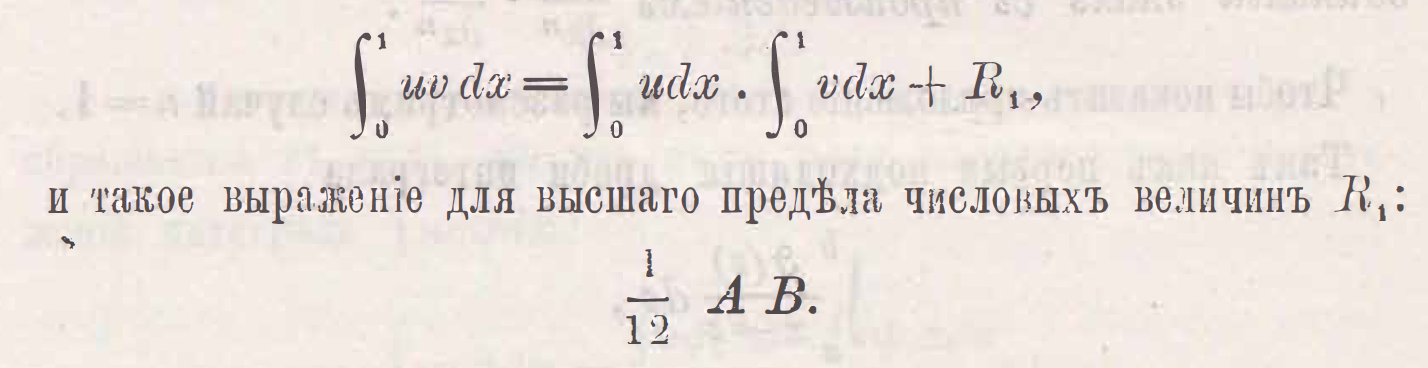
2$\begingroup$ This can’t hold with equality for general $u$, $v$. Likely the statement is $|R_1|\le AB/12$, or something of that sort. $\endgroup$ Commented Dec 13, 2020 at 13:42
-
5$\begingroup$ It reads as "the following expression is the maximum possible value for $R_1$: $\frac1{12}AB$". $\endgroup$ Commented Dec 13, 2020 at 14:36
-
2$\begingroup$ Chebyshev (whose name has a startling number of transliterations) has no fewer than THREE inequalities named after him. The one here, the one used in statistics, and the ones that gave the limsup and liminf result before the prime number theorem had been proved. $\endgroup$– AndreyFCommented Dec 13, 2020 at 14:50