This question is related to this one: Connectedness of the set having a fixed distance from a closed set. Suppose $F$ is a closed and connected set in $\mathbb{R}^n$ ($n>1$). Suppose the complement of $F$ is connected and let $$A=\{x\in \mathbb{R}^n: dist(x, F)=\delta\}, $$ where $\delta>0$ is fixed, and $dist$ is the Euclidean distance. If $F$ is unbounded with empty interior, can the complement of $A$ still have a bounded component?
$\begingroup$
$\endgroup$
3
-
1$\begingroup$ How about $n=3$, $F$ consists of the $x$-axis from $-\infty$ to $-2$ followed by a 0.1-dense continuous curve on the sphere of radius 2 about the origin followed by a line from 2 to $\infty$; and $\delta=1$. There is a component of $A$ inside the sphere (close to the unit sphere) and there is a component outside the sphere. $\endgroup$– Anthony QuasCommented Dec 11, 2020 at 7:05
-
3$\begingroup$ Or in $n=2$, an almost-complete arc of a circle, together with some ray heading to infinity to make it unbounded. $\endgroup$– Nate EldredgeCommented Dec 11, 2020 at 7:28
-
$\begingroup$ The complement of $F$ must be connected. Is this the case here? $\endgroup$– M. RahmatCommented Dec 11, 2020 at 18:00
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
6
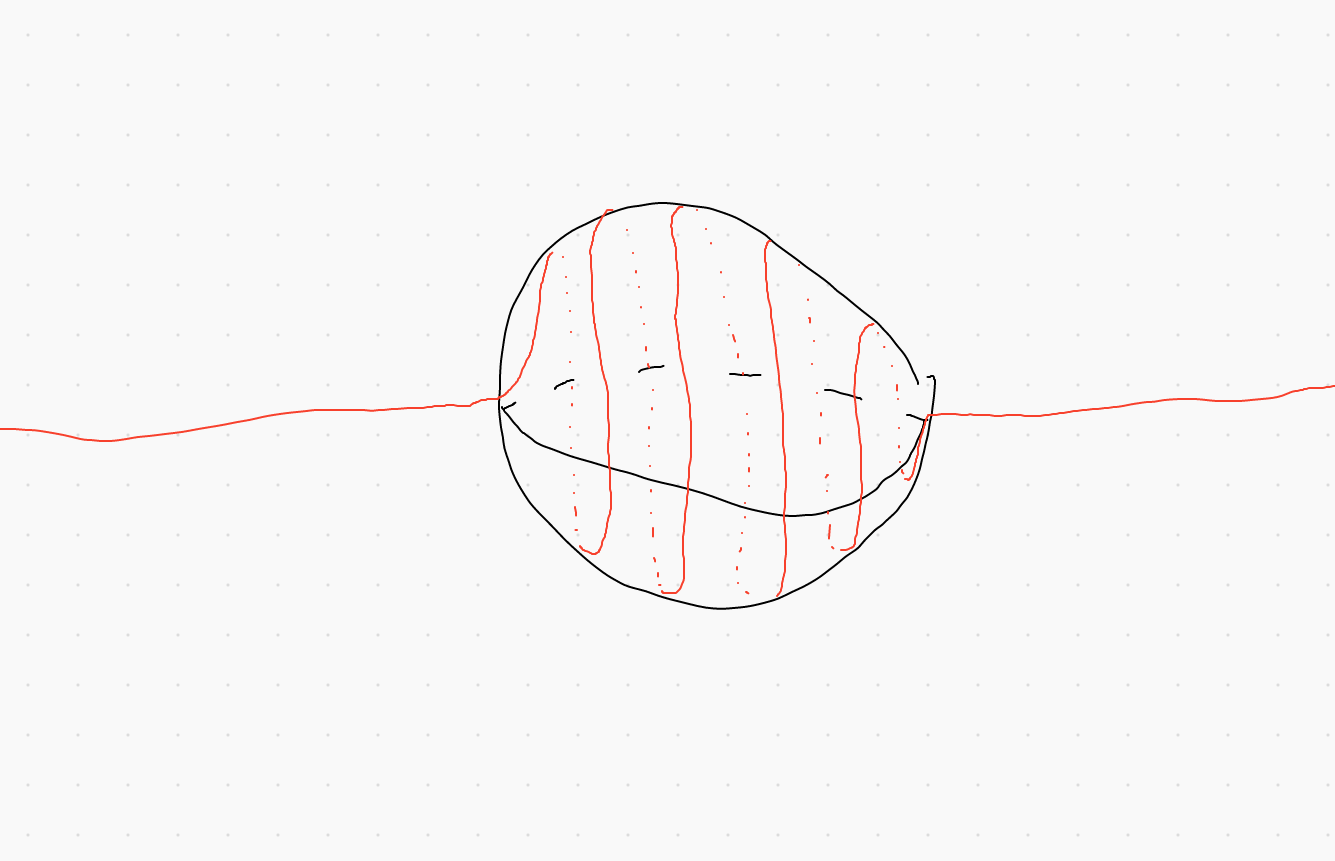
Here is a picture of the set $F$ (in red) in my comment above (the black lines represent the sphere of radius 2). There is a component of $A$ inside the sphere and a component outside the sphere.
-
$\begingroup$ The complement of $F$ must be connected. It seems to me that in your counter example this not the case. Could you please clarify? $\endgroup$ Commented Dec 11, 2020 at 18:36
-
$\begingroup$ @M.Rahmat $F$ is the red line, and its complement is connected $\endgroup$ Commented Dec 11, 2020 at 20:19
-
$\begingroup$ @ Anthony Quas I see. Thanks. Your construction is very good, but could you please tell me what you mean by 0.1-dense continuous curve? I have also another question (it was not in the question that I asked but to better understand): Is the complement of $A $ in your construction still connected, or disconnected because of the component of $A$ that is inside the ball? Thanks for your explanation. $\endgroup$ Commented Dec 11, 2020 at 21:44
-
1$\begingroup$ 0.1-dense in the sphere means that for each point in the sphere, there is a point on the curve at most 0.1 away. I think the complement of $A$ has three components: two components where $d(x,F)>1$: one of these is approximately the unit ball; and the other one an infinite component consisting of everything outside (in the everyday sense) of a fattened version of $F$. The third component is things where the distance to $F$ is strictly between 0 and 1. $\endgroup$ Commented Dec 11, 2020 at 21:50
-
$\begingroup$ @ Anthony Quas. It seems to me that the component of $A$ that is inside the sphere of radius 2 is not a complete sphere, but a smaller copy of $F$ on the sphere of radius 2; i.e. it is a 0.1-dense continuous curve around the sphere of radius 1. Could you tell me what you think? $\endgroup$ Commented Dec 12, 2020 at 18:31