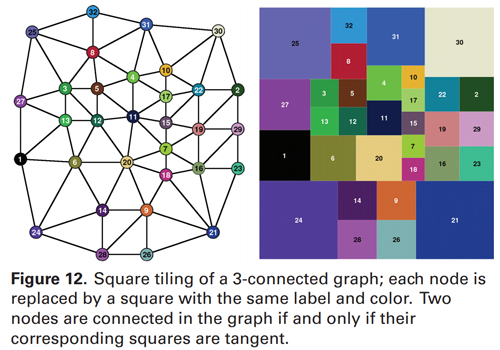
Is this a theorem?
Every $3$-connected planar graph $G$ may be represented as a tiling of a square by squares, one square per node of $G$, with nodes connected in $G$ corresponding to tangent squares.
I ask because the cited AMS Notices paper1 suggests that this might be a theorem, offering it as an example of "discrete Ricci flow" and a "generalization of circle packing." But no explicit reference is provided.
There are so many unresolved questions concerning square dissections that it would be interesting to see if this square packing result (if indeed it holds in some form) could address some of the unknowns.
1Gu, Xianfeng, Feng Luo, and Shing Tung Yau. "Computational conformal geometry behind modern technologies." Notices of the American Mathematical Society 67, no. 10 (2020): 1509-1525.