3
$\begingroup$

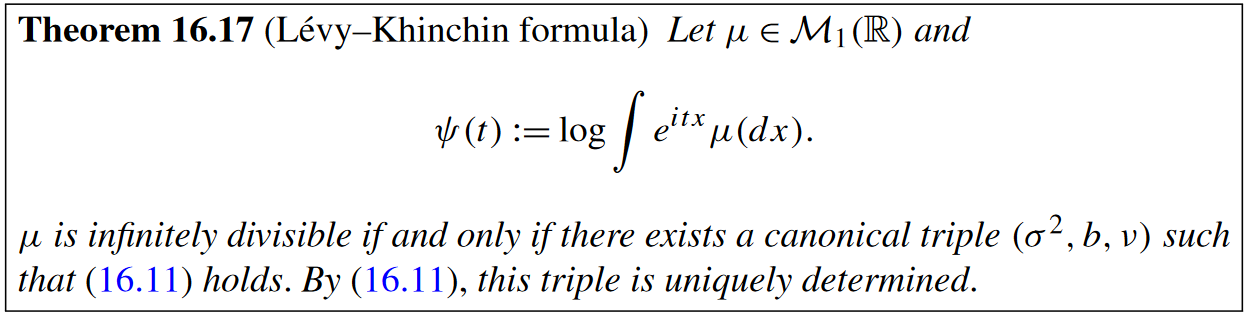
Please take a look at the following statement of the Lévy–Khintchine formula given in Probability Theory: A Comprehensive Course (2nd edition)$^1$:

enter image description here

Am I missing something or is this an ill-posed statement? What I mean is the following: If $\mu$ is infinitely divisible, we can show that the characteristic function $\varphi_\mu$ of $\mu$ is $\ne0$ on its entire domain, which in turn implies that there is a unique $f\in C^0(\mathbb R,\mathbb C)$ with $f(0)=0$ and $$\varphi_\mu=e^f.\tag1$$ I'm still trying to understand the purpose of the Lévy–Khintchine formula, but if I got it right, the goal is to give a characterization of $f$.

Now, I guess some authors (including the one of the cited book) write $\ln\varphi_\mu:=f$; which is confusing, since $f$ is clearly not the composition of $\ln$ and $\varphi_\mu$.

However, even with this possible notational hack in mind, if we are not a priori assuming that $\mu$ is infinitely divisible, the existence of $f$ cannot be shown and hence $\psi$ is (at best) a multivalued function.

Am I missing something? Every source I was able to find states the Lévy–Khintchine formula in this way. Wouldn't it be better to state the claim for $\int e^{{\rm i}tx}\mu({\rm d}x)=\varphi_\mu(t)$ instead?

$^1$ enter image description here enter image description here

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ This assumes "good will" of the reader, I believe. Compare with the following statement: If $\sqrt{x} = 1$, then $x = 1$. This is usually assumed to be true, but you could argue that if $x < 0$, then what is meant by $\sqrt{x}$? Here, in a similar way, you should read the definition of $\psi$ as "Suppose that the characteristic function of $\mu$ is non-zero, and its continuous logarithm is denoted by $\psi$." $\endgroup$
    – Mateusz Kwaśnicki
    Commented Nov 15, 2020 at 21:48
  • $\begingroup$ Hi, I am wondering why $\phi_\mu\neq 0$ implies that there exiets a $f$ such that $\phi_\mu=e^f$? $\endgroup$
    – Mingzhou Liu
    Commented Sep 21 at 2:20

1 Answer 1

Reset to default
1
$\begingroup$

Apologies if this is an inappropriate place to post a comment (I am a newbie). But I wanted to mention that an easier to read treatment of infinite divisibility is Chapter 3 of the classic book by Gnedenko and Kolmogorov. Other possibilities (but I cannot access them just now in order to be sure) are the books by Lukacs on Characteristic Functions, and possibly Sato's book on Levy Processes and Infinitely Divisible Distributions. As an aside, my preferred way to think of infinitely divisible distributions is that they coincide with the limiting distributions of weighted sums of independent Poisson distributed random variables.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .