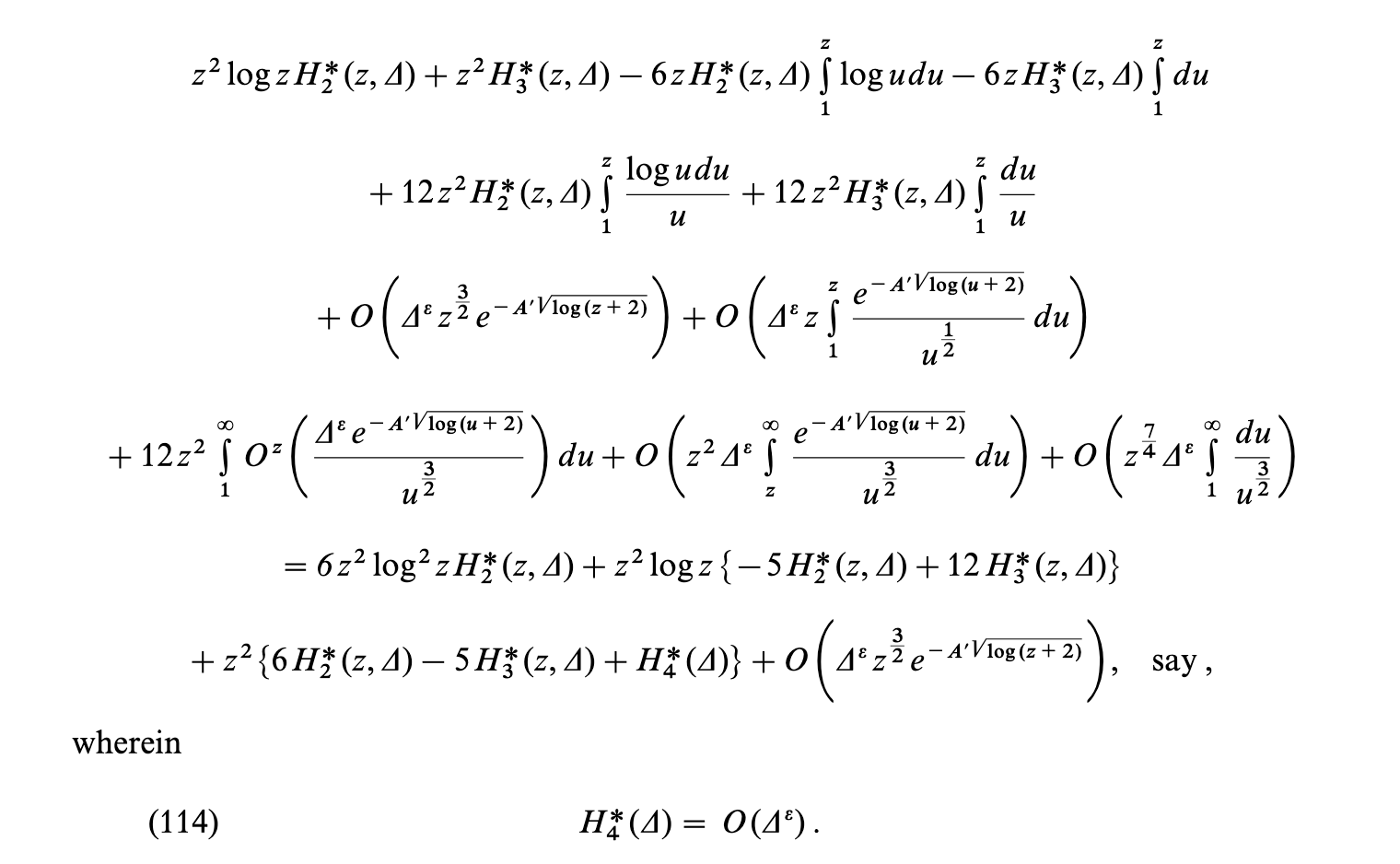My question is just a ``I don't understand what goes on in X of paper Y" so I don't know if I can post it; on the other hand it is research. I posted it in stackexchange but it received no answers, so does that mean I'm allowed to post it here? I'm never sure.
Anyway, my question is on Christopher Hooley's paper on the third moment for primes in arithmetic progressions (``On the Barban-Davenport-Halberstam Theorem VIII"):
For variables $z,z_1,\Delta $ he defines two quantities, $\mathcal J_1(z,\Delta )$ and $\mathcal J_2(z_1,\Delta )$, in terms of quantities $\Gamma _\Delta (\cdot )$. As far as my question is concerned, I don't think we don't need to know anything about these $\Gamma _\Delta (\cdot )$. The quantities $\mathcal J_1(z,\Delta )$ and $\mathcal J_2(z_1,\Delta )$ are as follows. From (64) we have \[ \mathcal J_1(z,\Delta )=\sum _{l<z}\frac {(z-l)^2}{l}\Gamma _\Delta (l) \sum _{l_1+l_2=l}\Gamma _\Delta (l_1)\Gamma _\Delta (l_2)\] and from (68) we have \[ \mathcal J_2(z_1,\Delta )=\sum _{l<z_1}(z_1-l)^2l\Gamma _\Delta (l) \sum _{l_1+l_2=l}\Gamma _\Delta (l_1)\Gamma _\Delta (l_2).\] From (112) we have \[ (1)\hspace {10mm}\mathcal J_2(z_1,\Delta )=\text { main term } +\mathcal O\left (\frac {\Delta ^\epsilon z_1^{7/2}}{z^{1/4}}\right ) +\mathcal O^z\left (\Delta ^\epsilon z_1^{7/2}e^{-\sqrt {\log (z_1+2)}}\right )\] for any $z$ with $z\geq z_1$, according to the top of page 38. Here the symbol $\mathcal O^z(f)$ denotes a quantity that is $\mathcal O(f)$ and independent of $z$, also said at the top of page 38.
(This introduction of a new variable is described through the expression \[ \mathcal J_2(z_1,\Delta )=\sum _{d<z\atop {(d,2\Delta )=1}}\frac {\mu ^2(d)}{\theta _2(d)} \sum _{l<z_1\atop {l\equiv 0\text { mod }d}}(z_1-l)^2l\sum _{l_1+l_2=l}\Gamma _\Delta (l_1)\Gamma _\Delta (l_2)\] which is the equality immediately after (68), and through the bottom of page 37, which says we can change the $d$ summation range from $d<z_1$ to $d<z$ for any $z\geq z_1$, since the condition $d<z_1$ is automatic from the $l\equiv 0\text { mod }d$ condition in the $l$ sum.)
From Lemma 3 (page 25) $\mathcal J_1(z,\Delta )$ and $\mathcal J_2(z_1,\Delta )$ are related through \[ (2)\hspace {10mm}\mathcal J_1(z,\Delta )=\frac {\mathcal J_2(z,\Delta )}{z^2}-6z\int _{0}^z\frac {\mathcal J_2(z_1,\Delta )dz_1}{z_1^4} +12z^2\int _{0}^z\frac {\mathcal J_2(z_1,\Delta )dz_1}{z_1^5}.\] At the bottom of page 38 and the first half of page 39 he says he inserts $(1)$ into $(2)$ to calculate $\mathcal J_1(z,\Delta )$ up to an error \[ \mathcal O\left (\Delta ^\epsilon z^{3/2}e^{-A'\sqrt {\log (z+2)}}\right ) +\mathcal O\left (\Delta ^\epsilon z\int _1^z\frac {e^{-A'\sqrt {\log (u+2)}}}{u^{1/2}}du\right )\] \[ 12z^2\int _1^\infty \mathcal O^z\left (\frac {\Delta ^\epsilon e^{-A'\sqrt {\log (u+2)}}}{u^{3/2}}\right )du +\mathcal O\left (z^2\Delta ^\epsilon \int _z^\infty \frac {e^{-A'\sqrt {\log (u+2)}}}{u^{3/2}}du\right )+O\left (z^{7/4}\Delta ^\epsilon \int _1^\infty \frac {du}{u^{3/2}}\right ).\] He then says (in the same equation passage) the total error above is \[ z^{3/2}\Delta ^\epsilon e^{-A'\sqrt {\log (z+2)}}\] which to me doesn't seem to be right in view of the $12z^2$ term.
My first question is simple - is this a mistake/slip?
If not, how does it follow? If it is a mistake, what could he have meant? I've tried to work out what he could have meant, but as far as I can see, even if $(1)$ read more strongly \[ \mathcal J_2(z_1,\Delta )=\text { main term }+\mathcal O\left (1\right )\] and we inserted this into $(2)$ we couldn't avoid an error term of the form \[ 12z^2\int _{0}^z\frac {dz_1}{z_1^5}\] so that we can't calculate $\mathcal J_1(z,\Delta )$ up to an error better than $z^2$.
But this would mean the whole result is wrong, since the main term is around this size, so my interpretation/understanding is clearly nonsense. So my second question is: can anyone clear this up from me?