3
$\begingroup$

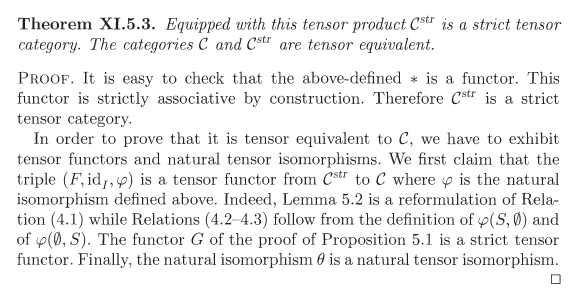
I'm reading the book "Quantum groups" by Kassel. I'm reading the proof that every tensor category is tensor equivalent with a strict tensor category. Here is the proof given. Below is more context.

enter image description here

The functor $G: \mathcal{C}\to \mathcal{C}^{str}$ is given by $G(V) =(V)$. However, I believe $G$ is NOT strict since $$G(U \otimes V) = (U \otimes V) \neq (U,V) = (U)*(V) = G(U)*G(V)$$

Is there a way to fix this proof? Maybe $G$ is not a strict tensor functor but still a tensor functor that gets the job done?

If not, can someone give a reference where this "finite sequence" approach to constructing the strict tensor category works?

Here is more context:

Let $\mathcal{S}$ be the class of finite sequences $(V_1, \dots, V_k)$ of objects in $\mathcal{C}$. We include the empty sequence $\emptyset$. The integer $k$ is by definition the length of the sequence. If $S= (V_1, \dots, V_k)$ and $S' = (W_1, \dots, W_l)$ are non-empty finite sequences, then we define $S*S' := (V_1, \dots, V_k, W_1, \dots, W_l)$. We also define $S*\emptyset = S = \emptyset*S$ for every finite sequence $S \in \mathcal{S}$.

We now associate to every $S \in \mathcal{S}$ an object $F(S) \in \mathcal{C}$. We give an inductive definition (recursion on the length of the finite sequence).

  • $F(\emptyset) = I$

  • $F((V)) = V$, $\quad V \in \mathcal{Ob} \mathcal{C}$

  • $F(S*(V)) = F(S) \otimes V$, $\quad S \in \mathcal{S}\setminus \{\emptyset\}, V \in \mathcal{Ob} \mathcal{C}$

More generally, if $(V_1, \dots, V_k) \in \mathcal{S}$, we get $$F((V_1, \dots, V_k)) = (\dots((V_1 \otimes V_2) \otimes V_3) \otimes \dots )\otimes V_{k-1})\otimes V_k$$

We can now define the category $\mathcal{C}^{str}$. This is the category determined by:

  • $\mathcal{Ob} \mathcal{C}^{str}= \mathcal{S}$

  • $Hom_{\mathcal{C}^{str}}(S,S') = Hom_\mathcal{C}(F(S), F(S')), \quad S,S' \in \mathcal{S}$

  • Composition of morphisms in $\mathcal{C}^{str}$ is simply the composition of the corresponding morphisms in $\mathcal{C}$.

__

The functor $G: \mathcal{C} \to \mathcal{C}^{str}$ is defined by $G(V) = (V)$ and $G(f) = f$.

Improve this question
$\endgroup$
6
  • 8
    $\begingroup$ I assume from what's said that the objects of $C^{\mathrm{str}}$ are finite sequences of objects of $C$ and that the functor $F$ multiplies a sequence of objects together using the tensor product of $C$. In this case I think the answer is just that $G$ is a strong monoidal functor. $\endgroup$
    – Mike Shulman
    Commented Jun 19, 2020 at 13:09
  • $\begingroup$ @MikeShulman In the book, strict tensor functor $F$ is defined as a tensor functor $(F, \varphi_0, \varphi_2)$ where $\varphi_0$ and $\varphi_2$ are identities, which is not the case here? What transformations should I use then instead of identities? $\endgroup$
    – user159891
    Commented Jun 19, 2020 at 13:17
  • $\begingroup$ Strong means the natural transformations are natural isomorphisms, that is strong is named because it's stronger than "lax" or "oplax." Strong does not mean strict! Strong is weaker than strict. I think Kassel just calls strong tensor functors "tensor functors." $\endgroup$
    – Noah Snyder
    Commented Jun 19, 2020 at 18:14
  • $\begingroup$ @NoahSnyder And do you see how to prove that $G$ is a tensor functor then? What transformations should I use? $\endgroup$
    – user159891
    Commented Jun 19, 2020 at 18:24
  • $\begingroup$ I agree that the monoidal functor $G$ is not strict. For the proof, it doesn't need to be strict. We only need a tensor equivalence, i.e. an equivalence of categories involving monoidal functors (in Kassel's terminology). The way Kassel defines a monoidal functor, it is what others (as Mike Shulman remarked), call a strong monoidal functor, i.e. the structural natural transformation $(G_2)_{U,V}\colon G(U)\otimes G(V)\to G(V\star U)$ consists of isomorphisms. $\endgroup$
    – Zahlendreher
    Commented Jun 19, 2020 at 18:56

1 Answer 1

Reset to default
3
$\begingroup$

Our goal is to find maps in $$\mathrm{Hom}_{\mathscr{C}^{str}}((U\otimes V), (U,V)) = \mathrm{Hom}_{\mathscr{C}}(U\otimes V, U\otimes V)$$ satisfying some identities. But there's a very natural element on the RHS which is $\mathrm{id}_{U \otimes V}$. Ah, and now we see why Kassel got confused, which is that these maps are written as identity maps, but they're not literally identity maps in $\mathscr{C}^{str}$ and so you don't literally have a strict functor.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Thanks! I will see if this works! $\endgroup$
    – user159891
    Commented Jun 19, 2020 at 18:43
  • $\begingroup$ So if I understand correctly, we have that $(G, \varphi_0 = id_I, \varphi_2)$ is a tensor functor where $id_I \in Hom_{C^{str}}(\emptyset, (I))$ and $\varphi_2(U,V):= id_{U \otimes V} \in Hom_{C^{str}}((U,V),(U \otimes V))$? $\endgroup$
    – user159891
    Commented Jun 19, 2020 at 19:30
  • $\begingroup$ @user745578: Yes, that's my proposal. $\endgroup$
    – Noah Snyder
    Commented Jun 19, 2020 at 19:35
  • $\begingroup$ It seems very tedious to verify that this indeed defines a tensor functor. I checked one of the three diagrams and it worked out, so I'm getting confident that it works! Many thanks for the help! $\endgroup$
    – user159891
    Commented Jun 19, 2020 at 19:59

You must log in to answer this question.