Who first characterized the real numbers as the unique complete ordered field?

Question

Nearly every mathematician nowadays is familiar with the fact that there is up to isomorphism only one complete ordered field, the real numbers.

Theorem. Any two complete ordered fields are isomorphic.

Proof. $\newcommand\Q{\mathbb{Q}}\newcommand\R{\mathbb{R}}$Let us observe first that every complete ordered field $R$ is Archimedean, which means that there is no number in $R$ that is larger than every finite sum $1+1+\cdots+1$. If there were such a number, then by completeness, there would have to be a least such upper bound $b$ to these sums; but $b-1$ would also be an upper bound, which is a contradiction. So every complete ordered field is Archimedean.

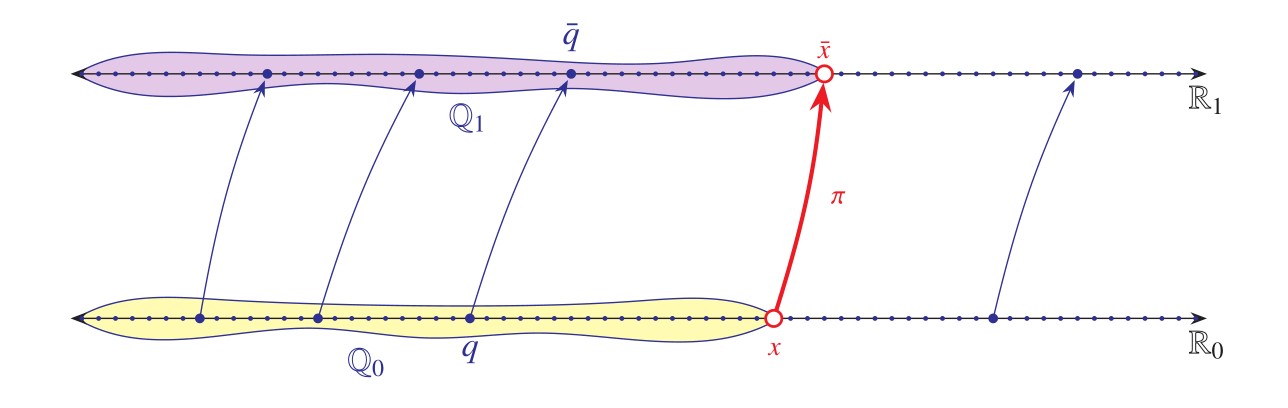
Suppose now that we have two complete ordered fields, $\R_0$ and $\R_1$. We form their respective prime subfields, that is, their copies of the rational numbers $\Q_0$ and $\Q_1$, by computing inside them all the finite quotients $\pm(1+1+\cdots+1)/(1+\cdots+1)$. This fractional representation itself provides an isomorphism of $\Q_0$ with $\Q_1$, indicated below with blue dots and arrows:

Next, by the Archimedean property, every number $x\in\R_0$ is determined by the cut it makes in $\Q_0$, indicated in yellow, and since $\R_1$ is complete, there is a counterpart $\bar x\in\R_1$ filling the corresponding cut in $\Q_1$, indicated in violet. Thus, we have defined a map $\pi:x\mapsto\bar x$ from $\R_0$ to $\R_1$. This map is surjective, since every $y\in\R_1$ determines a cut in $\Q_1$, and by the completeness of $\R_0$, there is an $x\in\R_0$ filling the corresponding cut. Finally, the map $\pi$ is a field isomorphism since it is the continuous extension to $\R_0$ of the isomorphism of $\Q_0$ with $\Q_1$. $\Box$

My expectation is that this theorem is familiar to almost every contemporary mathematician, and I furthermore find this theorem central to contemporary mathematical views on the philosophy of structuralism in mathematics. The view is that we are entitled to refer to the real numbers because we have a categorical characterization of them in the theorem. We needn't point to some canonical structure, like a canonical meter-bar held in some special case deep in Paris, but rather, we can describe the features that make the real numbers what they are: they are a complete ordered field.

Question. Who first proved or even stated this theorem?

It seems that Hilbert would be a natural candidate, and I would welcome evidence in favor of that. It seems however that Hilbert provided axioms for the real field that it was an Archimedean complete ordered field, which is strangely redundant, and it isn't clear to me whether he actually had the categoricity result.

Did Dedekind know it? Or someone else? Please provide evidence; it would be very welcome.

Answer 1

Joel, I believe this was first explicitly stated and proved by E.V. Huntington in his classic paper: Complete sets of postulates for the theory of real quantities, Trans. Am. Math. Soc. vol. 4, No. 3 (1903), pp. 358-370. See Theorem II', p. 368.

Edit (June 14, 2020): It is perhaps worth adding that in 1904, the year following the publication of Huntington's paper, O. Veblen published his paper A System of Axioms for Geometry, Trans. Am. Math. Soc. vol. 5, no. 3, pp. 343-384, in which he introduced the idea of a categorical system of axioms. He illustrated his conception with Huntington's above mentioned characterization of the reals (pp. 347-348). No doubt, this is mentioned in the paper referred to below by Ali Enayat.

EDIT (8/27/23)

In light of the doubts raised by Zvonimir Sikic regarding Huntington being the originator of the idea of a categorical system of axioms, it should be noted that while Huntington was well aware that there existed categorical axiom sets in the literature prior to his isolation of the concept, including those axiom sets mentioned by Sikic, he held the view that the earlier writers neither isolated the idea nor expressed any interest in it. He made this point explicitly as follows in 1913 when he provided a novel categorical characterization of Euclidean geometry.

More important than the question of independence is the proof of the sufficiency of the postulates to determine a unique type of system; or, to use a phrase of Veblen’s, the proof that the postulates form a categorical set. Little attention seems to have been paid to this question except by the present writer in connection with the foundations of analysis, and by Veblen in connection with the foundations of geometry; and yet there appears to be no other way of proving that all the propositions of a science are deducible from a given set of postulates, than by showing that the postulates form a ‘sufficient' or ‘categorical' set.” (pp. 524-525)

—Huntington, E. V. 1913. “A Set of Postulates for Abstract Geometry, Expressed in Terms of the Simple Relation of Inclusion.” Mathematische Annalen 73 (4):522–59. (pdf from archive.org)

For what it's worth, David Hilbert was the managing editor of Mathematische Annalen at the time of the publication of Huntington's paper.

Edit: 8/28/23

I should have mentioned in my previous edit, that contrary to Zvonimir Sikic contention, Huntington's paper of 1903 does indeed contain an axiomatization of the ordered field of real numbers as well as a proof of it categorical nature. In Section 2 he provides an axiomatization of the ordered field of real numbers (consisting of 14 axioms including the Dedekind Completeness axiom) and in Theorem II' on page 368 he proves that the axiomatization is categorical.

Answer 2

The key question here is that Dedekind complete implies Archimedean, as in the first paragraph of Joel's Proof.

This was shown by Otto Stolz, in Zur Geometrie der Alten, insbesondere über ein Axiom des Archimedes, Mathematische Annalen, 22(4):504–519, 1883.

Apparently Stolz was persuaded to retract this paper.

I confess that I can't read German with any fluency and only got through the first part of the paper, but it does seem to me that that contains the correct argument that Joel states. Therefore Stolz deserves the credit.

The later part of the paper looks rather strange, but that's in part because I didn't follow the text.

However, Mikhail knows more about the history and will no doubt explain.

Footnote: I conjecture that a version of Conway's "surreal" numbers using computably enumerable subsets instead of general ones might be able to avoid Stolz's argument and yield a Dedekind complete but non-Archimedean field.

Some years ago, I put this conjecture to (the late) John Conway, he scratched his head and said "maybe".

Answer 3

Hilbert's „Über den Zahlbegriff” published in 1900. is the first modern axiomatization of Archimedean fields. To axiomatize R, Hilbert added the maximality axiom (i.e. R is a maximal Archimedean field). He asserted that the existence of the model of the axioms is „nur einer geeigneten Modification bekkanter Schlussmethoden” i.e. „is just a suitable modification of known methods of reasoning”, and uniqueness trivially follows from maximality.

I guess that „bekkanter Schlussmethoden” were the following: If S is an ordered field it contains an isomorphic copy of Q, so let’s call it Q. Every point in S determines a cut in Q. If S is also Archimedean then every cut in Q determines none or one point in S. If S is also maximal then every cut in Q must determine one point in S. Namely, if it doesn’t it could be extended to an Archimedean field with this property, which is by Dedekind construction isomorphic to R. Hence, there is, up to isomorphism, exactly one maximal Archimedean ordered field which is isomorphic to R and every other is, up to isomorphism, contained in R.

This kind of argument has been well known since the time of Dedekind's „Stetigkeit und irrationale Zahlen", i.e. for more than 30 years. Everyone interested in the topic knew the argument, so Hilbert did not consider it necessary to repeat it. This is why I believe that Hilbert proved that maximality uniquely determines R just by stating that the proof is "a suitable modification of known methods of reasoning" (even though he didn't actually formulate the result in terms of isomorphism and didn't give the proof himself).

Hölder published a more general results in „Die Axiome der Quantität und die Lehre vom Mass” from 1901. He proved, in modern terms, that every continous system of magnitudes is isomorphic to R (it is a trivial corollary that every complete ordered field is isomorphic to R). A continous system of magnitudes was axiomatized with trichotomy, associativity, density, positivity (a < a + b), difference (if a < b then there is c such that a+c= b), and Dedekind's axiom (there is no gaps). In his terminology „there is a [real] measure-number for each given magnitude and there is a magnitude for each given [real] measure-number“ and the correspondence preserves the defining properties. It follows taht „if one has two systems of magnitudes, both fulfilling axioms I to VII, then the systems can be explicitly related to each other such that the sums [and order] of corresponding magnitudes also correspond“.

Huntington, in his „A complete set of postulates for the theory of absolute continuous magnitude“ from 1902, wrote: “The set of postulates adopted in the present paper is nearly the same as the second set of Hölder’s, with the exception that Dedekind's postulate of continuity is here replaced by Weierstrass's”. So, Huntington is not concerned with Archimedean fields but with Hölder's systems of magnitudes. He proved in „Complete Sets of Postulates for the Theory of Real Quantities“ from 1903. that „any two assemblages, M (<, +) and M ' (<, +), which satisfy the [Hölder's] postulates 1-10 are equivalent; that is, they can be brought into one-to-one correspondence in such a way that when a and b in M correspond to a' and b' in M', we shall have, a' < b' whenever a < b and a + b will correspond to a' + b' “.

So, Huntington proved the categoricity of (R, <, +) in Section 1 of his 1903 paper, as Hölder did in #15 of his 1901 paper. As Philip Ehrlich pointed out, Huntington also proved the categoricity of (R, <, +, x) in section 2 of his 1903 paper, which Hölder apparently did not. So it may seem that Huntington did something more after all. However, in #16 Hölder defined multiplication in (R,<,+) and proved in #17 that it is unique, so there was no need for special treatment of (R, <, +, x).

Hence, as far as categoricity is concerned, there is nothing in Huntington's work that we do not already find in Hölder's. Therefore, it is not surprising that Ebbinghaus et. al. in references to the chapter on real numbers and their axiomatization (in their book "Zahlen") do not mention Huntington, only Hilbert and Hölder.

Answer 4

I am confused by the failure to recognize the set-theoretic issue. Without requiring Archimedeanity, Conway’s Surreals are ordered, and satisfy the field axioms, and have no gaps! The only reason they can’t be a complete ordered field, if you use a no-gap definition of “completeness” rather than one involving sequences or the Archimedean property or a least upper bound property, is that they are too big to be a set.

You need to give a simple answer to “why aren’t the surreal numbers a second complete ordered field?”