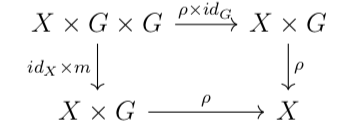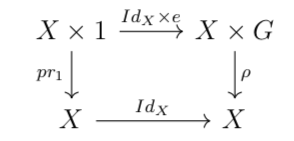It is well known that a group object in a category $C$ (with terminal object $1$ and such that any two objects of $C$ have a product) is defined as an object $G$ in $C$ with the following morphisms:
$m:G \times G \rightarrow G$, $e:1 \rightarrow G$, $\mathit{inv}: G \rightarrow G$
satisfying some conditions modelled on the group axioms such that $m$ behaves as the multiplication map, e behaves as the identity and $\mathit{inv}$ behaves as the inverse map.
The group action (right) of a group object $G$ on an object $X$ in $C$ can be defined as a morphism $\rho:X \times G \rightarrow X$ in $C$ such that the following two diagrams are commutative:
where $\mathit{id}_X$, $\mathit{id}_G$ are identity morphisms at $X$ and $G$ respectively and $\mathit{pr}_1$ is the first projection on $X$ from the product $X \times 1$.
My Question are the following:
(1) What is the analogue of the above notion in a $2$-category? I couldn't find any literature in this direction.
So I tried to guess it's definition roughly in the following way:
Let $C$ be a 2-category (with terminal object $1$ and such that any two objects of $C$ have a product in the context of 2- category as mentioned in https://ncatlab.org/nlab/show/2-limit#2limits_over_diagrams_of_special_shape ). I define a group object in the 2-category $C$ as an object $G$ with the following 1-morphisms:
$m \in C(G \times G ,G)$, $e \in C(1,G)$, $inv \in C(G,G)$ satisfying some conditions similar as above but in this case every equality in the conditions will be replaced by an invertible 2-morphism satisfying certain appropriate coherent conditions.
Correspondingly the action of $G$ on an object $X$ in $C$ will be defined exactly in the same way but the above two diagrams will be commutative only upto invertible 2-morphisms.
Is my guess correct?
Even if it is correct but writing all the details (taking care of all the invertible 2 morphisms) seems very complicated to me and seems an inappropriate definition to work with.(Both in the context of strict 2-category and bicategory)
So what should be an appropriate definition for a group object in a 2-category and its corresponding action on an object? (Both in the context of strict 2-category and bicategory)
Secondly,
It is well known that the Strict 2-Group is a group object in Cat(when Cat is considered as a 1 -category or a usual category ).
But then
(2) What is the group object in Cat (when Cat is considered as strict 2-category)?
I would be also very grateful if someone can refer some literatures in this direction.
Thank you.