Trying to bound the generalized hypergeometric function ${}_2F_3(x+1,x+1;1,1,1;\alpha)$ as $x\to \infty$?

Question

(See also edit below)...

I am trying to get a nice, explicit, bound on the hypergeometric function $$ {}_2F_3(a_1,a_2;b_1,b_2,b_3;\alpha), $$ in the case of a large parameter. In particular I am interested in the case where $$ {}_2F_3(x+1,x+1;1,1,1;\alpha), \quad \quad x \to \infty. $$

I found this paper that shows how we can decompose hypergeometric functions into sums of hypergeometric functions of lower orders, when they have integer parameters differences.

Using the method in the paper I decomposed the above function as \begin{align*} {}_2F_3(x+1,x+1;1,1,1;\alpha) & = \sum_{j=0}^x {x\choose j} {}_1F_2(x+1+j;1+j,1+j;\alpha) \frac{(x+j)!}{x!(j!)!} \alpha^j \\ \end{align*}

I then bound the binomial coefficient with ${x\choose j}\le (ex)^j(1/j)^j$, and applied Sterling's approximation $(1/j)^j \le 1/(j!e^j)$ to get: \begin{align*} {}_2F_3(x+1,x+1;1,1,1;\alpha) & \le \sum_{j=0}^x x^j {}_1F_2(x+1+j;1+j,1+j;\alpha) \frac{(x+j)!}{x!(j!)^4} \alpha^j \\ \end{align*}

However, while the hypergeometric function has now been reduced, I don't seem to be any closer to being able to get a nice explicit expression.

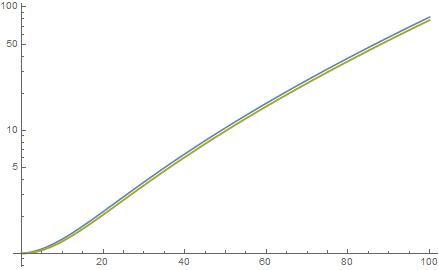
Somewhat surprisingly, upon running some numerics, simply neglecting the ${}_2F_1$ seems to still give an excellent approximation (yellow line in image):

\begin{align*} {}_2F_3(x+1,x+1;1,1,1;\alpha) & \lessapprox \sum_{j=0}^x x^j \frac{(x+j)!}{x!(j!)^4} \alpha^j \\ \end{align*}

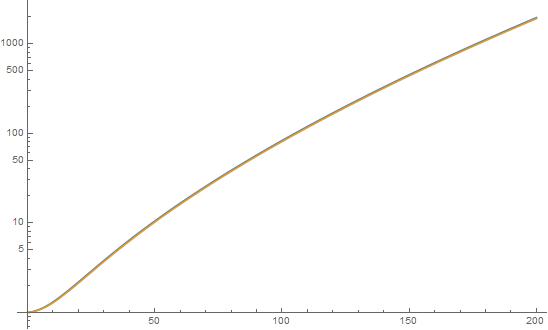
Here, $\alpha=1/4$ was used.

But even with that simplification, I don't seem to be much better off when it comes to letting $x\to \infty$ and finding an explicit bound; I am looking for bound that doesn't feature a series/integral/special function.

The function looks pretty straightforward on the plot so I would hope it is possible to get a nice bound for it. Apart from my attempt above, I have looked through a lot of literature for useful identities/techniques but haven't been able to find anything.

So is it possible to get a nice explicit bound on this function?

EDIT: Ok, it seems there is another simplification (numerically at least for the moment); it seems we can 'transfer' the $x$ and reduce (blue line in image) $$ {}_2F_3(x+1,x+1;1,1,1;\alpha) $$ to (green line in image): $$ {}_0F_3(;1,1,1;x^2\alpha) $$

So this suggests that it may be possible to get a nice bound on ${}_2F_3(x+1,x+1;1,1,1;\alpha)$ if we can find an asymptotic representation of ${}_0F_3(;1,1,1;x^2\alpha)$ for large x?

Note that ${}_0F_3(;1,1,1;x^2\alpha) = \sum_{j=0}^\infty (1/j!)^4 (x^2\alpha)^j$.

Answer 1

You have done much of the work for yourself. Here is the last missing step:

Writing the $_2 F_3$ as integral (see, e.g., http://dlmf.nist.gov/16.5.E1): $$ _2 F_3(x+1,x+1;1,1,1;\alpha)=\Gamma(x+1)^{-2}\ \frac{1}{2 \pi i}\int_{\cal{L}} d s \frac{\Gamma(x+1+s)^2}{\Gamma(s+1)^3} \ \Gamma(-s) \ (-\alpha)^s, $$ where the path $\cal{L}$ is described in the source given above. Then we insert the approximation for large $x$ (see, e.g., http://dlmf.nist.gov/5.11.E12) $$ \left(\frac{\Gamma(x+1+s)}{\Gamma(x+1)}\right)^2 \sim x^{2 s} . $$ Therefore for large $x$ $$ _2 F_3(x+1,x+1;1,1,1;\alpha)\sim \int_{\cal{L}} d s \ \Gamma(s+1)^{-3} \ \Gamma(-s) \ (-\alpha x^2 )^s = \ _0 F_3(;1,1,1;x^2 \ \alpha), $$ which gives you the desired asymptotic similarity.

Answer 2

I found large argument asymptotics in for ${}_0F_3$ in this book, specifically Formula 16.11.9.

Using these, I obtained \begin{align} {}_2F_3(x+1,x+1;1,1,1;\alpha) \approx {}_0F_3(;1,1,1;x^2\alpha) \sim Ce^{4(x^2 \alpha)^{1/4}}, \quad x\to \infty, \end{align} where $C > 0$. Numerics confirm it.

Now I just need to find out why ${}_2F_3(x+1,x+1;1,1,1;\alpha) \approx {}_0F_3(;1,1,1;x^2\alpha)$...