I suspect that the function
$$F(q) = \sum_{n \geq 0} (2n + 1) \, q^\binom{n+1}{2}$$
may be some kind of modular form. It looks like a weighted theta function, but is not exactly an harmonic theta series.
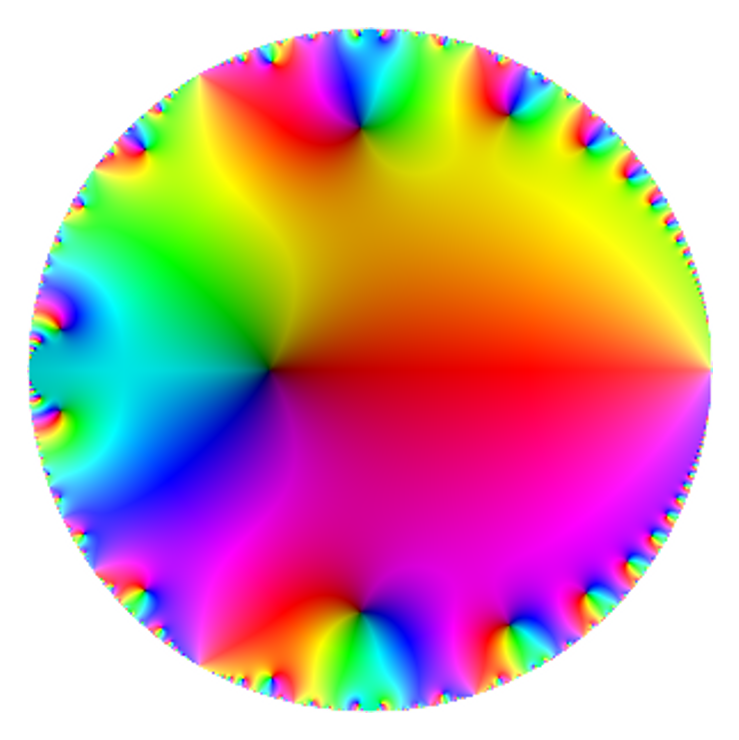
Plotting this function in the complex unit disc suggests a modular behaviour. There is a real zero around $-0.3$, and other zeros inside the disc.
The formula above has an hidden symmetry, because summation over all negative integers would give the opposite function.
Is $F(q)$ indeed a modular form ?
And if so
Does it appear in the literature ?
I am also interested in the expansion around $q=1$, where there is a pole. But this would be another question.
EDIT:
The answer below by Somos is interesting, but it only tells us that another resembling series (with alternating signs) is a modular form. It is not possible to pass from this modular form to $F(q)$ by just changing $q$ to $-q$.
EDIT:
Trying to follow the suggested track of Eichler integrals for modular forms of half-integer weights and quantum modular forms, one auxiliary question is now whether the following is a modular form:
$$\sum_{n \geq 0} q^{(2n+1)^2/8}.$$
Here the sum can also be taken over $\mathbb{Z}$, so this is rather similar to a theta function...
EDIT: ... and in fact is equal to the difference
$$\sum_{n} q^{n^2/8} - \sum_{n} q^{n^2/2}.$$
EDIT: Here is a picture