What is the order of the left tail of a mixture of non-central chi-square?

Question

Let $\mu\sim N(0,1)$, $Z\sim N(\mu,1)$. Then $Z$ can be viewed as a mixture of Gaussians. It can also be viewed as a Gaussian but there is a prior for the mean.

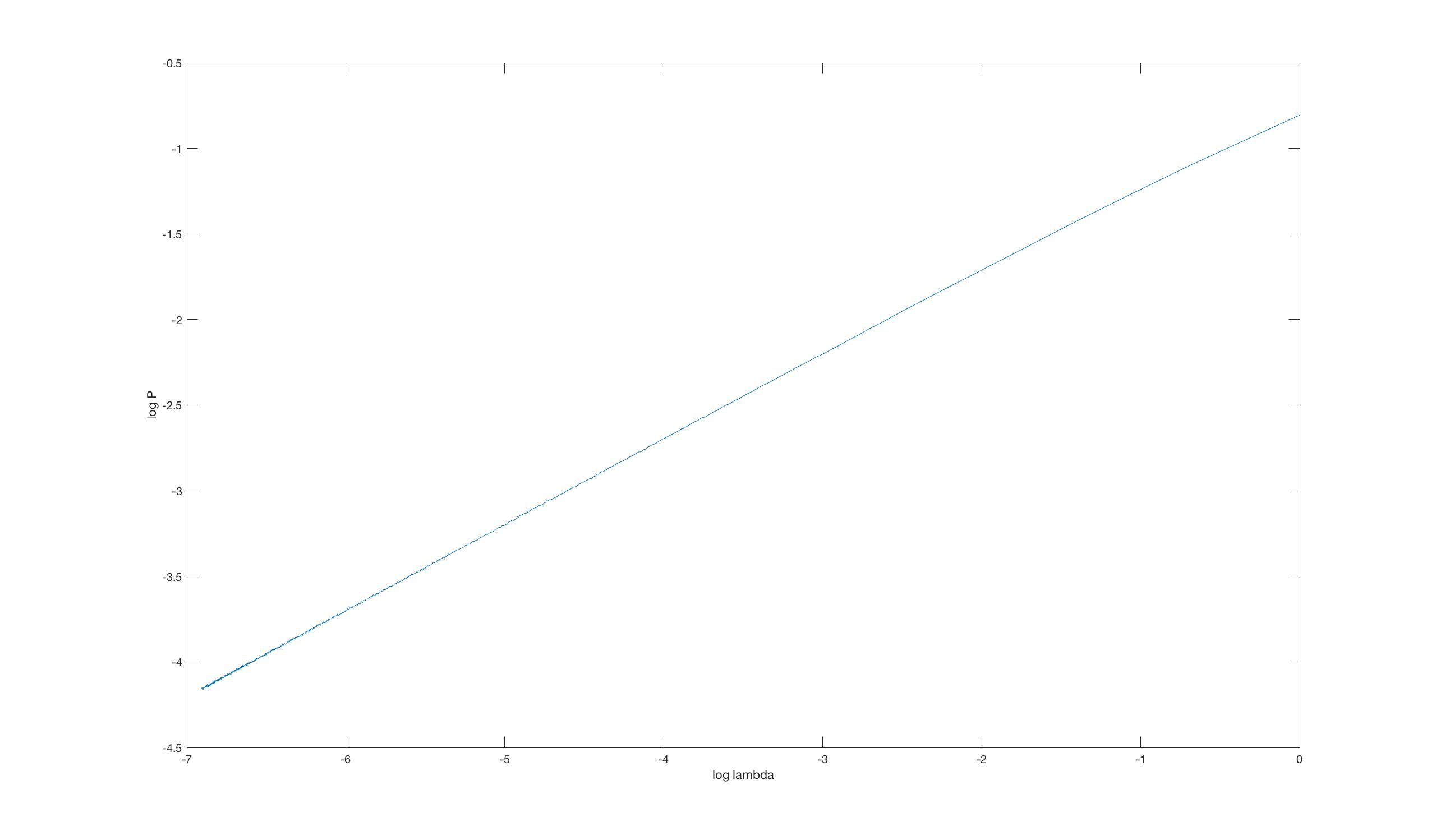
Let $X\sim\exp(\lambda)$ where the density of $\exp(\lambda)$ is parameterized as $\exp(-x/\lambda)/\lambda$. The independence of $X$ and $Z$ is also assumed. Now I'm interested in the asymptotic behavior of the following probability: $$\mathbb{P}_\lambda(Z^2\leq X)$$ as $\lambda\to0^+$.

Here $Z^2$ can also be viewed as a non-central chi-squared distribution with 1 degree of freedom and a prior distribution of $\chi_1^2$ on the non-central parameter.

Obviously this probability tends 0 as $\lambda\to0^+$. I also did some simulation study and it seems that the order should be $\sqrt{\lambda}$. However, I'm not able to come up with a rigorous proof and have no idea on where to start.

Answer 1

The probability can be computed exactly as $$ \int_{\mu=-\infty}^\infty \int_{z=-\infty}^\infty \int_{x=z^2}^\infty \frac{e^{-\mu^2/2}}{\sqrt{2\pi}} \frac{e^{-(z-\mu)^2/2}}{\sqrt{2\pi}} \frac{e^{-x/\lambda}}{\lambda} dx\, dz\, d\mu = \sqrt{\frac{\lambda}{4+\lambda}} $$ So this looks like $\sqrt{\lambda}/2$ as $\lambda\rightarrow 0^+$.