16
$\begingroup$

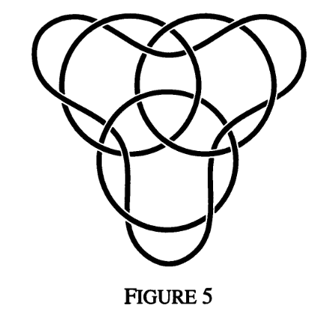
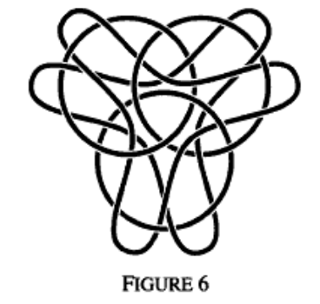
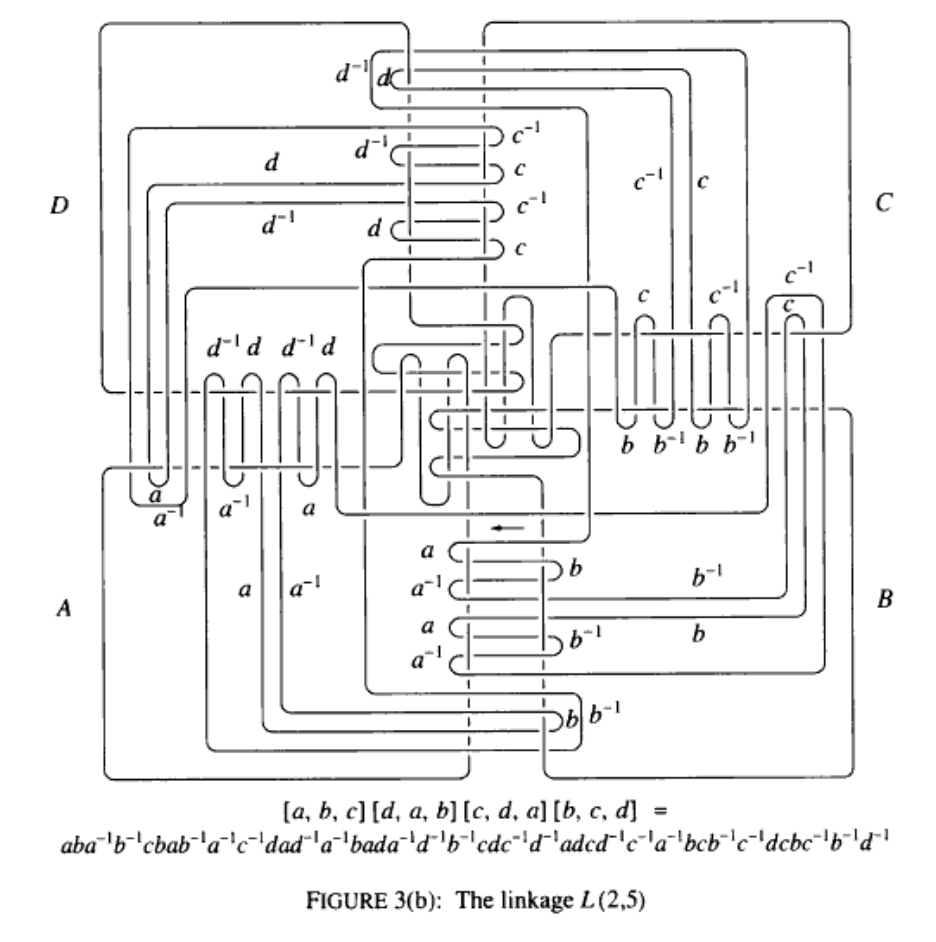
Brunnian links are well known, where deleting any component allows you to isotope the rest to an unlink. It's common to construct them by taking an $n-1$ component unlink and defining the $n$th component as a chain of commutators in the Wirtinger presentation such as $[x_1,[x_2,[x_3,x_4]]]]$

Can you construct $n \ge 4$ component links for any $n$ where deleting any two components leaves an unlink, but the link will not be split after deleting any single component?

Improve this question
$\endgroup$
0

2 Answers 2

Reset to default
21
$\begingroup$

Yes, this is done in

Penney, D.E., Generalized Brunnian links, Duke Math. J. 36, 31-32 (1969). ZBL0176.22201.

Call a link $(n,k)$-Brunnian if it has $n$ components, and every sublink with $m$ components: does not split when $k<m\le n$; completely splits when $1\le m\le k$. Then it is shown in the above paper how to use iterated commutators to build an $(n+1,k)$-Brunnian link from an $(n,k)$-Brunnian link.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Nice! It would be great to have a picture for $(n,k)=(4,2)$, if it exists... $\endgroup$
    – YCor
    Commented May 8, 2018 at 14:11
  • 1
    $\begingroup$ @YCor: yes, it would. An alternative (earlier) construction is given in [Debrunner, Hans, Links of Brunnian type. Duke Math. J. 28 1961 17–23]. Alas, the pictured link there is $(4,3)$-Brunnian. $\endgroup$
    – Mark Grant
    Commented May 8, 2018 at 14:35
  • 1
    $\begingroup$ Of course, someone who understands Penney's construction sufficiently well should be able draw a $(4,2)$-Brunnian link by adding a component to the Borromean rings which represents the appropriate product of iterated commutators. $\endgroup$
    – Mark Grant
    Commented May 8, 2018 at 14:37
18
$\begingroup$

Here is a figure of a (4,2)-Brunnian link (in the terminology of Mark Grant's answer):

figure 5 of Shephard, "Interlinked Loops"

And here is an image of a (5,3)-Brunnian link:

figure 6 of Shephard, "Interlinked Loops"

These are taken from G.C. Shephard's 2006 article "Interlinked Loops". He was not aware of the work of Debrunner and Penney at that time as he states essentially this MO question as an open problem.

However, the 2009 followup article "More Interlinked Loops" by W. R. Brakes and G. C. Shephard remedies this by giving a nice pictorial explanation of a version of Penney's construction. Here's an image of a (5,2)-Brunnian link from that paper, where I believe the letters $a,b,c,d$ correspond to elements of the Wirtinger presentation of the fundamental group of the link complement:

enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ Lovely pictures! $\endgroup$
    – Mark Grant
    Commented May 8, 2018 at 16:33
  • $\begingroup$ It might be a fun project for someone to implement Penney's construction in SageMath. $\endgroup$
    – j.c.
    Commented May 9, 2018 at 21:01

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .