Is there a smallest tile (in terms of diameter) that isohedrally tessellates the hyperbolic plane?
In this question, we ask the same question without the isohedral requirement, and the answer was no. We also asked about area instead of diameter (which turned out to not matter). I'm wondering if the isohedral requirement will lead to there being a smallest tile.
To clarify, I'm assuming that by isohedral, you mean a tiling of the hyperbolic plane in which the symmetry group acts transitively on the tiles. In this case, I can prove that the $(2,3,7)$-triangle group tiling by triangles with angles $\pi/2,\pi/3,\pi/7$ achieves the minimal diameter (as suggested in the comments).
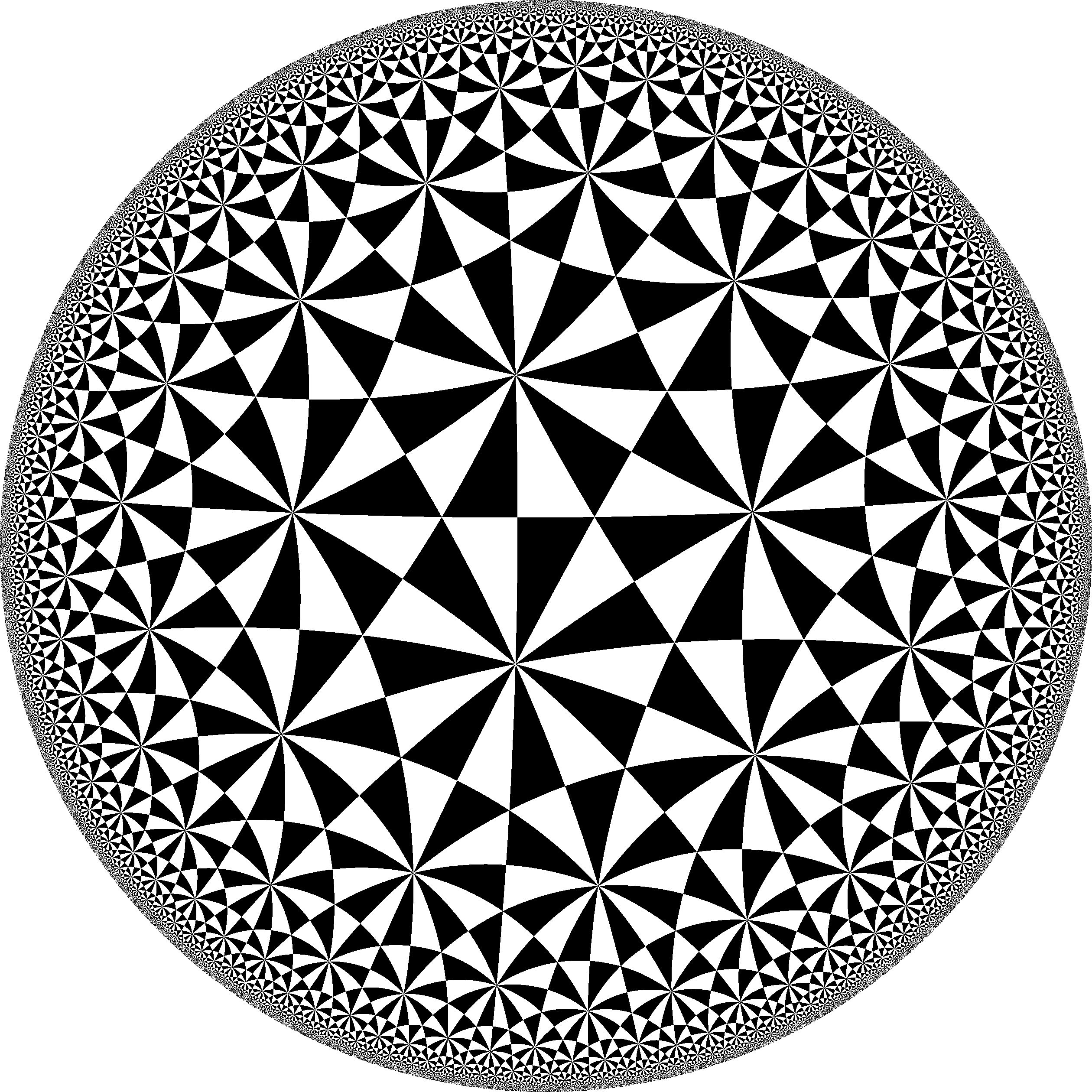
First, to answer the substance of the question, one may prove that a minimal diameter isohedral tile exists. The bound on the diameter bounds the area of the quotient orbifold. Moreover, the orbifold must be of diameter bounded by that of the fundamental domain. One may worry that a sequence of diameters of isohedral tiles approaching the infimum might not converge to the diameter of an isohedral tile. However, one may argue by the compactness of the Hausdorff metric for hyperbolic 2-orbifolds of bounded diameter and their bounded diameter fundamental domains that the sequence converges, and hence a minimal diameter will be realized.
More explicitly, let's show that the $(\pi/2,\pi/3,\pi/7)$-triangle achieves the infimal diameter of isohedral tiles. The diameter of this right hyperbolic triangle is the hypotenuse $l$ (projection to either of the legs is length-decreasing) satisfying $\cosh(l)=\cot(\pi/3)\cot(\pi/7)$ by the hyperbolic law of cosines. Then we may bound the area of a fundamental domain of diameter at most $l$, since it lies in a disk of radius $\leq l$. In fact, we may do a bit better: let $F$ be a compact fundamental domain, then there is a pair of points $x,y \in F$ such that $d(x,y)=diam(F)=d$. Then $F\subset D(x,d)\cap D(y,d)$, the intersection of two disks of radius $d$ whose centers are distance $d$ apart.
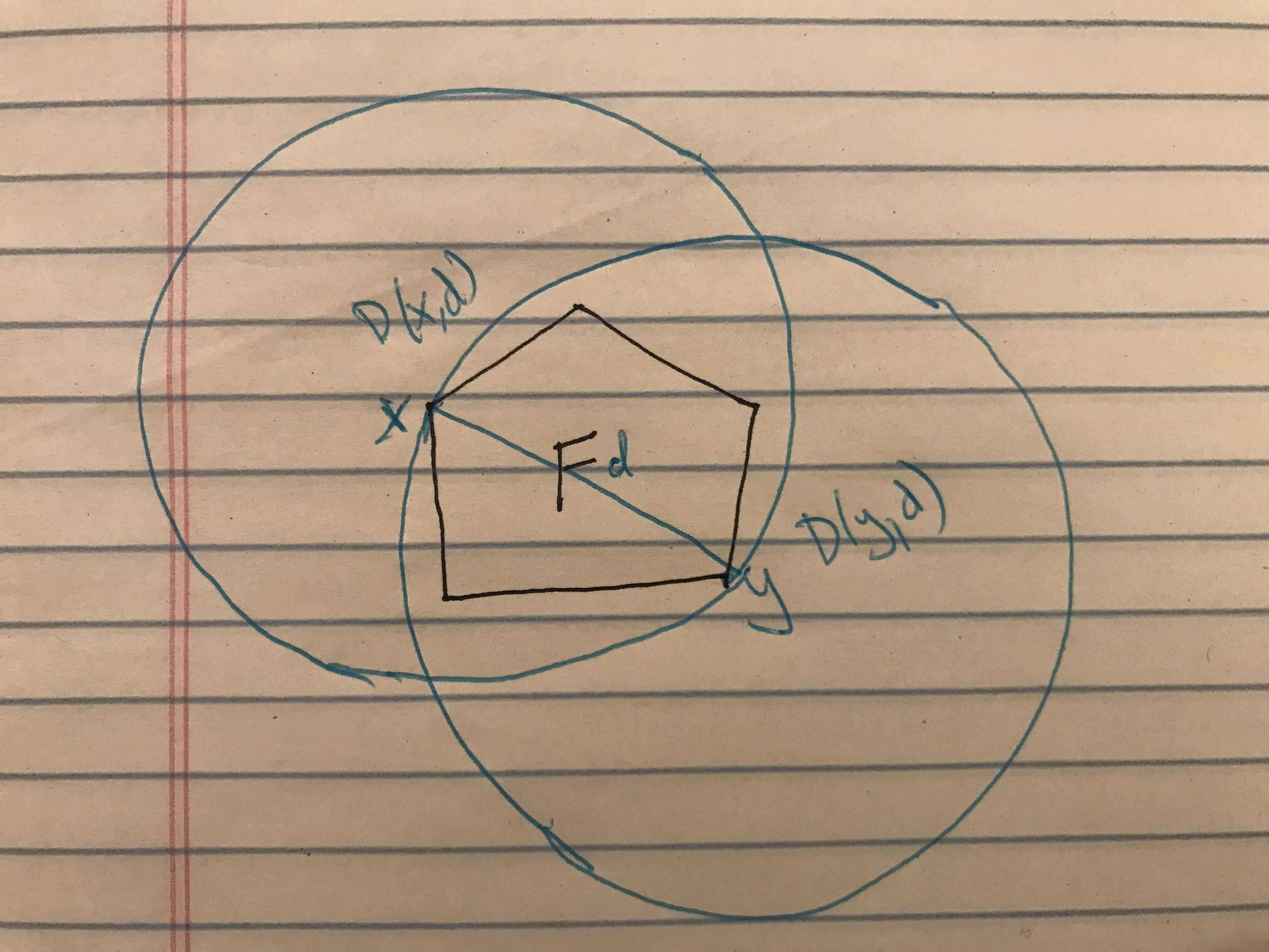
A computation in hyperbolic geometry gives the area of this lune as $4\delta (\cosh d +1/2) -2\pi$, where $\cos \delta = 1-\frac{1}{1+\cosh d}$. Clearly this area is monotonic in terms of $d$. Hence the area must be $\leq 0.472521$, which we get by setting $\cosh d= \cot(\pi/3)\cot(\pi/7)$, the diameter of the $2,3,7$-triangle. This area is less than the minimal limit area for 2-orbifolds $\pi/6\approx 0.523599$.
There are a few possible orbifold types with area $\leq 0.523599$, which one may consider by computing the possible euler characteristics and use the Gauss-Bonnet theorem. In orbifold notation, the possibilities are $pqr, *pqr$ with $1/p+1/q+1/r <1$, and $p*q$ (with certain restrictions). However, the $pqr$ orbifolds are turnovers which double cover the $*pqr$ triangle orbifolds. Hence their diameter will be at least as big as that of the $*pqr$ orbifold. Similarly, the $p*q$ orbifolds are index two covers of the $*2 p (2q)$ triangle orbifolds. Thus, we need only consider the case of triangle orbifolds.
For triangle orbifolds, the minimal diameter is that of the triangle fundamental domain, since the diameter of any fundamental domain will be greater than or equal to the diameter of the orbifold, which is the same as the diameter of the triangle.
One may then argue that the hyperbolic triangle with angles $(\pi/p,\pi/q,\pi/r)$ will have minimal diameter for $(p,q,r)=(2,3,7)$. The diameter is the maximum of the edge lengths. One may easily see that a triangle with angles $(\alpha,\beta,\gamma)$ fits inside any triangle with angles $(\alpha',\beta,\gamma)$, where then angles are acute, and $\alpha' \leq \alpha$. Along the edge opposite to $\alpha$, slide the adjacent edges apart to decrease the angle to $\alpha'$, and making the triangle larger.
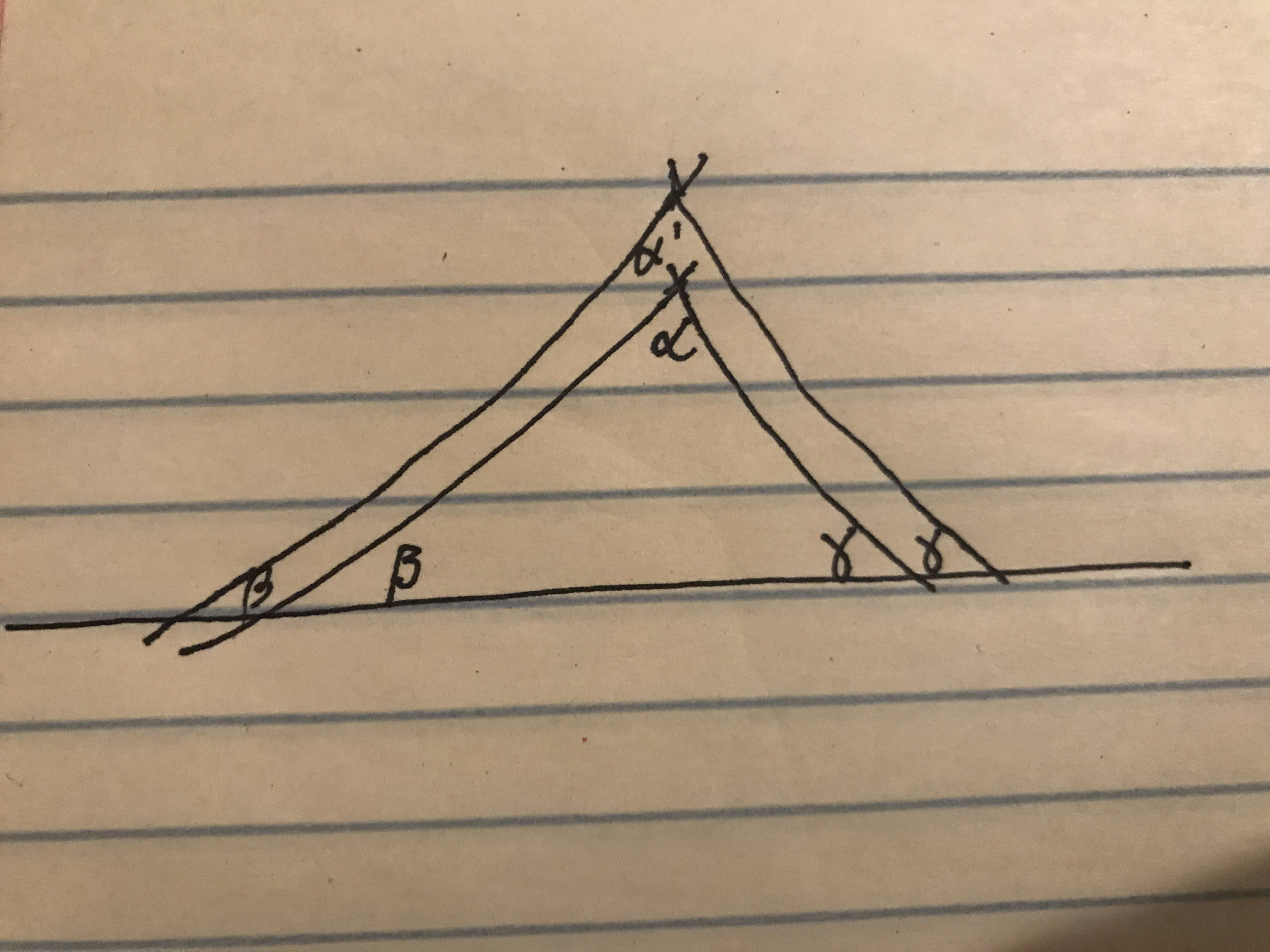
Hence one wants to maximize the angles to minimize the diameter. For triangle tiles, we therefore want to minimize $p,q,r$ with $1/p+1/q+1/r <1$. Decreasing any one of these by one will either make one of them $1$, or will give the angles of a spherical or euclidean triangle. Therefore the minimal such triples are $(2,3,7), (2,4,5), (3,4,4), (3,3,4)$, obtained from the euclidean triangles and spherical triangles by increasing one coordinate, and then eliminating non-minimal triples. The triples $(3,4,4)$ and $(3,3,4)$ give triangle orbifolds covering orbifolds $(2,4,6)$ and $(2,3,8)$ respectively, so have larger diameter, and hence are not minimal. Then a computation shows that the right triangle with angles $(\pi/2,\pi/4,\pi/5)$ has hypotenuse (and hence diameter) $\cosh^{-1}(\cot(\pi/4)\cot(\pi/5)) > \cosh^{-1}(\cot(\pi/3)\cot(\pi/7))$, so is not minimal. Thus, the $(2,3,7)$ triangle has minimal diameter.
Isohedral means the tiling has group symmetry, so the quotient is an orbifold, and the smallest area such is the 2-3-7 triangle orbifold, as pointed out by Andre Henriques. However, the $\pi/7$ angle pretty much kills any chances this has of being smallest diameter, and for that I would guess that the smallest such is the $3-3-4$ triangle orbifold.
