How many ways can you inscribe five 24-cells in a 600-cell, hitting all its vertices?

Question

You can inscribe five tetrahedra in a dodecahedron so that each vertex of the dodecahedron is the vertex of just one tetrahedron, as drawn here by Greg Egan:

Warmup question: How many ways can you do this?

I believe there are just two: the way shown here, and its mirror image.

But here's my real question. I just noticed that the above phenomenon has a four-dimensional analogue. The 24-cell and 600-cell are four-dimensional regular polytopes, and you can can inscribe five 24-cells in a 600-cell so that each vertex of the 600-cell is the vertex of just one 24-cell.

Question: How many ways can you do this?

To see that it's possible at all, note that the unit quaternions form a group that is the double cover of $\mathrm{SO}(3)$. Thus, the rotational symmetry group of the dodecahedron, $\mathrm{A}_5$, has a 120-element double cover that is a subgroup of the unit quaternions. This is called the 'binary icosahedral group' $2\mathrm{I}$. Its points, thought of as quaternions, are the vertices of the 600-cell, as drawn here by Robert Webb's Stella software:

Similarly, the double cover of the tetrahedron's rotational symmetry group $\mathrm{A}_4$ is called the 'binary tetrahedral group' $2\mathrm{T}$, and its points are the vertices of the 24-cell:

Since $\mathrm{A}_4 \subset \mathrm{A}_5$ is a subgroup with index 5, so is $2\mathrm{T} \subset 2\mathrm{I}$. Thus, the five left cosets of $2\mathrm{T}$ in $2\mathrm{I}$ give a way of inscribing five 24-cells in a 600-cell so that each vertex of the 600-cell is the vertex of just one 24-cell.

The right cosets give another method. Furthermore, there are five ways to embed $\mathrm{A}_4$ as a subgroup of $\mathrm{A}_5$, which are actually the symmetry groups of the five tetrahedra in the dodecahedron in Egan's picture above. So, we get 2 × 5 = 10 ways to inscribe five 24-cells in a 600-cell so that each vertex of the 600-cell is the vertex of just one 24-cell. I believe, but haven't carefully checked, that these are all distinct. I can't think of any other ways to do it. Thus, I conjecture that the answer to my question is: 10.

Answer 1

The 600-cell can be tiled by five 24-cells in exactly ten different ways. These are written explicitly in table 2 of "Parity proofs of the Bell-Kochen-Specker theorem based on the 600-cell", where you can also see an application of this fact to giving a proof of the Kochen-Specker theorem, ruling out the existence of noncontextual hidden variable theories in quantum mechanics.

The authors say that these ten different tilings were first discovered by P.H. Schoute and give a reference to Coxeter's book on regular polytopes.

Answer 2

$\newcommand\Z{\mathbb{Z}}$

This is an elementary answer to the warmup question.

There are precisely $10$ ways of inscribing a tetrahedron inside a dodecahedron. The symmetry group $G \subset O(3)$ of the dodecahedron acts on these tetrahedra. Note that $G = A_5 \times \Z/2\Z$. The stabilizer of a tetrahedron has to act by symmetries on the tetrahedron and thus be a subgroup of $S_4 \subset O(3)$ which acts on $T$. But $S_4$ is not a subgroup of $A_5$, and so the stabilizer (which must have order at least $10$ by the Orbit-Stabilizer theorem) is $A_4$, and the orbit of $G$ has size $10$, and $G$ is transitive.

On the other hand, since $A_4$ is contained in $A_5$, again by the Orbit-Stabilizer theorem, the action of $A_5$ is not transitive, and the $10$ tetrahedra are thus naturally divided up into two sets $U$ and $V$ of size $5$.

Let $X$ denote the set of subsets of $U \cup V$ of order $5$. Then $|X| = \binom{10}{5} = 252$. There is certainly an action of $G$ on $X$, and both $U$ and $V$ are the unique fixed points of this action. We may think of an element $x \in X$ as giving a configuration of $5$ tetrahedra inside $D$, the question is which elements of $X$ hit every vertex of $D$.

Note that $U$ and $V$ give the two configurations indicated in your answer (which are invariant under the action of $A_5$). We seek the others (if they exist).

Lemma: If $T$ and $T'$ are two tetrahedra with no vertex in common, then the $8$ vertices $S$ of $T$ and $T'$ determine both $T$ and $T'$.

Proof: Certainly if we can determine one $T$ from $S$, we can determine $T'$ as well. Take a vertex $v \in S$. It lies on some $T$. The three other vertices of $T$ lie on a plane $P(v)$ corresponding (uniquely) to $v$. The plane $P$ has $6$ vertices which are divided up into two sets of $3$. In particular, if we cannot determine $T$ from $S$ and $v$, then $S$ must contain all $6$ verticies. But now choose a different point $w$ in $S$, and we determine that $P(w)$ also contains at least $6$ points of $S$, and hence $P(v) \cap P(w)$ contains at least $4$ points of $S$. But the intersection of any two such planes is a line, and no four points of $D$ lie on a line.

Now we show the only $x \in X$ which hit every point on $P$ are $U$ and $V$. WLOG (by symmetry), we may assume that $x$ has at least $3$ tetrahedra in $U$. Let $T$ and $T'$ determine the two remaining tetrahedra in $x$ (which may or may not be in $U$). Since the complete set of vertices of $U$ is all of $D$, it follows that the two remaining tetrahedra in $U$ have the same vertices as $T$ and $T'$. Hence, by the Lemma, we must have $x = U$.

Answer 3

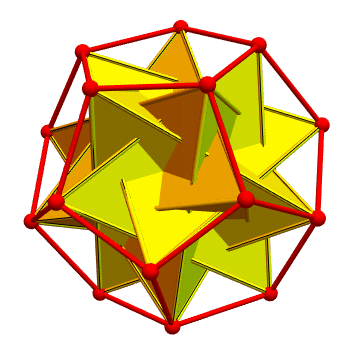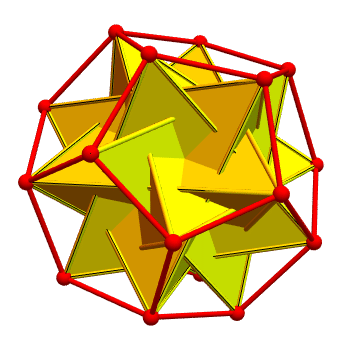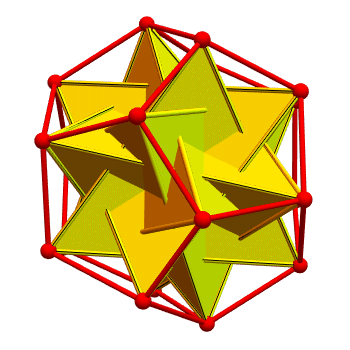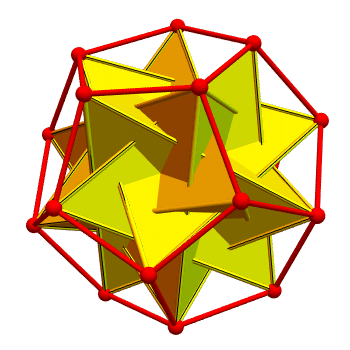
There are indeed exactly 10 ways to inscribe five 24-cells in a 600-cell, hitting all the vertices. This question was answered by Coxeter in a footnote to Section 14.3 of his Regular Polytopes, where he wrote:

Thus Schoute (6, p. 231) was right when he said the 120 vertices of {3,5,3} belong to five {3,4,3}’s in ten different ways. The disparaging remark in the second footnote to Coxeter 4, p. 337, should be deleted.

However, I knew of no published proof of this fact. So, I was pleased when David Roberson used a computer to solve this and some other puzzles which I posed on my blog.

I was even more pleased when this paper appeared, claiming to give a human-readable proof:

• Tomme Denney, Da’Shay Hooker, De’Janeke Johnson, Tianna Robinson, Majid Butler and Sandernisha Claiborne, The geometry of H₄ polytopes, December 2019. Available as arXiv:1912.06156.

They also claim that there are 25 ways to inscribe a 24-cell in a 600-cell, and that these ways can be arranged in a 5 × 5 grid, such that each row and each column gives a different way to inscribe five 24-cells in a 600-cell, hitting all the vertices. Moreover, these 10 ways are the only ways.

What follows are some puzzles of mine, and then David Roberson's solutions to some of these puzzles. Puzzles 1 and 2 remain open, so I'd love help with those.

If we take this description of the vertices of the 600-cell:

$$ \displaystyle{ (\pm \textstyle{\frac{1}{2}}, \pm \textstyle{\frac{1}{2}},\pm \textstyle{\frac{1}{2}},\pm \textstyle{\frac{1}{2}}) }$$

$$ \displaystyle{ (\pm 1, 0, 0, 0) }$$

$$ \displaystyle{ \textstyle{\frac{1}{2}} (\pm \Phi, \pm 1 , \pm 1/\Phi, 0 )} $$

and even permutations thereof, we can see that there are

$$ 2^4 = 16$$

points of the first kind,

$$ 2 \times 4 = 8$$

points of the second kind, and

$$ 2^3 \times 4! / 2 = 96 $$

points of the third kind, for a total of

$$ 16 + 8 + 96 = 120 $$

points — as desired, since the 600-cell has 120 vertices.

The 16 points of the first kind are the vertices of a 4-dimensional hypercube, the 4d analogue of a cube. The 8 points of the second kind are the vertices of a 4-dimensional orthoplex, the 4d analogue of an octahedron. Taking these together, we get the 16 + 8 = 24 vertices of a 24-cell, another regular polytope in 4 dimensions.

Note that

$$120/16 = 7\frac{1}{2}$$

Puzzle 1. Can we partition the 120 vertices of the 600-cell into the vertices of 7 hypercubes and one orthoplex?

Puzzle 2. If so, how many ways can we do this?

On the other hand,

$$120/8 = 15$$

Puzzle 3. Can we partition the 120 vertices of the 600-cell into the vertices of 15 orthoplexes? (Answer: yes.)

Puzzle 4. If so, how many ways can we do this? (Answer: 75.)

On the third hand,

$$ 120/24 = 5$$

Puzzle 5. Can we partition the 120 vertices of the 600-cell into the vertices of 5 24-cells? (Answer: yes.)

Puzzle 6. If so, how many ways can we do this? (Answer: 10.)

Here are David Roberson's answers:

I don't know how helpful this is, but (the 1-skeleton of) the 600-cell lies in a symmetric association scheme. The classes of this scheme correspond to the nine possible inner products of the vectors you wrote above (they also correspond to the nine conjugacy classes of the group $ \Gamma$). So for each possible inner product $ \alpha$, we construct a graph whose vertices are the 120 vectors above, and put an edge between two vectors if their inner product is $ \alpha$, and this gives us one class of our scheme. For instance, the 600-cell is the graph constructed in this way for $ \alpha = \Phi/2$, i.e., making vectors adjacent to the 12 vectors nearest it. But you also get an isomorphic copy of the graph of the 600-cell for $ \alpha = -1/(2\Phi)$ (this embedding gives an "optimal vector coloring" of the graph). The classes of this scheme are also exactly the orbitals (orbits on ordered pairs) of the automorphism group of the graph of the 600-cell.

This makes things a bit easier (for me) to construct the classes of this scheme in Sage. The graph of the 600-cell is built into Sage, and then I can either find the orbitals of its automorphism group, or I can compute the projection onto its second largest eigenspace. This eigenspace has dimension four, and the projection onto it is (up to a scalar) the Gram matrix of the 120 vectors above. So you can use the entries of this matrix to construct the scheme.

You can use this scheme to help with puzzles 3-6, though it seems you mostly already know the answers. I will write some things anyways in case it is of interest.

For puzzles 3 and 4, you can consider the union of all of the classes of the scheme except those corresponding to $ \alpha = \pm 1$ or $ \alpha = 0$. Call this graph $ X$. Then an orthoplex will be an independent set of size 8 in $ X$. Conversely, it is not hard to see that any independent set of size 8 in this graph must correspond to an orthoplex, since any two vectors in an independent set must be either orthogonal or antipodal. You can ask Sage and it will tell you that there are exactly 75 independent sets of size 8 in this graph, so there are 75 orthoplexes in the 600-cell, but you probably already knew this. Moreover, any partition of the 600-cell into orthoplexes corresponds to a coloring of this graph with 15 colors and vice versa. Sage tells me that there are 280 such colorings. Not exactly a proof though.

For puzzles 5 and 6, you can consider the union of all the classes of the scheme except those with $ \alpha = \pm 1, \pm 1/2, 0$. Call this graph $ Y$. A 24-cell corresponds to an independent set of size 24 in $ Y$. It is not as easy to see that any independent set of size 24 necessarily corresponds to a 24-cell, but we will get some help with this later. A partition of the 600-cell into five 24-cells corresponds to a 5-coloring of $ Y$. This is an optimal coloring of $ Y$ and Sage tells me that there are 10 of these (actually, the graph of the 600-cell also has exactly 10 5-colorings). So there are at most 10 partitions of the 600-cell into 24-cells, but you already know that there are at least 10 by the coset construction, and so every 5-coloring of $ Y$ does actually come from one of these partitions. In this case, we can use this to show that the independent sets of size 24 in $ Y$ do in fact correspond to 24-cells. This is because $ Y$ (and in fact all the graphs in the scheme) is a "normal" Cayley graph. This means that its connection set is closed under conjugation by any element of the group, i.e., it is a union of conjugacy classes. Actually every single class in the scheme corresponds exactly to a single conjugacy class in this way. I put "normal" in quotes because some people use "normal Cayley graph" to mean something else. Anyways, $ Y$ is a normal Cayley graph and it has clique number 5 and independence number 24, and 5*24 = 120 which is the number of vertices in $ Y$. This means that if $ C$ and $ S$ are maximum cliques and maximum independent sets respectively, then the sets $$ cS = \{cs : s \in S\}$$ for $ c \in C$ form a partition of $ Y$ into maximum independent sets, i.e., they give a 5-coloring of $ Y$. If there were any maximum independent set in $ Y$ that did not correspond to one of the cosets from your construction, then we could use the above construction to give a 5-coloring of $ Y$ that would necessarily be different (no color class forms a subgroup) from the 10 you constructed. Therefore, every maximum independent set of $ Y$ must correspond to a 24-cell. Sage tells me that there are 25 maximum independent sets of $ Y$, and so there are 25 24-cells contained in the 600-cell. Interestingly, the graph of the 600-cell (which is a subgraph of $ Y$) also has exactly 25 independent sets of size 24 (which is maximum) and so these must be the same.

You don't really need to use association schemes for any of this, you could have just constructed the graphs $ X$ and $ Y$ directly. But it is interesting that there is an association scheme in the background, and it could maybe be useful for turning some of these computations into human proofs, since there is a lot of stuff known about independent sets in association schemes.

By the way, for finding and counting colorings I used a Gap package called Digraphs because Sage's built-in coloring functions are not nearly fast enough.

Answer 4

"The Geometry of $H_4$ Polytopes" by Denney et al. (pages 6-8) shows the answer to Puzzle 1 is no. Each hypercube within a 600-cell appears in just one 24-cell, and any two 24-cells are either disjoint or intersect in a hexagon, where each of the three orthoplexes in a 24-cell contains two of the hexagon's six vertices. Thus each of the three hypercubes in a 24-cell, consisting of two orthoplexes, contains four of the hexagon's six vertices; but since the seven hypercubes are disjoint (by assumption) there cannot be any hexagonal intersections, and so the seven 24-cells that contain them would have to be disjoint as well, which is clearly impossible.