2
$\begingroup$

Consider a simple closed curve $C$ in $\mathbb{R}^2$. For any points $a$ and $b$ on this curve we associate point $c$ on the left (or right) side to chord $ab$ such that $\angle acb = 90^{\circ}, ac=cb.$ Continuously moving (sliding) a chord $ab$ in such a way that it goes to $ba$ (and $a(t)$ never equals $b(t)$, i.e. $\|a(t)-b(t)\|>0 ~\forall t$), the point $c$ will draw a line $L$. My hypothesis is that for any such trajectory and for any $C$, $L$ will intersect $C$. Is it true? 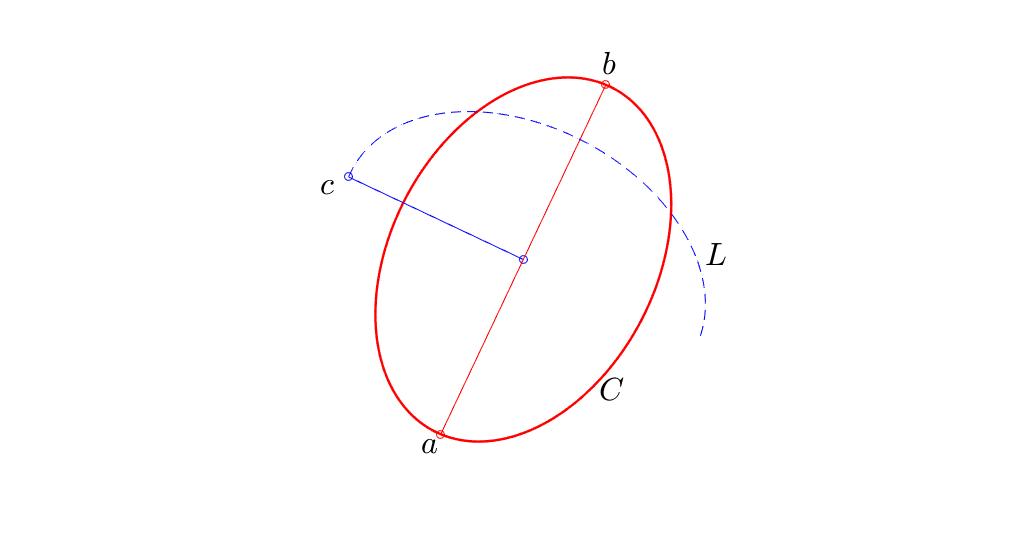enter image description here

My intuition says that curve $L$ can never be completely inside or outside $C$, but I don't now how to prove it, because geometry is not not my domain.

P.S. Curve $C$ can be non smooth.

Thanks in advance.

Improve this question
$\endgroup$
3
  • $\begingroup$ Surely for the outside case you can just take a circle and keep the length of the chord $ab$ small and fixed? $\endgroup$
    – James Smith
    Commented Aug 14, 2017 at 20:39
  • $\begingroup$ @James Smith: No, you can't keep the length small and fixed. It must become a diameter at least once. More precisely, for the circle the problem itself makes sense only if the initial chord is a diameter. Then if you shorten your chord, move it around half a circle, and blow up again, you can realize a solution in which C lies completely outside or completely inside (except at the beginning and at the end, when it is on the boundary). See my answer below. $\endgroup$
    – Luca Ghidelli
    Commented Aug 14, 2017 at 21:12
  • $\begingroup$ Forgive me then I don't think I read the question quite carefully enough (although I certainly did try). I thought it was a bit too easy... $\endgroup$
    – James Smith
    Commented Aug 15, 2017 at 15:14

1 Answer 1

Reset to default
6
$\begingroup$

No, you may make the chord close to the curve, and then transport wherever you want:enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ The above sketch works for both the 'outside case' and 'inside case', for most smooth curves with good initial position. $\endgroup$
    – Luca Ghidelli
    Commented Aug 14, 2017 at 16:27
  • $\begingroup$ Notice that in the non-smooth 'solution 1' it is crucial that there is at most one acute angle. Indeed, if there are two, and the region bounded is convex, there is no way to make C stay on the outside, as you predicted. $\endgroup$
    – Luca Ghidelli
    Commented Aug 14, 2017 at 16:43
  • $\begingroup$ Ok, thx. I will reformulate my question and I'll create new topic in few minutes. Pls look at it too ) $\endgroup$
    – makkostya
    Commented Aug 14, 2017 at 16:48

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .