Is this set of sum over a diagram is uncountable set?

Question

This problem has been posted in Mathstack but number of responses is very low (a answer is given but does not look correct).

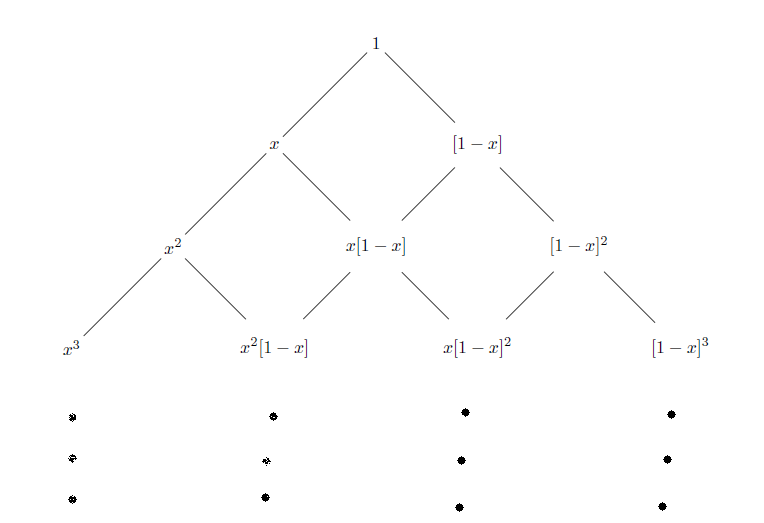
Consider the following diagram:

Let $0<x<\frac{1}{2}$

Note that $[.]$ is not box function

It is easy to see that $1$ split into $x$ and $(1-x)$. In next stage $x$ split into $x^2$ and $x(1-x)$ and $(1-x)$ split into $x(1-x)$ and $(1-x)^2$. Note that I do not write $x(1-x)$ twice, instead of that in this stage we have three values $x^2,x(1-x),(1-x)^2$. Continue this process in the next stage (see the diagram for more detail)

Now comes the important part:

Start with $1$. Then add either $x$ or $(1-x)$. If you added $x$ then next add either $x^2$ or $x(1-x)$ and if you added $(1-x)$ then up next add either $x(1-x)$ or $x^2$. Continue this process again.

For example you will get a value $1+\sum_{n=1}^{\infty}x^n$. Another one could be $1+\sum_{n=1}^{\infty}x(1-x)^{n-1}$. It depends on which path you choose. Every zigzag path will give you a $\color{red}{\text{different sums}}$.

$\color{red}{\text{different sums}}$ means sum over different zigzag path. Note that $\color{red}{\text{different sums}}$ does not mean two different path always give different values.

$\large{F}$or example $1+(1-x)\sum_{n=0}^{\infty}x^n$ and $1+x\sum_{n=0}^{\infty}(1-x)^n$ are two $\color{red}{\text{different sums}}$ but they yeilds same value $2$ i.e. $1+(1-x)\sum_{n=0}^{\infty}x^n=1+x\sum_{n=0}^{\infty}(1-x)^n=2$

Let say $T_x=\{\text{set of all $\color{red}{\text{different sums}}$ for a given $x$ }\}$

It can be easily seen there is uncountable number of $\color{red}{\text{different sums}}$.

Question: is there uncountable number of distinct elements in $T_x$ for a given $x$?

Observation:

1. It can be easily seen that minimum element of $T_x$ is $\frac{1}{1-x}$ and maximum element of $T_x$ is $\frac{1}{x}$.

2. $2\in T_x$ for every $x\in \Big(0,\frac{1}{2}\Big)$

But I could not find a way to prove or disprove uncountability. Any help would be appreciable.

Answer 1

Convert a left-right sequence to a binary expansion of a number, with lefts 0 and rights 1. I claim the sum of one sequence is less than, equal to, or greater than another if and only if the associated binary numbers are less than, equal to, or greater than. It follows that there are uncountably many possible sums as there are uncountably many unequal binary numbers.

It also follows that every number between $1/(1-x)$ and $1/x$ arises this way, as we have an increasing function $f: [0,1] \to [1/(1-x),1/x]$ that sends every binary number to the sum of the associated sequence, which is also clearly continuous as if two binary numbers agree in the first $n$ digits than the associated sums differ by at most $(1-x)^n$, hence is a homeomorphism onto a closed interval, which must be the whole interval as it contains $1/(1-x)$ and $1/x$.

To check this claim, consider two different sequences which correspond to two binary numbers, not necessarily different, and examine the first place where the two sequences diverge. We can ignore all the terms in the sum up to that place, as they are equal on both sides. We can also ignore the multiplicative factor of some power of $x$ and some power of $(1-x)$ coming from that point, so we may assume the sequences diverge at the first step - i.e. one goes left and the other goes right.

The one that goes left can take a maximum value of $1 + x (1/x) = 2$, obtained if it goes right every other step, and otherwise is less than $2$. The one that goes right can take a minimum value of $1+(1-x) 1/(1-x)=2$, obtained if it goes left every other step, and otherwise is greater than two. So the one that goes right is greater except in the special case of left followed by infinitely many rights vs. right followed by infinitely many lefts. This is identical to the situation with binary numbers, where a number starting with one is greater than a number starting with zero, unless they are $.1000\dots$ and $.0111\dots$. So the sum is greater if and only if the binary number is greater.