About left cell of a permutation

Question

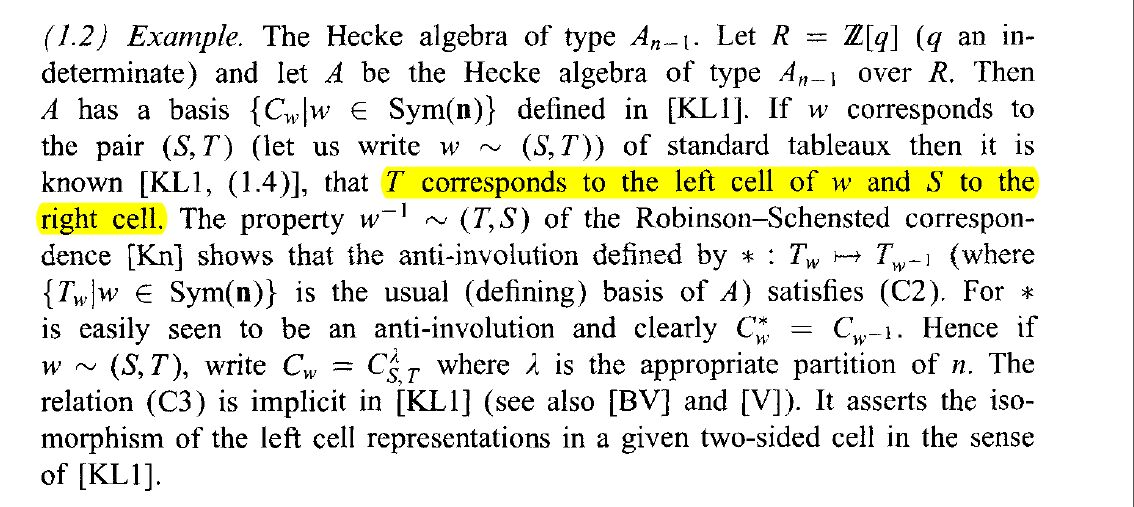
I am reading a paper Cellular algebras by J.J. Graham, G.I. Lehrer. I do not understand the follwing words labelled by yellow.

First, I know Robinson-Schensted correspondence of a permutation in the symetric group $\textrm{Sym}(\bf n)$.

For example, in $\textrm{Sym}(\bf 3)$, a permutation $\binom{123}{312}$ corresponds to a pair $(S,T)$ of standard tableaux. If $T$ correspond to the left cell of $\binom{123}{312}$ and $S$ to the right cell, I need to know whether $T$ is \begin{array}{cc} & 1 & 2 \\ & 3 & \end{array} and $S$ is \begin{array}{cc} & 1 & 3 \\ & 2 & \end{array}?

Thank you very much.

Answer 1

The KL right cell is the collection of permutations that have the same insertion tableau S from RSK algorithm, the left cell is those of the same recording tableau T. KL right(left) cell are the equivalence classes of KL right(left) preorder on $\mathfrak{S}_n$.

The S of 312 is : $$1 \;\; 2$$ $$3\;\;\;$$ The T is:$$1 \;\; 3$$ $$2\;\;\;$$