Euler product approximation of the Riemann zeta function

Question

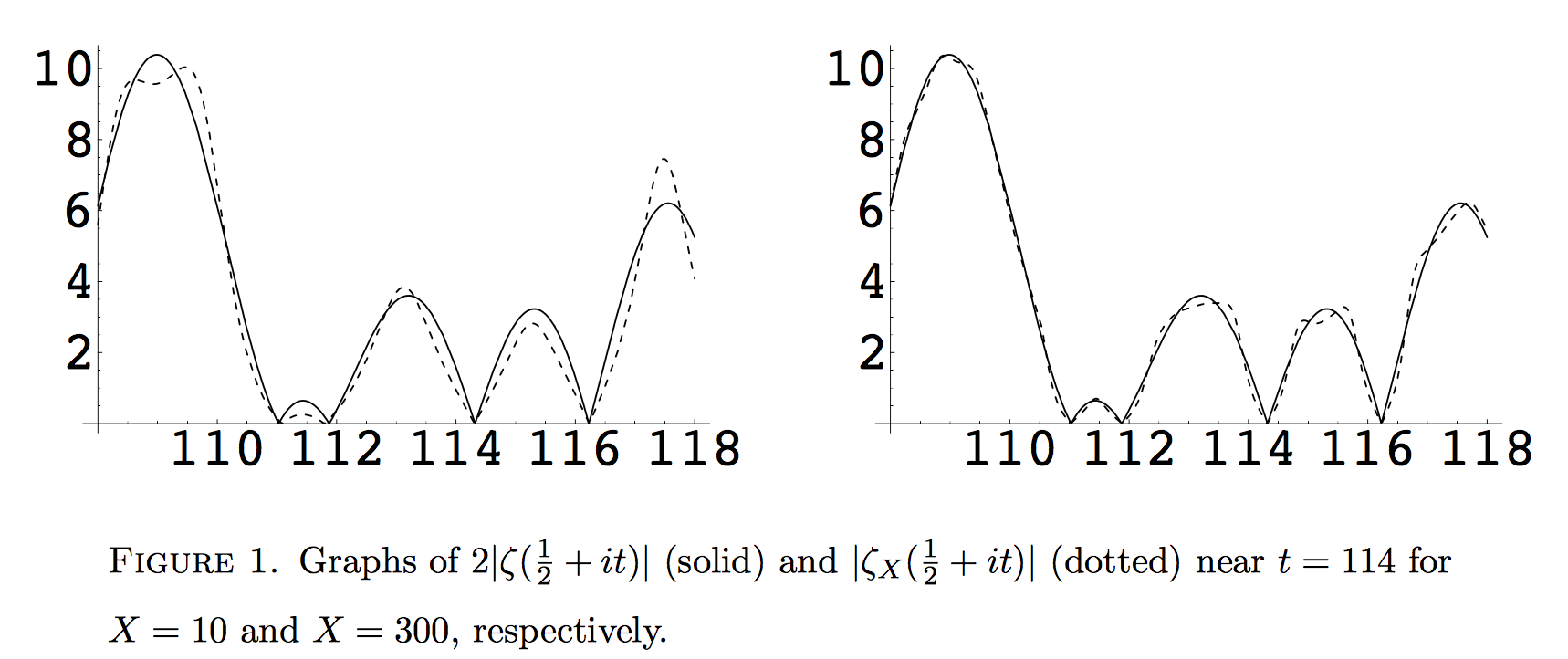
It is well-known that the Riemann zeta function can be approximated in the critical strip by a finite Dirichlet polynomial. More precisely, $$ \zeta(\sigma+it) = \sum_{n \leq X} n^{-\sigma-it} + \frac{X^{1-\sigma-it}}{\sigma+it-1} + O ( X^{-\sigma}) $$ uniformly for all $X$ satisfying $2 \pi X / C \geq |t|$, where $C$ is a fixed constant greater than 1. (This is Theorem 4.11 in Titchmarsh's book). In particular this holds for $\sigma = 1/2$.

Question: is there a similar (simple) approximation of zeta by a finite Euler product?

Answer 1

See Finite Euler products and the Riemann Hypothesis by S. M. Gonek (2007):

We show that if the Riemann Hypothesis is true, then in a region containing most of the right-half of the critical strip, the Riemann zeta-function $\zeta$ is well approximated by short $X$-term truncations $\zeta_X$ of its Euler product. Conversely, if the approximation by products is good in this region, the zeta-function has at most finitely many zeros in it.

This approximation also provides insight into why the zeroes on the critical line "repel", in the sense of level repulsion in the Gaussian Unitary Ensemble.