3
$\begingroup$

The most famous version of packing problems deals with perfectly symmetrical shapes such as spheres. But how about anisotropic shapes? More prcisely, if we want to compare spherocylinders (cylinders with spherical caps) with ellipsoids, individually, to my understanding an ellipsoid and a spherocylibder have the same symnetries from a group theoretic point of view. But does anything change if we now consider the packing of many of such objects? That is, do two ellipsoids, compared to two spherocylinders, exhibit different symmetrical or packing properties?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
4
$\begingroup$

Not a direct answer, but this paper indicates considerable differences between packing densities of ellipsoids and spherocylinders, for both random and ordered packings. So, under one interpretation, the answer to your question, "does anything change?" is Yes. (But perhaps that's not what you mean by "anything.")

  • Li, S., Zhao, J., Lu, P., & Xie, Y. (2010). Maximum packing densities of basic 3D objects. Chinese Science Bulletin, 55(2), 114-119. ResearchGate link.

Abstract. Numerical simulation results show that the upper bound order of random packing densities of basic 3D objects is cube (0.78) > ellipsoid (0.74) > cylinder (0.72) > spherocylinder (0.69) > tetrahedron (0.68) > cone (0.67) > sphere (0.64), while the upper bound order of ordered packing densities of basic 3D objects is cube (1.0) > cylinder and spherocylinder (0.9069) > cone (0.7854) > tetrahedron (0.7820) > ellipsoid (0.7707) > sphere (0.7405); these two orders are significantly different. The random packing densities of ellipsoid, cylinder, spherocylinder, tetrahedron and cone are closely related to their shapes. The optimal aspect ratios of these objects which give the highest packing densities are ellipsoid (axes ratio = 0.8:1:1.25), cylinder (height/diameter = 0.9), spherocylinder (height of cylinder part/diameter = 0.35), tetrahedron (regular tetrahedron) and cone (height/bottom diameter = 0.8).

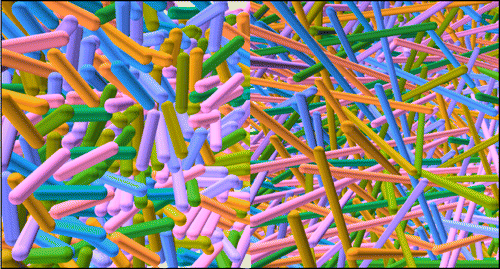
Just for fun, here's a nice image of two different random spherocylinders packings, from: Ferreiro-Córdova, Claudia, and Jeroen S. van Duijneveldt. "Random packing of hard spherocylinders." Journal of Chemical & Engineering Data 59.10 (2014): 3055-3060. Journal link.

          Fig

Update (in response to comment: "is there any intuition as to how the shape of ellipsoid allows for other types of packing?"). See the figure below from the indicated paper, showing that "a remarkable maximum density of 0.7707 is achieved ..., when each ellipsoid has 14 touching neighbors."

          Fig4
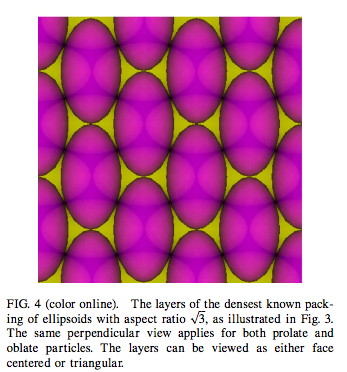
Donev, A., Stillinger, F. H., Chaikin, P. M., & Torquato, S. (2004). Unusually dense crystal packings of ellipsoids.
Physical Review Letters, 92(25), 255506. PDF download.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Thanks a lot, these are wonderful references that I'll look into. A partial reformulation of whether "anything" changes, would be to ask: Suppose we've found the densest optimal packing of spherocylinders, will the found configuration be also the densest packing of ellipsoids? Something that may be expected given that these shapes have individually the same symmetries (?). $\endgroup$
    – user88381
    Commented Feb 20, 2017 at 16:45
  • 1
    $\begingroup$ @user929304: The evidence suggests No. The densest ordered packings differ. But it would take some digging in the literature to understand in detail how they differ. $\endgroup$
    – Joseph O'Rourke
    Commented Feb 21, 2017 at 0:16
  • $\begingroup$ I imagine in the limit of long aspect ratios (approaching thin rods/needles) this difference ought to vanish. But on the more interesting side, namely when the aspect ratio is well finite, is there any intuition as to how the shape of ellipsoid allows for other types of packing? For example, one difference is that with cylinder type shapes one can tile the space but with ellipsoids there are always voids left in the packing however one goes about it. Does this mean that ellipsoids leave a greater room for the mobility of other ellipsoids in vicinity, when they tend to pack along long axis? $\endgroup$
    – user88381
    Commented Feb 21, 2017 at 15:00

You must log in to answer this question.