How can one represent vector addition diagrammatically in categorical quantum mechanics?

Question

I am currently studying the use of string diagrams and monoidal categories in quantum mechanics, in particular the recent papers of Coecke & Kissinger, with an eye towards potential use in specifying and analyzing protocols in cryptography. In particular, the monoidal product for string diagrams should be thought of as the tensor, not the direct sum.

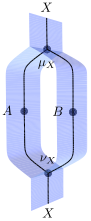
My question is whether/how the additive vector structure can be represented diagrammatically. In the simplest case, suppose I have two linear maps $A:X\to Y$ and $B:X\to Y$, represented as string diagrams:

$\overset{X}{\longrightarrow}\fbox{$A$}\overset{Y}{\longrightarrow}$

$\overset{X}{\longrightarrow}\fbox{$B$}\overset{Y}{\longrightarrow}$

Is there any way to build up a diagram from these pieces which represents the map $A+B:X\to Y$?

Answer 1

The short answer is yes, you put $A+B$ in the box.

To be more specific, in the graphical calculus for monoidal categories strings correspond to equivalence classes of objects (in your case $X$ and $Y$) in some monoidal category (We'll call it $\mathcal C$ just to name it) and the ticket or box (in your case labelled by $A$ and $B$) represents a morphism between them, i.e. that $A, B \in Hom_{vec}(X,Y)$. That subscript $vec$ is important because specifically, for your objects $X$ and $Y$, morphisms between them are linear and so are additive, i.e. if $A, B \in Hom_{vec}(X,Y)$ then $A+B \in Hom_{vec}(X,Y)$. Thus, since the ticket can be labelled by any morphism between $X$ and $Y$, one does not need to add a new convention to the graphical calculus.

Answer 2

A more interesting way to go, which we haven't even fully developed yes, is to use W-spiders (a.k.a. anti-special ones), which are introduced here:

https://arxiv.org/abs/1002.2540

since they allow for diagrammatic representation of addition:

https://arxiv.org/abs/1103.2812

Answer 3

You can use sheet diagrams for bimonoidal categories for this.

The tensor product and the direct sum give a bimonoidal structure on $\text{FVect}$ (meaning that one distributes over the other). On top of that, you can define maps $\nu_X : X \rightarrow X \oplus X$ and $\mu_X : X \oplus X \rightarrow X$ by $\nu_X(x) = x \oplus x$ and $\mu_X(x \oplus y) = x + y$.

With these definitions, $A + B = \mu_X \circ (A \oplus B) \circ \nu_X$. As a sheet diagram, this gives you:

This notation lets you draw $A + B$ compositionally while still being able to decompose objects using the tensor product on each sheet.

Answer 4

Clearly either of

$$\overset{X}{\longrightarrow}\fbox{$A$}\overset{Y}{\longrightarrow}\\+\\ \overset{X}{\longrightarrow}\fbox{$B$}\overset{Y}{\longrightarrow}$$

or

$$\overset{X}{\longrightarrow}\fbox{$A+B$}\overset{Y}{\longrightarrow}$$

are acceptable.

Another way to write a sum, say of $A_i:X\to Y$ for $i\in\{1,\dots,n\}$, is to let $A$ be the map $X\otimes \Bbb C^n\to Y$ such that $A(-,e_i)=A_i(-)$, and also let $\eta: \Bbb C\to\Bbb C^n$ be the map sending $1$ to $(1,\dots,1)$. Then we have

$$\overset{X}{\longrightarrow}\fbox{$\sum_iA_i$}\overset{Y}{\longrightarrow}\quad=\quad\begin{align}\overset{X}{\longrightarrow\longrightarrow}\\ \fbox{$\eta$}\overset{\Bbb C}{\rightarrow}\end{align}\fbox{$\begin{align}\\\quad A\quad\\\\\end{align}$}\overset{Y}{\longrightarrow}$$

if you'll forgive my awful formatting (or, if you won't forgive it, $\sum_iA_i=A\circ(\mathrm{id}_X\otimes\eta)$).

Answer 5

Btw, are you reading CQM I and CQM II? Since the actual meat is in the forthcoming CQM III, and in our forthcoming book. When one starts to use the categorical algebra of complementary observables, things for which one uses sums like control operations can actually be down without. You can see this here in section 12.1:

http://iopscience.iop.org/article/10.1088/1367-2630/13/4/043016/meta