Geodesics (with the same limit point) in a surface group of genus two

Question

Consider a discrete Gromov-hyperbolic group $\Gamma$ (and its Cayley graph $\mathcal{G}$ w.r.t. some generating set). The notion of Gromov-boundary, indicated with $\partial\Gamma$, is naturally associated with the group.

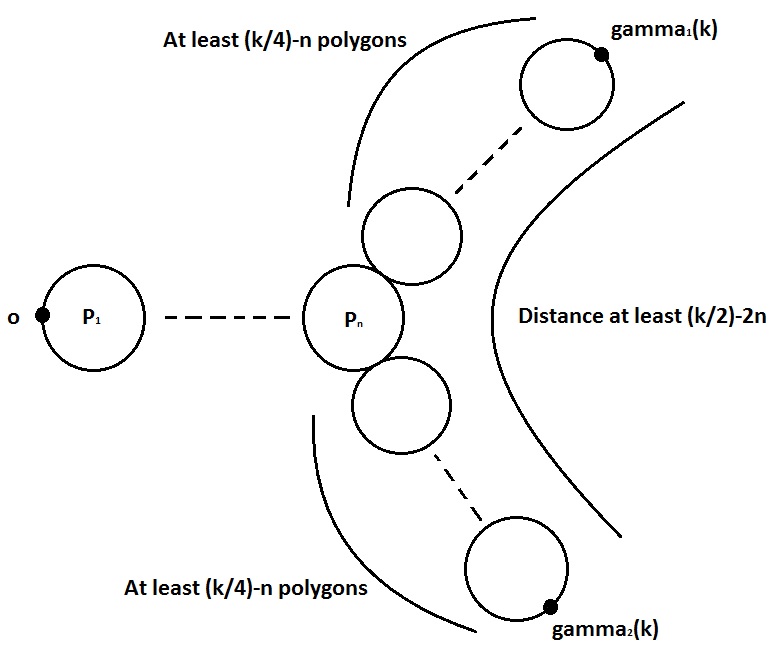
It is well known that two geodesics starting at the same point (assume a vertex) in the graph $\mathcal{G}$ and having the same limit point at infinity (i.e., on the boundary) have to stay at a bounded distance: if $\gamma_1$, $\gamma_2$ are s.t. $\gamma_1(0)=\gamma_2(0)$ and $\gamma_1(\infty)=\gamma_2(\infty)$, then, there exists $K>0$ s.t. $\sup_{t\in [0,\infty)}\mathrm{dist}(\gamma_1(t),\gamma_2(t))<K$, where "$\mathrm{dist}$" denotes the path-distance in the graph.

Let us now specialize to the case where $\Gamma$ has the presentation $\langle a_1,b_1,a_2,b_2\mid [a_1,b_1][a_2,b_2]\rangle$. This is the fundamental group of an orientable, connected and compact surface of genus $2$, for example, a connected sum of two tori.

The Cayley graph of this group is (dual to) a tessellation of the hyperbolic disc by (hyperbolic) octagons, obtained joining at each vertex eight different octagons.

It can be proved that two geodesics $\gamma_1,\gamma_2$ s.t. $\gamma_1(0)=\gamma_2(0)$ and $\gamma_1(n)=\gamma_2(n)$ for some $n\in\mathbb{N}$ satisfy $\sup_{t\in[0,n]}\mathrm{dist}(\gamma_1(t),\gamma_2(t))\leq 4$, meaning in particular that two vertices on the geodesics corresponding to the same parameter $t\in\mathbb{N}$ are at most four edges apart.

My claim is that the same bound holds for the case where $\gamma_1(\infty)=\gamma_2(\infty)$, but $\gamma_1(t)\neq \gamma_2(t)$ for $0<t<\infty$.

Frist of all, I wonder if this claim is actually true. I ask if someone is aware of a counterexample. Then I ask if someone is already in possess of a proof of this claim. I strike that I am referring to a very specific case: I'm interested in the particular group presented above.

Answer 1

This is a consequence of Strebel's classification of geodesic triangles in C'(1/6) polygonal complexes; see Ghys and de la Harpe's book, Sur les groupes hyperboliques d'après Mikhael Gromov.

Notice that the cases IV and V are not possible in the hyperbolic plane because the tessellation is T(4).

Let $P_1, \ldots, P_n$ be a sequence of polygons which intersect both $\gamma_1$ and $\gamma_2$, where $\gamma_1(0)=\gamma_2(0)=o \in P_1$, and suppose that further $P_n$, no polygon intersect both $\gamma_1$ and $\gamma_2$. Next, take $k$ large enough and consider a geodesic triangle $[o, \gamma_1(k), \gamma_2(k)]$. Following the classification above, we are in case II or III, where the central polygon is $P_n$. Finally, conclude that it is possible to find a $k$ so that $d(\gamma_1(k),\gamma_2(k))$ is arbitrarily large. The situation is roughly the following:

As a consequence, $\gamma_1(+ \infty) \neq \gamma_2(+ \infty)$.

Consequently, if you suppose that $\gamma_1(+ \infty)= \gamma_2(+ \infty)$, then the classification implies that any geodesic triangle $[o,\gamma_1(k),\gamma_2(k)]$ must have the form I, so that $\gamma_1(k)$ and $\gamma_2(k)$ belong to a common polygon. Hence $d(\gamma_1(k), \gamma_2(k)) \leq 4$.

There are probably some missing cases in the previous argument, but I think a rigourous proof can be extracted from it. (Because you are working in the hyperbolic plane, Strebel's classification can probably be proved directly and elementarily.)