6
$\begingroup$

This is related to my last question that went sadly unanswered (Is my matrix perturbation analysis legitimate?).

Again, I have a matrix whose entries are integer polynomials in a single real variable. In this situation, the determinant and (the entries of) any suitably normalised eigenvectors associated with simple eigenvalues vary continuously with the variable.

However it may be the case with a higher-dimensional eigenspace that there is no possible choice of basis such that each vector in the basis has continuously varying entries. The small examples that I know have the property that at the point of discontinuity two eigenvalues collide forming a 2-dimensional eigenspace and then as the parameter increases further, they split again but now with two completely different eigenvectors.

However I cannot find any criteria that enable one distinguish between the two situations - when it is possible to find a "continuous basis" and when it isn't?

Numerically (by computer) my troublesome eigenspace admits a continuous basis, but numerical is not enough...

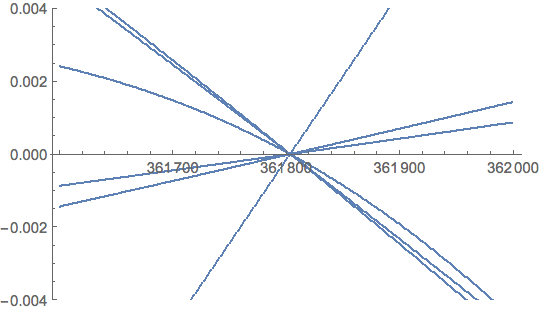
ADDED INFORMATION

Here is a Mathematica plot of the 6 smallest (in magnitude) eigenvalues of my matrix as the variable varies from less-than to greater-than the point at which the eigenvalues coincide.

enter image description here

Improve this question
$\endgroup$
4
  • $\begingroup$ This may be relevant. I think it is more worrisome that the eigenvectors may collide: consider the 2x2 matrix $[x, 1; 0,0]$. Away from $x = 0$ you have two distinct eigenvectors. At $x = 0$ you only have one eigenvector $[1;0]$. Do you count this as having "continuous eigenvectors"? Also, since in your previous question you make no assumption that your matrix is symmetric: you are not even guaranteed the existence of an eigenbasis. $\endgroup$
    – Willie Wong
    Commented Sep 8, 2016 at 15:49
  • 2
    $\begingroup$ Are you familiar with Kato's Perturbation Theory for Linear Operators (Ch 2), [downloadable from U. of Edinburgh](www.maths.ed.ac.uk/~aar/papers/kato1.pdf) . $\endgroup$
    – Keith McClary
    Commented Sep 8, 2016 at 19:27
  • $\begingroup$ @WillieWong Changing dimension is definitely one case where the answer is "can't be done". Fortunately I can now show that my matrix avoids this problem. $\endgroup$
    – Gordon Royle
    Commented Sep 8, 2016 at 22:49
  • 1
    $\begingroup$ @KeithMcClary Familiar is probably too strong a word to describe my relationship with Kato, but I do know of its existence. $\endgroup$
    – Gordon Royle
    Commented Sep 9, 2016 at 6:53

1 Answer 1

Reset to default
1
$\begingroup$

The beginning of the following paper has a quite complete overview on available results:

  • Andreas Kriegl, Peter W. Michor, Armin Rainer: Denjoy-Carleman differentiable perturbation of polynomials and unbounded operators. Integral Equations and Operator Theory 71,3 (2011), 407-416. (pdf)
Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ This seems to cover only self-adjoint or normal $A(t)$ (though I can't quite tell because the main theorem has many different parts). $\endgroup$
    – Gordon Royle
    Commented Sep 9, 2016 at 1:12

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .