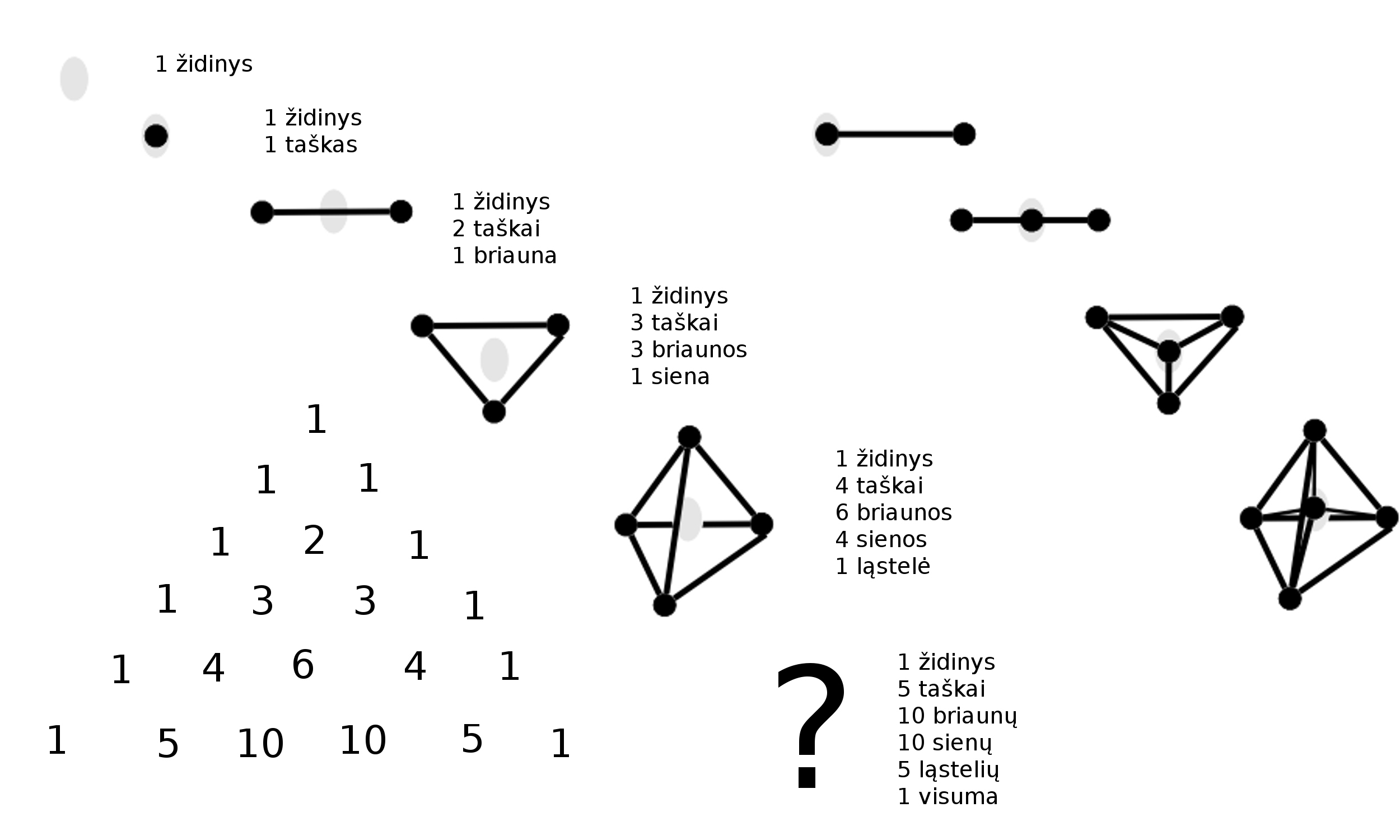
The number of $k$-simplex elements in an $n$-simplex is counted by the binomial coefficient $\binom{n+1}{k+1}$. For example, the $3$-simplex is the tetrahedron, which has the following elements: $4$ vertices ($0$-simplexes), $6$ edges ($1$-simplexes), $4$ triangular faces ($2$-simplexes) and also itself, $1$ tetrahedron ($3$-simplex). Apparently, in every $n$-simplex there should also be one $-1$-simplex.
The Wikipedia article Simplex cites Combinatorial Algebraic Topology by Dmitry Kozlov which terms the $-1$-simplex the "empty simplex" of dimension $-1$, but does not consider it further. See page 22, Remark 2.3 and Remark 2.4.
I am interested whether the $-1$-simplex has ever been interpreted to be significant in any way.
I'm also interested to learn of the significance of the unique center (or barycenter) of any simplex because that's how I'm interpreting the $-1$-simplex.
I am interested in the regular simplex family because of its relevance for the "field with one element". If we think of the family as a whole, then it is interesting how the $n+1$st simplex arises from the $n$th simplex, namely by adding a new vertex along with edges to each of the existing vertices. Indeed, if we give the $n+1$st vertex the weight $q^{n}$ and we balance that with a weight $q^{-1}$ to each of the n new edges, then the weights of the $k+1$ simplex elements yield the Gaussian binomial coefficients (the $q$-analog of the binomial coefficients) $\binom{n+1}{k+1}_{q}$. In other words, the $n+1$-simplex can be interpreted as a total order of its $n+1$ vertices as given by their order of creation. When $q\rightarrow 1$, then the total order is lost and we are left with an unordered set of vertices. Similarly, if we interpret $\binom{n+1}{k+1}_{q}$ as the number of $k$-dimensional subspaces of an $n$-dimensional vector space over a finite field $F_{q}$ with $q$ elements, then so long as we have a real choice of scalars, any basis is implicitly an ordered list: we fix a first basis element, a second, a third, and the count grows accordingly by powers of $q$. But when $q\rightarrow 1$, we have only one scalar to choose from, we have no real choice, and so the ordered list becomes an unordered set.
The $0$-simplex (an isolated point) should arise from the $-1$-simplex. If we define the $-1$-simplex as the (pointless) center of the simplex, then we can imagine the unique center as ever generating a new vertex (and a new dimension) and then moving to the new center. Indeed, we can imagine ourselves viewing the simplex and center as if from above. Viewed from above, the center of a line segment becomes the top of an equilateral triangle, and the center of an equilateral triangle becomes the top of a tetrahedron. Indeed, we can see three of the faces of the tetrahedron. If we imagine the center of a tetrahedron, then we can see that it divides the tetrahedron into four smaller tetrahedrons, which along with the original tetrahedron become the five cells of the $4$-simplex as soon as the center moves to create a new $5$th vertex in a new $4$th dimension. Here is a picture in Lithuanian. :)
In my essay, Discovery in Mathematics: A System of Deep Structure, I note that we can generate the cross-polytopes by having the center create pairs of vertices. I also discuss the totality, the dual of the center, the unique (but growing) simplex which consists of all of the vertices. Together, the four combinations of center (exists or not) and totality (exists or not) accord with four polytope families: simplexes, cross-polytopes, cube and demicubes. I speculate that they might ground an intuitive explanation for the four classical Lie algebras/groups and four associated geometries: affine, projective, conformal and symplectic.
Finally, I note that the Euler characteristic $V-E+F=2$ might be more elegant if we included the Center and Totality, namely: $C-V+E-F+T = 0$. In the Wikipedia article on the Euler characteristic I see alternating formulas where it seems this is done. Again, it is interesting what $C$ and $T$ are understood to mean.
My Ph.D. thesis was in algebraic combinatorics. All that I know of algebraic geometry and algebraic topology is what I read in Wikipedia and watch on YouTube. :) So I appreciate directions that you may point me in. Thank you!