Xi Function on Critical Strip - Mellin Transform

Question

Story

I'm trying to prove following identity

$$\int_0^\infty \frac{\Xi(t)}{t^2 + \frac{1}{4}} \cos(xt) dt = \frac{1}{2} \pi (e^{\frac{1}{2}x} - 2e^{-\frac{1}{2}x} \psi(e^{-2x}))$$

where

$$\psi(x)=\sum_{n = 1}^{\infty} e^{-n^2 \pi x}$$

and

$$\Xi(t) = \xi(\frac{1}{2} + it)$$

is the xi function on the critical line.

Problem

I only don't understand the following equality

$$-\frac{1}{4 i \sqrt{y}} \int_{\frac{1}{2} - i\infty}^{\frac{1}{2} + i\infty} \Gamma(\frac{1}{2}s) \pi^{-\frac{1}{2}s} \zeta(s) y^s ds = -\frac{\pi}{\sqrt{y}} \psi(\frac{1}{y^2}) + \frac{1}{2} \pi \sqrt{y}.$$

Where does latter summand $\frac{1}{2} \pi \sqrt{y}$ come from? When I write $(\frac{1}{y})^{-s}$ and substitute with $s = 2w$ I only get the first summand. We know that by the Mellin transform theorem $\psi(x)$ can be recovered by the Mellin transform integral over $\Gamma(s) \pi^{-s} \zeta(2s)$.

Source

This is from Titchmarsh's book "The Theory of the Riemann Zeta-function" p. 35−36

Answer 1

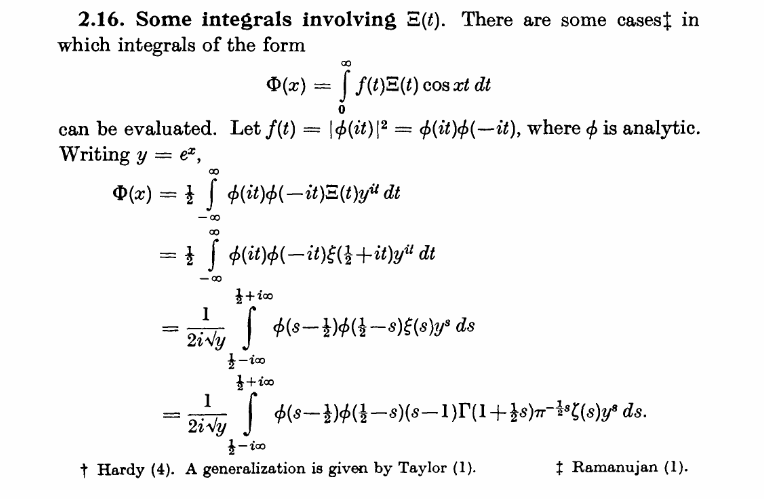
Let $f(x) = 2\sum_{n = 1}^\infty e^{- \pi n^2 x^2}$ and $E(s) = \pi^{-s/2} \Gamma(s/2)\zeta(s)$.

For $Re(s) >0$ : $2\int_0^\infty x^{s-1} e^{-\pi n^2 x^2} dx = n^{-s} \pi^{-s/2}\Gamma(s/2) $ so we have for $Re(s) > 1$ :

$$E(s) = \Gamma(s/2) \pi^{-s/2} \zeta(s) = 2\sum_{n=1}^\infty \int_0^\infty x^{s-1} e^{-\pi n^2 x^2} dx = \int_0^\infty f(x) x^{s-1} dx \quad (1)$$

By inverse Mellin transform : $$f(x) = \frac{1}{2 i \pi}\int_{\sigma-i\infty}^{\sigma+i\infty} E(s) x^{-s} ds$$

but this is only true for $\sigma > 1$, since $E(s)$ has a pole at $s=1$.

Note that for $Re(s) > 1$ : $\displaystyle\quad\frac{1}{s-1} = \int_0^1 x^{s-2} dx = \int_0^\infty \frac{1_{x < 1}}{x} x^{s-1} dx $,

and for $Re(s) < 1$ : $\displaystyle\quad-\frac{1}{s-1} = \int_1^\infty x^{s-2} dx = \int_0^\infty \frac{1_{x > 1}}{x} x^{s-1} dx $

Hence, at least for $Re(s) > 1$ :

$$E(s) - \frac{1}{s-1} = \int_0^\infty \left( f(x)- \frac{1_{x < 1}}{x}\right) x^{s-1} dx \qquad (2) $$

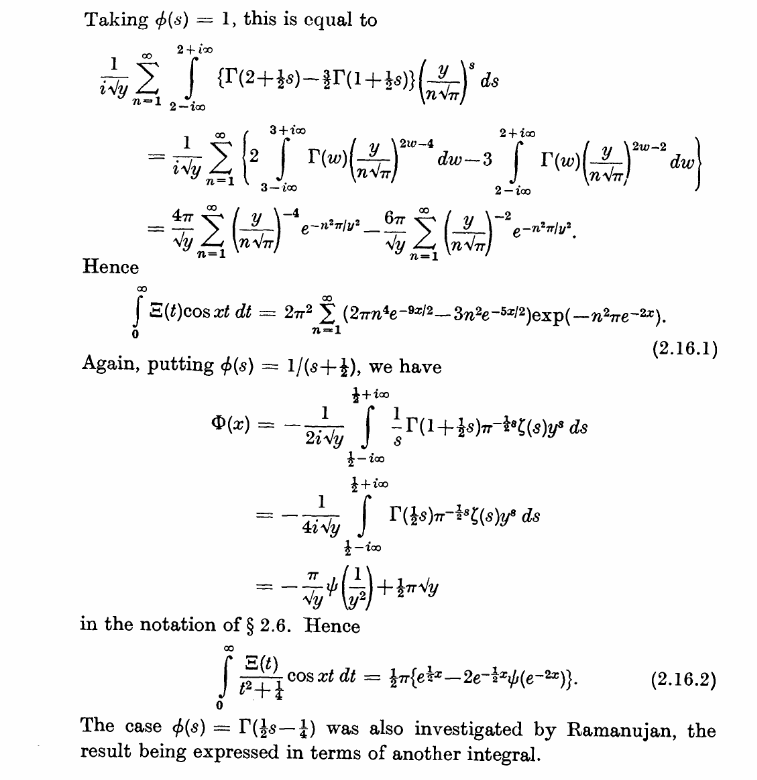
indeed, this is true also for $Re(s) > 0$ (see below) and we get, for $Re(s) \in (0,1)$ :

$$E(s) = \int_0^\infty \left( f(x) - \frac{1}{x}\right) x^{s-1} dx$$

Finally, by inverse Mellin transform, for $\sigma \in (0,1)$ : $$f(x) - \frac{1}{x} = \frac{1}{2 i\pi}\int_{\sigma -i \infty}^{\sigma +i \infty} E(s) x^{-s}dx$$ and $\displaystyle f(1/y) - y = \frac{1}{2 i\pi} \int_{\sigma -i \infty}^{\sigma +i \infty} E(s) y^{s}dy$ as expected.

---

You can show $(2)$ converges for $Re(s) > 0$ by proving $\displaystyle\theta(x) = 1+ f(x) = \sum_{n =- \infty}^\infty e^{-\pi n^2 x^2}$ fulfills the functional equation $$\theta(1/x) = x\,\theta(x)$$ using the Poisson summation formula (see this proof).

So that $\displaystyle f(1/x) = \theta(1/x)-1= x \,\theta(x)-1 = x(f(x)+1)-1$ and : $$\int_0^1 \left(f(x)- \frac{1}{x}\right) x^{s-1} dx = \int_1^\infty (f(1/y) - y) y^{-s+1} \frac{dy}{y^2} $$ $$= \int_1^\infty (y (f(y)+1)-1-y) y^{-s-1} dy = \int_1^\infty (y f(y)-1) y^{-s-1} dy$$ whence $$E(s) - \frac{1}{s-1} = \int_0^1+\int_1^\infty \left(f(x)-\frac{1_{x<1}}{x}\right) x^{s-1}dx $$ $$= \int_1^\infty \left( f(x) (x^{s-1} + x^{-s}) - x^{-s-1}\right) dx$$ converges for $Re(s) > 0$.

Riemann obtained the functional equation $E(s) = E(1-s)$ from $$E(s) - \frac{1}{s-1} + \frac{1}{s} = \int_1^\infty f(x) (x^{s-1} + x^{-s}) dx$$ which is entire.