@Todd Trimble is correct; a strict fusion category is just a fusion category that is strict as a monoidal category. (Being strict or being fusion is just a property of a monoidal category that can either hold or fail.) In particular, the pentagon axiom holds, where the associators are identities on the nose. Moreover, there is a version of MacLane's theorem for pivotal fusion categories, see Section 2 of arXiv:0503167v2.
As someone who studies planar algebras, at one time I was very confused about this, and I think I know where the confusion lies. Let's focus on the fusion category $\operatorname{Vec}(G,\omega)$ where $\omega\in Z^3(G,\mathbb{C}^\times)$ is a cohomologically nontrivial 3-cocycle. The discussion below comes from a conversation I had with Scott Morrison which cleared up my confusion.
We will give two realizations of the fusion category $\operatorname{Vec}(G,\omega)$. The first has only one representative for each isomorphism class of simple objects. There are exactly $|G|$ many 1-dimensional simple objects denoted $V_g$ for $g\in G$, with fusion rules given by $V_g \otimes V_h = V_{gh}$, and associator given by
$$
(V_g\otimes V_h) \otimes V_k \xrightarrow{\omega_{g,h,k}} V_g\otimes (V_h\otimes V_k).
$$
The associators are easily seen to satisfy the pentagon axiom because of the 3-cocycle condition.
Now there's also a planar presentation of $\operatorname{Vec}(G,\omega)$. Usually, when we talk about a planar presentation, we're working with strict categories, because drawing strings next to each other in the plane is associative on the nose. (One can get around this by bending strings closer or further from other strings, or by drawing coupons for associators, but let's not do this here.)
The category $\operatorname{Vec}(G,\omega)$ has a planar presentation via oriented strands labelled by group elements (considering an oriented label the same as the reverse orientation with the inverse label), trivalent vertices
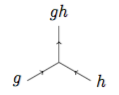
which correspond to a choice of isomorphism $g\otimes h \to gh$ for each $g,h \in G$, and relations
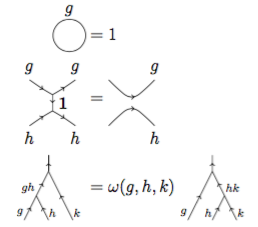
The 3-cocycle condition ensures the pentagon axiom holds for the trivalent vertices. (This is an important exercise to do!)
Since $\operatorname{Vec}(G,\omega)$ is pointed, there is only a one-dimensional space of maps $g\otimes h \to gh$, so choosing different isomorphisms for the trivalent vertices is equivalent to multiplying each trivalent vertex by a scalar $\mu(g,h)\in \mathbb{C}^\times$.
This results in changing the third relation above by a coboundary:
$$
\omega'(g,h,k) = \omega(g,h,k) \mu(g,hk)\mu(h,k)\mu(g,h)^{-1}\mu(gh,k)^{-1}.
$$
Notice that in the second description, there's not just one simple object in each isomorphism class. For example, $g\otimes h \neq gh$. One of these objects corresponds to two oriented strands labelled g and h, and the other corresponds to one oriented strand labelled gh. However, they are isomorphic. The associator is hidden in the trivalent vertices. If one forms a skeletal category that is equivalent to the second category by choosing one representative of each isomorphism class of simple objects, one would see a non-trivial associator reemerge in the pentagon axiom.
The moral is as follows. Your category can be strict, or it can have one simple object for each isomorphism class, but in general, it can't have both of these properties at the same time.
As for your application, even trying to categorify a fusion ring into a strict fusion category is extremely difficult. You can think of this as parallel to constructing non-trivial planar algebras, which is highly non-trivial...


