This is a variant of two as-yet unsolved MO questions cited below. Let $P$ be a closed polyhedron in $\mathbb{R}^3$. The task is to find a shortest path $\sigma$ on the surface of $P$ from which all the surface may be "inspected," which is interpreted as requiring that $\sigma$ touch every face of $P$. The path $\sigma$ might only touch a corner or edge of a face, but that suffices to inspect the face. For example, these seem straightforward:
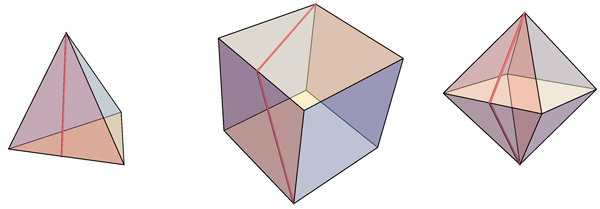
Optimal inspection paths on the tetrahedron, cube, octahedron.
I am less certain of the icosahedron. Update: Gerhard Paseman's $5$-edge path is shorter.

Left path length: $2+\sqrt{13} \approx 5.6$. Right: $5$.
I believe it is not difficult to show the problem is NP-hard (by reduction from TSP), so approximations are likely necessary. I have a sense, perhaps a vague memory, that this has been investigated in the literature, maybe only for restricted sets of convex polyhedra (e.g., the Platonic & Archimedean solids). But I have not been able to find any citations. If anyone can find a reference, or make a useful observation, it would be appreciated.
"Optimal inspection path on a sphere"
"Shortest closed curve to inspect a sphere"
