3
$\begingroup$

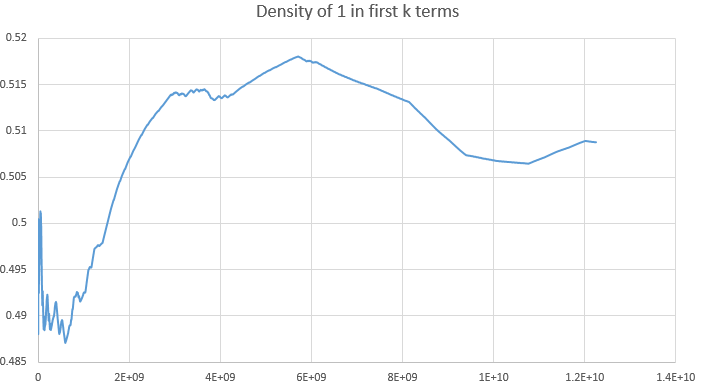
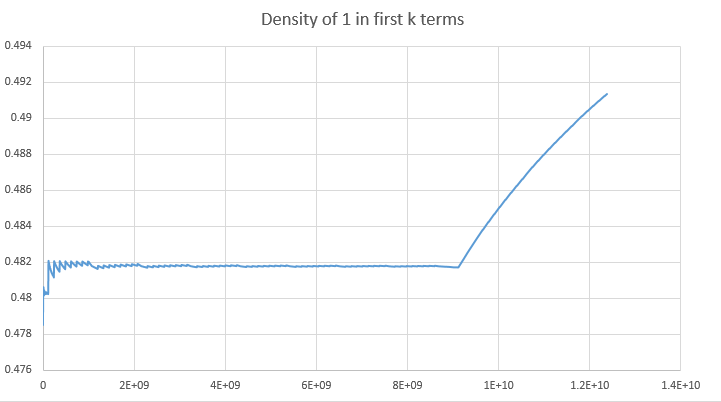
If we let $\operatorname{Kol}(a_1,\dots,a_n)$ be the run sequence determined by the rules of Kolakoski Frequencies, we ask is there a sequence of $\operatorname{Kol}$ that DOES NOT obey the $1/n$ frequency law?

For example $\operatorname{Kol}(1,2,3)$ starts $1,2,2,3,3,1,1,1,2,2,2,3,1,2,3,3,1,1,2,2,3,3,3\dots$, and we have $7\;1's, 8\;2's$ and $8\;3's$, and which is close to the distribution of $1/3:1/3:1/3$ predicted by the conjecture.,

We can prove $\operatorname{Kol}(2,4)$ does obey the law - the sequence begins $2,2,4,4,2,2,2,2,4,4,4,4,\dots$ and because all run-sequences are even, we have equality after end of a $4$-run.

It is a famous conjecture that all $\operatorname{Kol}$ sequences do have an equal distribution, and all that is needed is a single counter-example for a disprove, which doesn't seem to exist!

Links:

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ I'd prefer the question be self-contained. $\endgroup$
    – Gerry Myerson
    Commented Dec 28, 2015 at 15:07
  • 1
    $\begingroup$ You just might attract more interest in the question, Jon, if you made it more self-contained. Well, you could hardly attract less interest. $\endgroup$
    – Gerry Myerson
    Commented Jan 4, 2016 at 3:16

1 Answer 1

Reset to default
2
$\begingroup$

This is hardly a definitive answer, but $K(1,8)$ exhibits very odd non-convergent behavior in its first 12 billion terms.

Plot of K(1,8) frequencies

$K(1,16)$ also exhibits very weird behavior. It's even more climactic because it seems to settle into some pattern (of converging to a value that's not 1/2!) then boom, at 9 billion terms it shoots off like an ant.

Plot of K(1,16) frequencies

As you can see, this is good evidence of a counterexample, and also good evidence for how strange these sequences are.

Improve this answer
$\endgroup$
1
  • $\begingroup$ that's very good - i actually expected they'd all be the same! $\endgroup$
    – JMP
    Commented Feb 23, 2016 at 13:24

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .