2
$\begingroup$

Does there exist any complex Lie group $G$ such that there are some positive-dimensional compact almost complex submanifolds (for example, $\mathbb{C}P^m$) of $G$?

I want to get some examples.

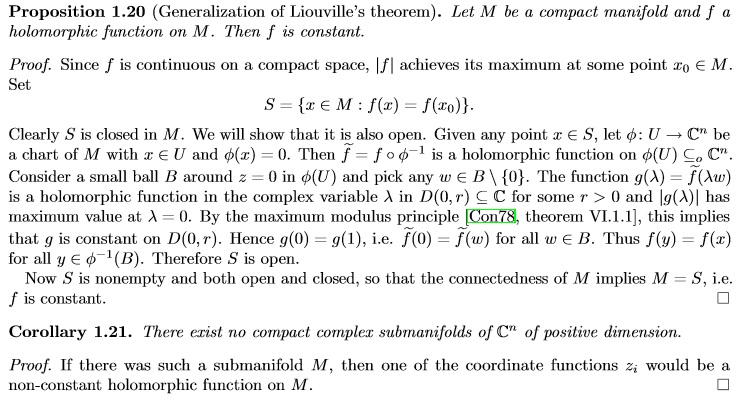
This is motivated by the following Corollary 1.21 from Complex Manifolds, Lecture Notes written by Clemens Koppensteiner (link)

Proposition 1.20 (Generalization of Liouville's theorem). Let $M$ be a compact [complex] manifold and $f$ a holomorphic function on $M$. Then $f$ is constant.

Corollary 1.21 There exist no compact complex submanifolds of $\mathbb{C}^n$ of positive dimension

Scan including the proofs:

enter image description here

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ It's best to cite the source of anything you quote or refer to. I was able to find it by google in this case caramdir.at/uploads/math/piii-cm/complex-manifolds.pdf $\endgroup$
    – j.c.
    Commented Sep 28, 2015 at 12:45
  • 2
    $\begingroup$ What do you mean by an "almost complex submanifold" of a complex manifold such as $G$? $\endgroup$
    – Jason Starr
    Commented Sep 28, 2015 at 12:45
  • $\begingroup$ It's hard to interpret the question in a nontrivial way (take any positive-dimensional compact complex Lie group $G$, then $G$ itself works...). Whether $\mathbb{P}^n$ embeds as a complex submanifold of a compact complex Lie group is possibly more reasonable (although I'm not a specialist, maybe the answer is obvious or well-known). $\endgroup$
    – YCor
    Commented Jan 23, 2017 at 21:08

2 Answers 2

Reset to default
1
$\begingroup$

Whatever almost complex submanifolds are, complex submanifolds should be a subclass. There are compact complex tori which admit complex sub-tori. One (natural) class of examples are given as follows: Consider a Riemann surface $\Sigma$ and its Jacobian $Jac,$ and a assume that there is a holomorphic double covering $\pi\colon \Sigma\to \Sigma'.$ wThen, by pulling back, you have a subtorus $Jac'\subset Jac$ and and also its $'complement'$ the Prym variety (of $\pi.$)

Another remark: you usually define complex hyper-surfaces as the zero locus of holomorphic sections of holomorphic line bundles (and not as the zero locus of holomorphic functions).

Improve this answer
$\endgroup$
2
$\begingroup$

Any almost complex submanifold of any complex manifold is a complex submanifold, as the Nijenhius tensor is the pullback of the Nijenhuis tensor. So the interesting problem is always in the other direction: when can we find complex submanifolds of an almost complex manifold? Any compact connected complex Lie group is abelian, because it has trivial image in any holomorphic representation (by Liouville's theorem), and so has trivial image in the adjoint representation, and so commutes with all elements near the identity (apply the exponential map) and so will all elements. But compact abelian complex Lie groups are complex tori. Their connected subgroups are also complex tori. These are rare: generic complex tori have no positive dimensional complex torus subgroups. Look at Birkenhake and Lange, Complex Tori for more information.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .