6
$\begingroup$

Asked at MSE here without response.

I realise that this resembles Odlyzko's famous nearest neighbours plot, and was wondering whether this is simply a manifestation of the same phenomenon.

That said, below is a partially scaled histogam plot of $\vartheta (\gamma_n) - \pi (n - 3/2) ,$ where $\gamma_n$ is the imaginary part of the $n$th zeta zero, and $\vartheta $ is the Riemann-Siegel theta function, for the first $2$m zeros:

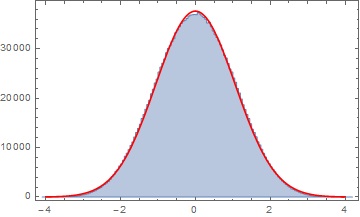
enter image description here

It certainly looks to have standard normal distribution, is this indeed the case? Does it depend on the RH, or is it independent of it?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
8
$\begingroup$

What you're observing is a remarkable theorem of Selberg. The usual notation is to let $N(T)$ denote the number of zeros of $\zeta(s)$ with ordinates between $0$ and $T$. Then the argument principle (with Stirling's formula) gives $$ N(T) = \frac{T}{2\pi} \log \frac{T}{2\pi e} + \frac{7}{8} + S(T) + O\Big(\frac 1T\Big), $$ where $S(T) = \frac {1}{\pi} \text{arg} \zeta(\tfrac 12+iT)$ (and the argument is defined by continuous variation along line segments from $2$ to $2+iT$ and then from $2+iT$ to $1/2+iT$). Then Selberg showed that $\pi S(T)=\text{arg}\zeta(\tfrac 12+iT)$ has a normal distribution with mean $\sim 0$ and variance $\sim \frac{1}2 \log \log T$. What you're looking at is the distribution of this remainder term in the asymptotic for the number of zeros when evaluated at the ordinates of the $n$-th zero, and from Selberg's work one can deduce that this is Gaussian. You'll find a discussion of Selberg's theorem in the books of Edwards or Titchmarsh.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ I think in your notation $N(\gamma_n)=n$ (by definition), and your $\theta(t)$ has the asymptotic expansion $\frac{t}{2}\log \frac{t}{2\pi e} -\frac{\pi}{8}$ etc. $\endgroup$
    – Lucia
    Commented Sep 23, 2015 at 23:34
  • $\begingroup$ Is this independent of the RH? I am presuming so ... $\endgroup$
    – martin
    Commented Sep 23, 2015 at 23:36
  • 1
    $\begingroup$ Yes, it's an unconditional result. $\endgroup$
    – Lucia
    Commented Sep 23, 2015 at 23:37
  • $\begingroup$ one last question - is it unrelated to GMT / GUE, etc? $\endgroup$
    – martin
    Commented Sep 23, 2015 at 23:57
  • 3
    $\begingroup$ Analogs of Selberg's theorem hold for the eigenvalues of random matrices. But the GUE conjectures give more precise local information on zeros -- eg information about how many zeros lie in an interval of length a fixed constant times 1/logT. Selberg's theorem is a bit coarser and gives information about typical intervals of length $\psi(T)/\log T$ say, provided $\psi(T) \to \infty$ with $T$. $\endgroup$
    – Lucia
    Commented Sep 24, 2015 at 0:14

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .