15
$\begingroup$

There is a theorem (I believe by Ocneanu) that the Markov trace on the tower of Temperley-Lieb algebras is (essentially) unique.

What about just traces on separate algebras? That is, take one of them, say $\mathbf{TL}_n$; what is the dimension of the space of linear functionals $\operatorname{tr}$ on it with the property $\operatorname{tr}(xy)=\operatorname{tr}(yx)$? (If one considers their versions with free parameters, i. e. as algebras over polynomials in parameters as free variables, then linearity is understood over these polynomials).

Equivalently, this is the question about the rank of the quotient of, say, $\mathbf{TL}_n$ by the subspace (resp. submodule over polynomials) spanned by all elements of the form $xy-yx$. If you want, about the rank of the 0th Hochschild homology (with itself as coefficients).

I could in fact restrict myself to the mother of them all - the group algebra of the braid group on $n$ strings. There, as Qiaochu Yuan says in a comment to the answer, there are as many independent traces as conjugacy classes in the group.

I've played with it a bit in lower dimensions, and got impression that the trace might be still essentially unique.

However as the answer below shows, this is certainly not so already for $\mathbf{TL}_4$ over $\mathbb C$.

Just for fun, here is the corresponding table of the (nontrivial cases of) "$xy=yx$" for $\mathbf{TL}_3$.

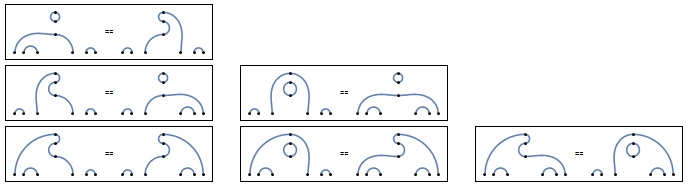
enter image description here

STILL LATER

Looked more carefully at the case of $\mathbf{TL}_4$; one sees that there are indeed three classes of basis elements with interdependent values of traces, which may be otherwise arbitrary. Representing the basis by four pairs of well-matched parentheses and denoting $k$ nested parentheses by $k$, these classes are

4
31, 22, 13, 211, 112, 1111
121, (12), (21), (11)1, 1(11), (111), ((11))
Improve this question
$\endgroup$
7
  • $\begingroup$ You mean the zeroth Hochschild homology group. $\endgroup$
    – Qiaochu Yuan
    Commented May 25, 2015 at 19:21
  • 2
    $\begingroup$ A finite-dimensional matrix algebra has a unique-up-to-scale trace, so your fourth paragraph is wrong. $\endgroup$
    – Theo Johnson-Freyd
    Commented May 25, 2015 at 22:55
  • $\begingroup$ @TheoJohnson-Freyd Although you are obviously right I must admit I am confused now. So for the time being I'll simply remove all mention of dimension two $\endgroup$
    – მამუკა ჯიბლაძე
    Commented May 26, 2015 at 14:00
  • $\begingroup$ Hint: linearity. $\endgroup$
    – Theo Johnson-Freyd
    Commented May 28, 2015 at 2:12
  • $\begingroup$ @TheoJohnson-Freyd Yes yes I thought about that, but without decomposing "my" algebras into product of matrix algebras (which is not available with the polynomial parameters I think) I cannot figure out how to invoke it. And even in the complex case there really is a separate one-dimensional summand, as the answer shows. $\endgroup$
    – მამუკა ჯიბლაძე
    Commented May 28, 2015 at 7:02

1 Answer 1

Reset to default
11
$\begingroup$

Let me work over $\mathbb{C}$ for simplicity. For generic values of the parameter the Temperley-Lieb algebra $TL_n$ is semisimple. A finite-dimensional semisimple algebra over $\mathbb{C}$ is a finite product $\prod M_{n_i}(\mathbb{C})$, and the zeroth Hochschild homology of such a product is $\prod \mathbb{C}$. So the dimension of the space of traces is the number of simple factors.

The number of simple factors is in turn the number of distinct simples occurring in the decomposition of the $n^{th}$ tensor power $V^{\otimes n}$ of the defining representation $V$ of $\mathfrak{sl}_2$ (or if you like, $U_q(\mathfrak{sl}_2)$, but this doesn't change the combinatorics), and there are $\left\lceil \frac{n+1}{2} \right\rceil$ of these. This is a straightforward induction on the identity

$$V \otimes S^n(V) \cong S^{n+1}(V) \oplus S^{n-1}(V).$$

The first case where this is bigger than $2$ is $n = 4$.

Improve this answer
$\endgroup$
7
  • 1
    $\begingroup$ @მამუკა ჯიბლაძე: I expect so, but I don't have a proof. And I mean $n = 4$: that's a ceiling, not a floor. $V^{\otimes 4}$ has three distinct simples in it, namely $1$, $S^2(V)$, and $S^4(V)$. $\endgroup$
    – Qiaochu Yuan
    Commented May 25, 2015 at 22:40
  • 1
    $\begingroup$ @მამუკა ჯიბლაძე: as for the group algebra, the zeroth Hochschild homology of a group algebra $\mathbb{C}[G]$ is the free vector space on the conjugacy classes of $G$. $\endgroup$
    – Qiaochu Yuan
    Commented May 25, 2015 at 22:41
  • 1
    $\begingroup$ @მამუკაჯიბლაძე and of course the "traces" are just the traces of the irreducible representations of $G$, which are equinumerous with the conjugacy classes. $\endgroup$
    – Will Sawin
    Commented May 26, 2015 at 14:53
  • 1
    $\begingroup$ @Will: that's fine as far as it goes if $G$ is finite, but the OP was specifically asking about the case that $G$ is a braid group. $\endgroup$
    – Qiaochu Yuan
    Commented May 26, 2015 at 17:16
  • 1
    $\begingroup$ @QiaochuYuan Good point. What I should have said is that traces of irreducible representations provide interesting explicit examples of traces on the group. $\endgroup$
    – Will Sawin
    Commented May 26, 2015 at 18:46

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .