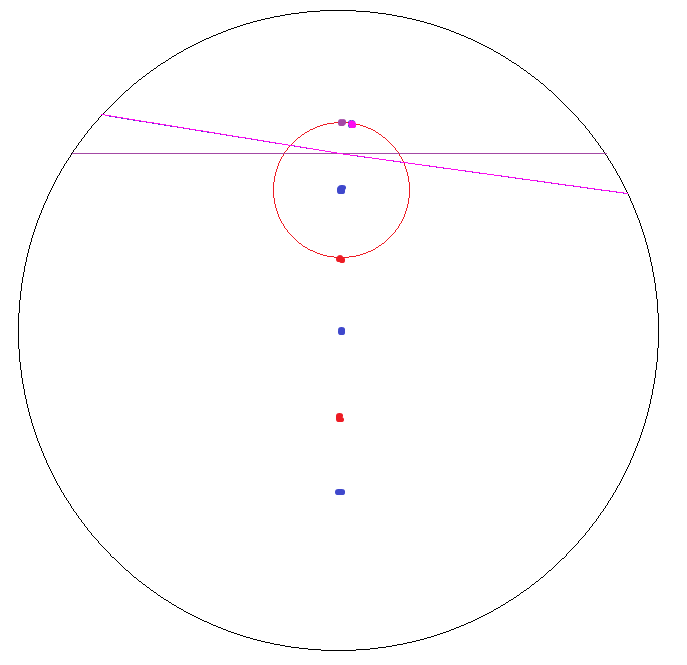
The board for this game is a compact convex region $\cal C$ of $\mathbb{R}^2$. Below I illustrate with $\cal C$ an equilateral triangle. Two players, $A$ and $B$, alternate turns. At each turn they add one point in $C$, not closer than $\epsilon > 0$ to any previously placed point. Label the points $a_1, b_2, a_3, b_4, a_5, \ldots$, with the letter indicating the player, and the subscript the turn number. The goal of the game is to control the most area. $A$'s area is the sum of the areas of the Voronoi regions of $a_1, a_3, a_5, \ldots$ within $\cal C$, and $B$'s area is similarly the areas of the Voronoi regions of of $b_2, b_4, b_6, \ldots$.
For example, below $A$ selects $a_1$ to be the centroid of $\cal C$, and $B$ selects $b_2$ to be $\epsilon$ below $a_1$. At this stage, $B$ is winning, owning about $\frac{5}{9}(\sqrt{3}/4) \approx 0.24$ while $A$ owns about $\frac{4}{9}(\sqrt{3}/4) \approx 0.19$. (Let us assume $\epsilon$ is very small.) It seems the next best step for $A$ is to grab most of $B$'s portion by placing $a_3$ just under $b_2$:
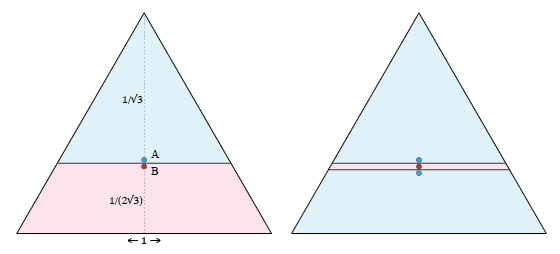
Q1. Does optimal (greedy at each step) play result in collinear points separated by $\epsilon$, for all $\cal C$? At least up until an accumulation of $\epsilon$s runs into boundary effects?
Q2. For any $\cal C$, is player $A$ ever ahead (under optimal play) after $B$ has just moved?