11
$\begingroup$

"A polygon is said to be rational if all its sides and diagonals are rational, and I. J. Schoenberg has posed the difficult question, ‘Can any given polygon be approximated as closely as we like by a rational polygon?’"

This is a quote from

D.D. Ang, D.E. Daykin, and T.K. Sheng. "On Schoenberg's rational polygon problem." Journal of the Australian Mathematical Society 9.3-4 (1969): 337-344. (journal link.)

I seek to understand the current status of this question. I know (from D.E.Daykin, "Rational polygons," (journal link)) that Mordell showed that the set of all rational quadrilaterals is dense in the set of all quadrilaterals. I am only finding ~40-yr-old papers, none of which are easily accessed online.

(Added:) As Aaron Meyerowitz and Gerry Myerson point out, it is unknown if there even exists a single rational octagon. But I am interested in the dense question. For example, is it already known that the set of all rational pentagons is not dense in the set of all pentagons? If not for pentagons, then for hexagons? Heptagons?

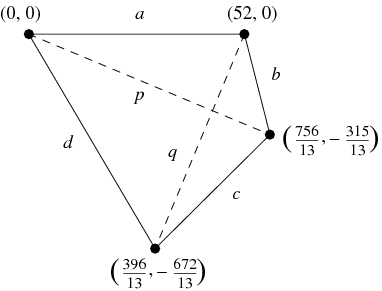
    MathWorld
    MathWorld image of a rational quadrilateral

Improve this question
$\endgroup$
5
  • 3
    $\begingroup$ Isn't it an open question if there can be a rational polygon with more than $8$ points? Well I suppose that is no $3$ on a circle, but certainly that would be an obstacle for an appropraitely drawn polygon. $\endgroup$
    – Aaron Meyerowitz
    Oct 12, 2014 at 2:37
  • 1
    $\begingroup$ Open even for 8, I think. 7 is the most anyone has found (I think). But Joseph knows that from earlier questions. $\endgroup$
    – Gerry Myerson
    Oct 12, 2014 at 6:34
  • $\begingroup$ Thanks Aaron & Gerry. My fault for not emphasizing the dense issue over mere existence. Now edited. $\endgroup$
    – Joseph O'Rourke
    Oct 12, 2014 at 12:38
  • $\begingroup$ It appears that this 1990 paper by A. Kemnitz, which I cannot access now (beyond the 1st two pages), might be relevant: "Integral Drawings of the Complete Graph K6." It appears that Kemnitz identifies an infinite class of rational hexagons, but perhaps not a dense set. $\endgroup$
    – Joseph O'Rourke
    Oct 12, 2014 at 23:53
  • 1
    $\begingroup$ @AaronMeyerowitz: yes, it is open, mathoverflow.net/a/100449/5340 gives a reference to a paper. $\endgroup$
    – Zsbán Ambrus
    Oct 31, 2014 at 14:49

1 Answer 1

Reset to default
2
$\begingroup$

This is an answer to your last question. As far as I know, it is still open whether there exists a dense subset $S$ of the plane with all pairwise distances rational. Such a set $S$ would imply a positive answer to Schoenberg's Problem. Thus, no one has currently shown that the set of rational pentagons is not dense in the set of all pentagons. Same for hexagons and heptagons.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Thank you, Tony. Remarkable that even the density question for pentagons remains open (although apparently solved by Mordell for quadrilaterals). $\endgroup$
    – Joseph O'Rourke
    Oct 12, 2014 at 23:14
  • $\begingroup$ You're welcome, Joseph. Note that it is not inconsistent with what I said that someone has proved that rational pentagons are dense (but I am unaware of such a result). If I had to bet a dollar, I would wager that it is false for pentagons. I think the consensus is also that the set $S$ does not exist, but these kinds of problems are notoriously difficult. $\endgroup$
    – Tony Huynh
    Oct 12, 2014 at 23:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.