15
$\begingroup$

I wonder if one can tile the plane with an order-$n$ L-polyomino in a fundamentally irregular manner. I seek help in defining what should constitute "irregular."

An L-polyomino of order $n \ge 2$ is a line of $n$ unit squares joined edge-to-edge, with one more square attached to the $n$-th to form an L-shape.

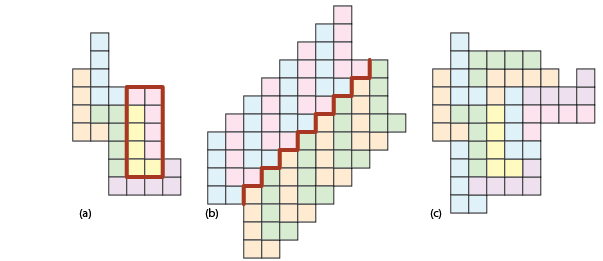
I am wondering for which $n$ is there an order-$n$ L-polyomino that can tile the plane "irregularly." Two regularities I would like to avoid are: (1) within the tiling any rectangle (of any size) tiled by L-polyominoes; see (a) in the figure below, using order-$4$ polyominoes. (2) any "periodic crack," which I define as an infinite staircase, with a finite periodic series of steps up/down and right/left, that never includes a point strictly interior to an L-polyomino. A simple example is in (b) below, but in general steps up and down, right and left, of varying length, would constitute a periodic crack if repeated infinitely. Such a periodic crack partitions the tiling into to "halves"; it is in some sense an infinite "digital line" cleaving the tiling in two halves.

Finally, (c) below shows a partial tiling by order-$4$ polyominoes which (so far) violates neither (1) nor (2).
Lpoly

Q1. Is there an irregular tiling by L-polyominoes under my definition of "irregular"?

Q2. Are there accepted definitions of what constitutes an irregular tiling, by one tile (a monohedral tiling)?

(Addendum 1). To respond to Zack's question, let us insist that the tiles can only be rotated—no mirror reflections permitted. (This may be not be standard notation...)

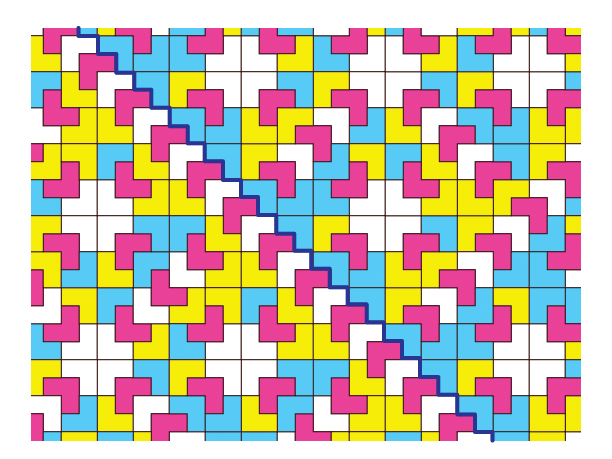
(Addendum 2). The chair tiling (from this link), as per Anthony Quas, with my superimposed periodic staircase:
Chair+Staircase

Improve this question
$\endgroup$
11
  • 1
    $\begingroup$ Do you allow mirror images of the tile? $\endgroup$
    – Zack Wolske
    Commented Jun 1, 2014 at 1:08
  • 1
    $\begingroup$ Of course if $n=2$, you have the `chair tiling'. There are interesting tilings of these. Non-periodic; no subrectangle is covered. I'm not sure if this counts as "regular" or not. $\endgroup$
    – Anthony Quas
    Commented Jun 1, 2014 at 1:22
  • 1
    $\begingroup$ There is a question that we always ask our students when they learn induction (to show them that it's not always a matter of proving that the sum up to $n$ of something is some given function of $n$). The example is showing that you can tile a $2^n\times 2^n$ grid with one $1\times 1$ square removed by "L"-shaped tiles (3 tiles arranged in an L shape). The tilings this proof gives are essentially the chair tiling. $\endgroup$
    – Anthony Quas
    Commented Jun 1, 2014 at 4:41
  • 2
    $\begingroup$ The chair tilings is the obvious example which is non-periodic. If you want a single aperiodic tile (that is, a tile which can tile the plane, but never periodically) then normally I would say you may be waiting a while - this is known as the monotile problem or einstein problem and remains open - math.stackexchange.com/a/533959/29059. However, L-polyominos do tile periodically so are not a solution. $\endgroup$
    – Dan Rust
    Commented Jun 1, 2014 at 9:17
  • 6
    $\begingroup$ The staircase in your chair tiling only happens because you chose a special point in the space of possible tilings. (These can be parameterized by quaternary sequences describing, for each level of deflation of the tiling, the position of the tile containing the origin in the next larger deflation...I think for aperiodic quaternary sequences you should avoid any infinite staircases.) $\endgroup$
    – David Eppstein
    Commented Jun 2, 2014 at 5:39

1 Answer 1

Reset to default
13
$\begingroup$

It is possible to not only avoid periodic monotonic staircases, but to avoid any infinite monotonic staircases whatsoever. This tiling of L-tetrominoes is periodic and features neither infinite monotonic staircases nor rectangles:

faultless tiling

I've highlighted copies of the fundamental region in different colours, so as to easily demonstrate the periodicity.

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ Beautiful, Adam! Thoroughly convincing. $\endgroup$
    – Joseph O'Rourke
    Commented Jun 8, 2014 at 1:42

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .