5
$\begingroup$

I would aim to know the complete classification of 6 dimensional non-semi simple Lie algebra (here the dimension stands for the generators; or the dimension $\leq 6$).

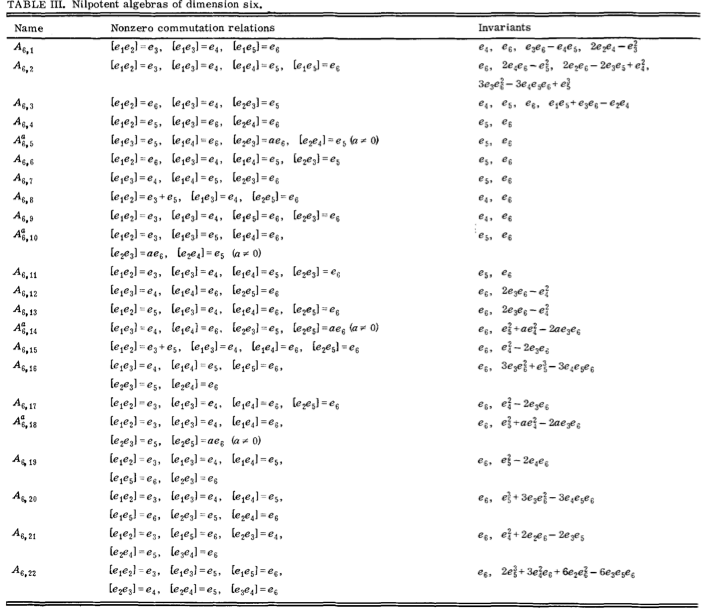
In this paper, in page 7, it stated that: "There is no complete classification of the six-dimensional real Lie algebras. However, all nilpotent six-dimensional Lie algebras are known." I also found this paper: J.Math.Phys. 17 (1976) 986, which lists nilpotent six-dimensional Lie algebras in Table III, p.991.

My question, again, is that: whether complete classification of 6 dimensional non-semi simple Lie algebra is known; for both real/complex non-semi simple Lie algebra of dimension 6? What is the most complete result? (In which paper/ref can I find a table of their Lie algebra?) Is that Table III a complete or incomplete for all real/complex non-semi simple Lie algebra of dimension 6?

Deep appreciation to whom concern and reply. (My question is motivated by a problem in topological field theory.)

enter image description here

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ The table is only for nilpotent Lie algebras. Is every six-dimenisonal Lie algebra nilpotent or semisimple? (That's a rhetorical question. The answer is clearly no, right?) $\endgroup$
    – Pete L. Clark
    Commented Dec 28, 2013 at 7:18
  • 1
    $\begingroup$ @cycles: the tag "central-simple algebras" is not relevant here (and probably neither is "representation theory"). $\endgroup$
    – YCor
    Commented Dec 28, 2013 at 12:33
  • $\begingroup$ This table is weird: several families depend on a parameter, but from several more modern treatments, it appears that there are finitely many 6-dimensional Lie algebras (over $\mathbf{R}$ or $\mathbf{C}$) (actually 30 over an arbitrary field of characteristic $\neq 2$, 34 over $\mathbf{R}$, see de Graff's paper: sciencedirect.com/science/article/pii/S0021869306005254, Journal of Algebra Volume 309, Issue 2, 15 March 2007, Pages 640–653) $\endgroup$
    – YCor
    Commented Dec 28, 2013 at 20:56
  • $\begingroup$ @Dear commenters: another refined question is posted by my colleague: mathoverflow.net/questions/153013/…; would you mind shed some light? Many thanks really. $\endgroup$
    – cycles
    Commented Dec 29, 2013 at 3:06
  • $\begingroup$ @Yves: In your last comment you want the word "nilpotent" somewhere, right? BTW I totally missed that some of the entries in the table have parameters! This makes my answer essentially worthless, so I have deleted it. However I amused by the fact (if it is a fact) that I was somehow actually right and there are only finitely many nilpotent Lie algebras. By the way: is it the case that there are only finitely many nilpotent Lie algebras of any fixed finite dimension (over $\mathbb{C}$, say)? $\endgroup$
    – Pete L. Clark
    Commented Dec 29, 2013 at 4:48

3 Answers 3

Reset to default
8
$\begingroup$

I am considering the complex case.

The list you wrote is complete for the NILPOTENT Lie algebras and was first obtained by Morozov in 1958 (paper in Russian). To obtain the calssification of ALL Lie algebras of dimension 6 one has to consider two more case:

  • non solvable

  • solvable but not nilpotent

As for the non solvable case there are only three possibilities:

1) ${\mathfrak sl}_2(\mathbb C)\oplus {\mathfrak sl}_2(\mathbb C)$

2) ${\mathfrak sl}_2(\mathbb C)\ltimes \mathbb C^3$ where the semidirect product is given by the adjoint action;

3) ${\mathfrak sl}_2(\mathbb C)\ltimes {\mathfrak n}_3(\mathbb C)$ where the semidirect product is by a sutable action on the Heisenberg -Lie algebra ${\mathfrak n}_3(\mathbb C)$ (3-dimensional, nilpotent).

Then you need to classify the solvable ones. This was achieved by Yamaguchi Mem. Fac. Sci. Kyushu Univ. Ser. A 35, pages 341-351 (1981). Some more informations are contained in a paper by Goze-Ancochea Bermudez (1985) where the variety of Lie algebras is studied as an algebraic variety for dimension up to 7. The relevant information connected to Clark's comment, is the number of irreducible components.

Improve this answer
$\endgroup$
11
  • 2
    $\begingroup$ There's one more non-solvable 6-dimensional Lie algebra: 4) $\mathbf{C}\times(\mathfrak{sl}_2(\mathbf{C})\ltimes\mathbf{C}^2)$. $\endgroup$
    – YCor
    Commented Dec 28, 2013 at 11:55
  • $\begingroup$ @Nicola Ciccoli, thanks for the reply. Please let me see. $\endgroup$
    – cycles
    Commented Dec 28, 2013 at 20:12
  • $\begingroup$ @Nicola and Yves, I am more interested in the real non-semi-simple Lie algebra which has non-degnerate invariant metric bilinear form $\Omega_{ab}$ (especially important if the Killing form degenerate). It is said that in 6 dimension the nilpotent one has only $A_{6,3}$ algebra admitting a non-degnerate invariant metric $\Omega_{ab}$. Also $\oplus^6 A_1$ and $A_{5,3}\oplus A_1$. Do you know more examples of 6 dim with non-degnerate invariant metric bilinear form $\Omega_{ab}$, please? $\endgroup$
    – cycles
    Commented Dec 28, 2013 at 20:37
  • $\begingroup$ Why not $\mathbb C^3 \times sl_2 (\mathbb C)$ $\endgroup$
    – Will Sawin
    Commented Dec 28, 2013 at 20:38
  • $\begingroup$ @Will: you're right: actually the missing are 4) $\mathbf{C}\times(\mathfrak{sl}_2(\mathbf{C})\ltimes\mathbf{C}^2)$; 5) $\mathbf{C}^3\times\mathfrak{sl}_2(\mathbf{C})$; 6) $\mathfrak{n}_3(\mathbf{C})\times\mathfrak{sl}_2(\mathbf{C})$, and unless I miss something, that's it. $\endgroup$
    – YCor
    Commented Dec 28, 2013 at 21:42
6
$\begingroup$

There are exactly four non-solvable complex Lie algebras of dimension $6$ with nontrivial Levi decomposition, namely \begin{align*} \mathfrak{sl}_2(\Bbb C) & \ltimes_{\phi} \Bbb C^3\\ \mathfrak{sl}_2(\Bbb C) & \ltimes_{\phi}\mathfrak{r}_{3,1}(\Bbb C) \cong \mathfrak{aff}(\Bbb C^2)\cong \mathfrak{gl}_2(\Bbb C)\ltimes \Bbb C^2\\ \mathfrak{sl}_2(\Bbb C) & \ltimes_{\phi} \mathfrak{n}_3(\Bbb C)\\ (\mathfrak{sl}_2(\Bbb C) &\ltimes_{\phi} \Bbb C^2))\oplus \Bbb C \cong \mathfrak{sl}_2(\Bbb C)\ltimes_{\psi}\Bbb C^3 \end{align*} The proof is straightforward, by computing all derivation algebras of $3$-dimensional Lie algebras $\mathfrak{r}$, and then considering Lie algebra homomorphisms $$ \phi\colon \mathfrak{sl}_2(\Bbb C)\rightarrow {\rm Der}(\mathfrak{r}). $$ The only derivation Lie algebras with are not solvable are the ones of $\Bbb C^3$, of the Heisenberg Lie algebra $\mathfrak{n}_3(\Bbb C)$, and of the solvable non-nilpotent Lie algebra $\mathfrak{r}_{3,1}(\Bbb C)$. Their derivation algebras are isomorphic to $\mathfrak{sl}_3(\Bbb C)\oplus \Bbb C$, $\mathfrak{aff}(\Bbb C^2)$ and $\mathfrak{aff}(\Bbb C^2)$.

I should add one reference, for the case of real numbers, namely the classification of all solvable real six-dimensional Lie algebras by Turkowsky:

P. Turkowski. Solvable Lie algebras of dimension six. J. Math. Phys. 31 (1990), 1344-1350.

As mentioned before, the classification of nilpotent Lie algebras of dimension $6$ has been done earlier by Morozow in $1958$, valid for all fields of characteristic zero. Recently this classification has been extended to arbitrary fields, by Serena Cicalo, Willem A de Graaf and Csaba Schneider here:http://arxiv.org/abs/1011.0361.

Improve this answer
$\endgroup$
5
  • $\begingroup$ an officemate/colleague of mine, she helps me to ask another relevant question here: mathoverflow.net/questions/152997; this is what we were looking for. Perhaps you could shed some light? Many thanks. $\endgroup$
    – cycles
    Commented Dec 28, 2013 at 22:03
  • $\begingroup$ @Dear Dietrich: another refined focused question is posted by my colleague: mathoverflow.net/questions/153013/…; would you mind shed some light? Many thanks really. $\endgroup$
    – cycles
    Commented Dec 29, 2013 at 3:07
  • $\begingroup$ @Dietrich, well Cicalo, almost sister let's say... $\endgroup$
    – Nicola Ciccoli
    Commented Dec 29, 2013 at 15:35
  • $\begingroup$ There's another one with nontrivial Levi decomposition: $(\mathfrak{sl}_2(\mathbb{C})\ltimes\mathbb{C}^2)\oplus\mathbb{C}$. In the real case, the list of forms is: to each of these four complex Lie algebras, its unique real analogue with split $\mathfrak{sl}_2(\mathbf{R})$, and in addition we only have $\mathfrak{so}_3(\mathbf{R})\ltimes\mathbf{R}^3$. $\endgroup$
    – YCor
    Commented Nov 9, 2022 at 14:59
  • $\begingroup$ @YCor Yes, it is also of the form $\mathfrak{sl}_2(\Bbb C)\ltimes_{\phi} \Bbb C^3$, but with $\phi=V(2)\oplus V(1)$ reducible, whereas the other one is with $\phi=V(3)$ irreducible. $\endgroup$
    – Dietrich Burde
    Commented Nov 9, 2022 at 15:24
3
$\begingroup$

A classification of d-dimensional Lie algebras for d < 7 appears in Appendix of the book

Classification and identification of Lie algebras L.Snobl and P.~Winternitz, vol. 33, CRM Monograph Series, 2014.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .