function that is the average of affine transformations of itself

Question

Consider the function $f : \mathbb{R} \to [-1,1]$ with $$ f(x) = \begin{cases} -1 & x \le -1 \\ +1 & x \ge +1 \\ \frac{f(\frac32 (x-\frac13)) + f(\frac32 (x+\frac13))}{2} & -1 \le x \le +1\,. \end{cases} $$ So $f$ is the average of two affine transformations of itself. The picture shows the graph of $f$ (in red) and the two affine transformations from the definition (in blue). 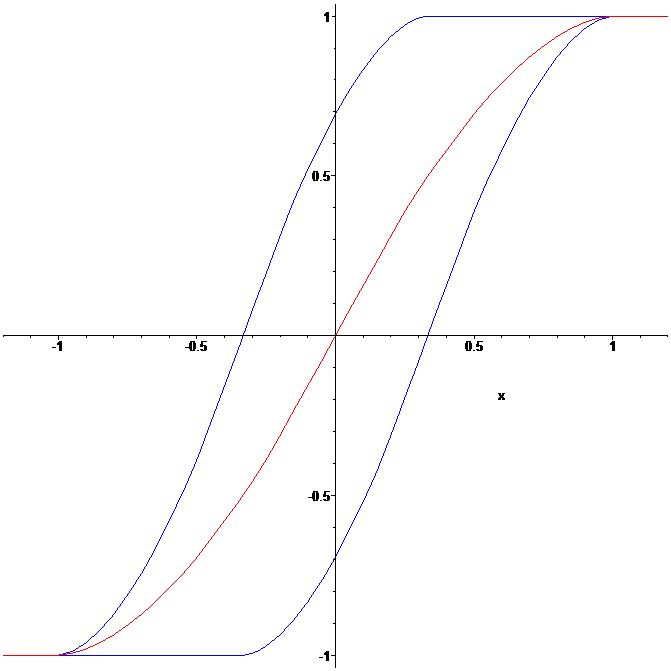What is known about such functions? Is $f(0.5) \approx 0.694064$ rational?

Answer 1

This function (with a parameter $0 < \lambda < 1$, more generally than $2/3$) arises in Probability as cumulative distribution function $f_\lambda$ of a sum $S:=\sum_{j=0}^\infty \pm \lambda^j$ with random signs, independently and identically chosen with equal probability $(1/2,1/2)$ (the $k$-th iterate considered here by Per Alexandersson corresponds to a partial sum, if I'm not wrong). In this context, a functional equation like the one you wrote is a simple consquence of the independence, partitioning into two cases, according to the sign of the first term.

For $\lambda <1/2$ one finds a function whose variation is concentrated on a Cantor like set -exactly the Cantor ternary set when $\lambda =1/3$. It is also easy to see that $\lambda=1/2$ corresponds to the uniform distribution $f(x) = x$ (due to elementary facts of the binary representation). What happens for $\lambda > 1/2$ is more difficult to understand; the problem is studied since the $30$s starting with Erdős, and found interesting connections in different areas of mathematics. I'm not adding other chats, but the keyword for a search, which is Bernoulli convolutions.

Here is a good survey paper on the subject: Sixty years of Bernoully convolutions, by Y.Peres W.Schlag, B.Solomyak .

Answer 2

We can define a sequence of functions converging to the one above:

$$f_1(x) = \begin{cases} -1 \text{ if } x<0 \\ 0 \text{ if } x=0 \\ 1 \text{ if } x>0 \end{cases}$$ and then $f_k(x) = \frac12[f_{k-1}(\frac23(x-\frac13)) + f_{k-1}(\frac23(x+\frac13)) ]$ for $k>1$.

Computing $f_k(1/2)$ for $k=1,...$ gives the sequence

$$ 1,\frac{1}{2},\frac{3}{4},\frac{5}{8},\frac{11}{16},\frac{23}{32},\frac{45}{64},\frac{89}{128},\frac{89}{128},\frac{89}{128},\frac{709}{1024}, \frac{1423}{2048},\frac{1421}{2048},\frac{5685}{8192},\frac{5687}{8192},\frac{22743}{32768},\frac{11371}{16384},\frac{2843}{4096}, \frac{181943}{262144},\frac{181947}{262144},\frac{727779}{1048576}$$

which seems to be alternating around some limit. No hits in OEIS on this, when multiplying with $2^k$.

It seems like looking at the base-2 and base-6 expansion would be helpful to analyze this problem, the expansions of the above numbers are relatively nice.