28
$\begingroup$

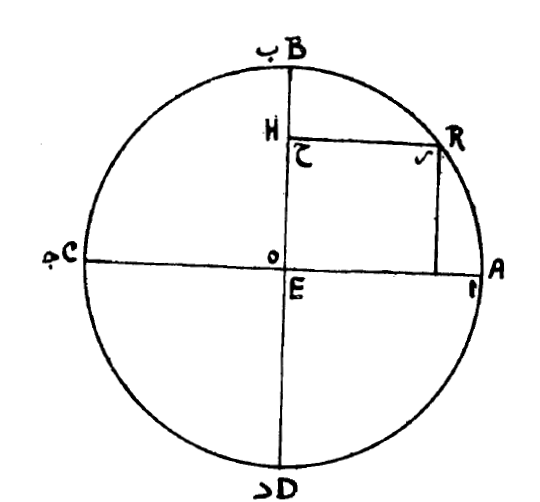
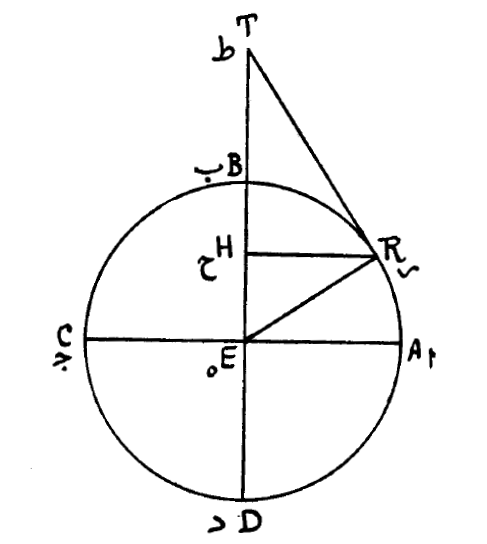
Evidently Omar Khayyam (1048-1131) was quite the mathematician. He did groundbreaking work on finding geometric solutions to the cubic equation, which is all the more notable since he did not have a good system of notation to work with.

As an example, suppose you want to solve $x^3 + 5x + 1 = 0$. Substituting $y = x^2$, one obtains $x(y + 5) + 1 = 0$, and so the solution lies at the intersection of a parabola and a hyperbola, which can be easily graphed.

More about his work can be found, e.g., here and here, and the ideas in his work also appear in modern papers such as this one of Wright and Yukie, and follow-up papers by Bhargava.

I was able to find this picture on Khayyam's Wikipedia page, which is possibly in Khayyam's own handwriting.

The first page of an untitled manuscript by Khayyam

I would love to use it in a talk. But what precisely does this picture represent? Judging from the sources I quoted (among others), it seems possibly related to the solution to $x^3 + 200x = 20x^2 + 2000$, but it is not clear exactly how.

Do any MO users read Arabic or otherwise know what this picture is?

Improve this question
$\endgroup$
4
  • $\begingroup$ I've asked someone from Syria who knows modern Arabic. He says it is a little challenging to read, that a ratio is involved, and that he might be able to say more tomorrow. My inference is that to compute the ratio of the two parts of the divided vertical radius in the top picture, a similar quantity involving the ratio of some areas in the bottom picture must be calculated. But I don't really know. $\endgroup$
    – The Masked Avenger
    Commented Sep 24, 2013 at 2:18
  • 1
    $\begingroup$ Brin's answer below seems to be the best reference, judging from MathSciNet reviews. The translation of Al-Khayyam into French by Rashed and Vahabzadeh seems to be a scholarly critical edition... $\endgroup$
    – Marty
    Commented Sep 26, 2013 at 7:32
  • 1
    $\begingroup$ The Stanford Encyclopedia of Philosophy discusses this document further: "Khayyam seems to have been attracted to cubic equations originally through his consideration of the following geometric problem: in a quadrant of a circle, drop a perpendicular from some point on the circumference to one of the radii so that the ratio of the perpendicular to the radius is equal to the ratio of the two parts of the radius on which the perpendicular falls. In a short, untitled treatise, Khayyam leads us from one case of this problem to the equation $x^3 + 200x = 20x^2 + 2000$... $\endgroup$
    – Marty
    Commented Sep 26, 2013 at 7:33
  • 1
    $\begingroup$ ...An approximation to the solution of this equation is not difficult to find, but Khayyam also generates a direct geometric solution: he uses the numbers in the equation to determine intersecting curves of two conic sections (a circle and a hyperbola), and demonstrates that the solution $x$ is equal to the length of a particular line segment in the diagram." (plato.stanford.edu/entries/umar-khayyam/#SolCubEqu) $\endgroup$
    – Marty
    Commented Sep 26, 2013 at 7:33

4 Answers 4

Reset to default
16
$\begingroup$

A gentleman named "Hadi Jorati" should be able to help you. He got his mathematics Ph.D. from Princeton and is getting a new Ph.D. on ancient texts of this sort from Yale.

Search his name (on google or facebook) and email him.

Improve this answer
$\endgroup$
8
$\begingroup$

There is this a translation in french of the algebra book by Omar AlKhayam. Maybe this could be helpful for your talk.

http://www.amazon.fr/Lalg%C3%A8bre-dOmar-Alkhayy%C3%A2m%C3%AE-accompagn%C3%A9e-manuscrits/dp/0543969797/ref=sr_1_26?ie=UTF8&qid=1380029771&sr=8-26&keywords=khayy%C3%A2m

Improve this answer
$\endgroup$
8
$\begingroup$

You could not relate the equation $x^3+200x=20x^2+2000$ to the figures because, in fact, it does not originate from them. Here, Khayyam tries to find a point on the circle such that $ \frac{AE}{RH} = \frac{EH}{HB} $.

enter image description here

The second figure represents his first attempt to solve the problem. enter image description here

He shows that points $E$ and $L$ lie on an equilateral hyperbola with asymptotes $TK$ and $TM$, but then he argues that there is not enough information to draw this hyperbola. Although this attempt fails, he includes it because it provides a good review of conic sections for learners!

Then, he changes his method and proposes a new solution. That new solution involves the following figure, and the equation you referred to comes from this approach. enter image description here

He first proves that we need $ER + RH = ET$, then as an example, takes $ER = 10$ ($RH$ being $x$) , and then does the algebra to arrive at the equation $x^3+200x=20x^2+2000$ (note that the trianlges $EHR$ and $ERT$ are similar.)

The importance of this essay lies in the fact that it was written before Khayyam's main work, as it is here that Khayyam mentions, if time permits, he will write an essay addressing all cases of cubic equations.

The figures and interpretation are courtesy of the following book in Persian, first published in 1960. enter image description here Gholamhossein Mosahab himself was one of the most influential mathematicians in Iran in the 20th century.

Improve this answer
$\endgroup$
7
$\begingroup$

These figures (second exact, first with an extra line) are discussed in two places (PP. 98-99 & 165-166) in "Omar Khayyam the Mathematician" ISBN 0-933273-46-0, by Rashed & Vahabzadeh, Bibliotheca Persica Press, NY. I have not tried to digest the discussion.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .