Looking for "large knot" examples

Question

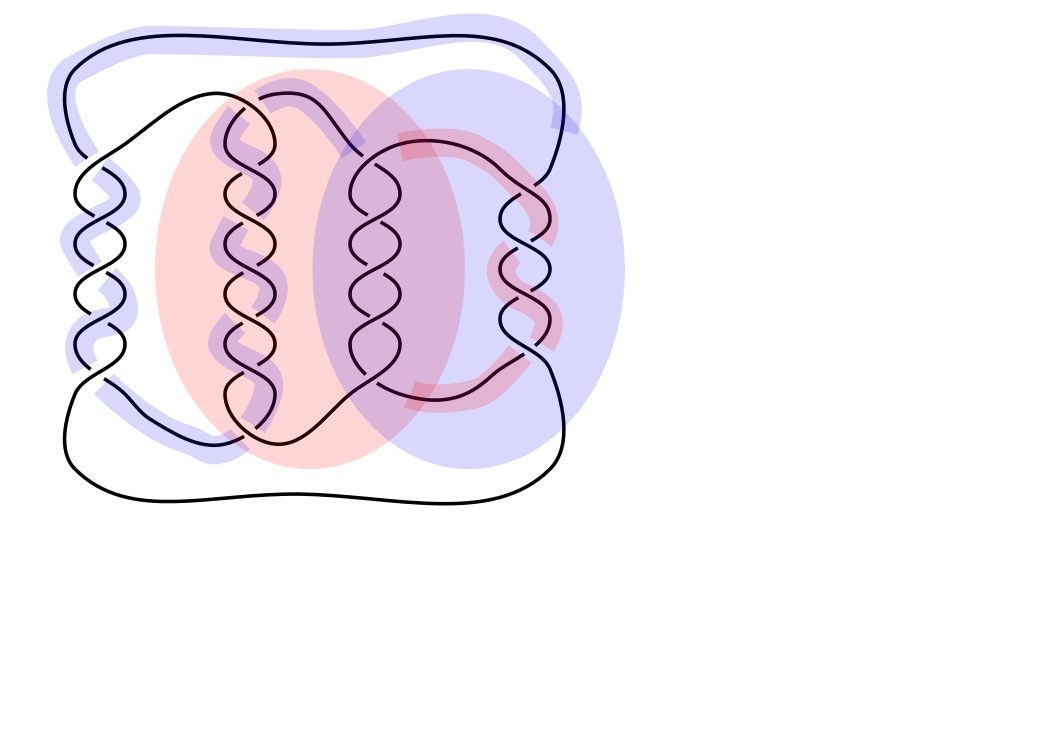
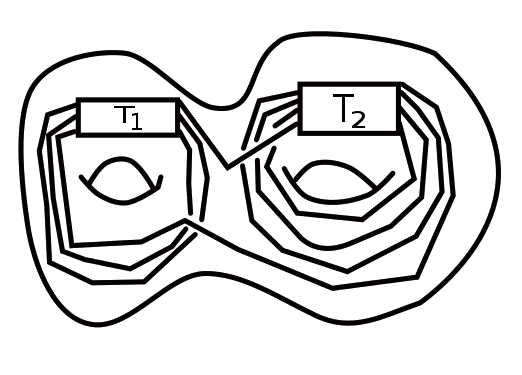
This question is about knots and links in the 3-sphere. I want to find an example of a "large" knot or link with some special properties. I'm looking for some fairly specific examples, but I'm also interested in related examples that don't precisely address my concern, so "near answers" are welcome.

Here is an example of something I would call a "large" knot.

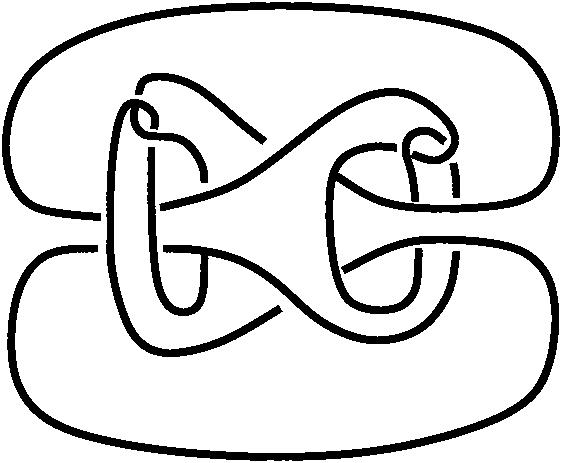
It is hyperbolic, but it also has an incompressible genus 2 surface in the knot complement. The genus 2 incompressible surface has a rather nice property, that it bounds a handlebody on one side (in $S^3$ rather than in the knot complement).

I'd like to find a similar example, but what I specifically want is a knot (or link) in $S^3$ which contains two genus 2 closed incompressible surface in the knot/link exterior, such that both surfaces bound handlebodies in $S^3$. The key thing I would like is for the two surfaces to intersect -- they can not be isotoped to be disjoint.

Is this possible? I presume it is, but I haven't seen examples of this type, and examples aren't rapidly coming to mind.

Other similar examples I'd like to see (although not "the" question here) would be knot or link exteriors that have infinitely many genus 2 closed incompressible surfaces -- preferrably bounding handlebodies on one side in $S^3$.

I'd also love to see a knot in $S^3$ with a genus 2 incompressible surface that does not bound a handlebody in $S^3$. Maybe this isn't possible?

Answer 1

As Ian Agol mentioned, manifolds with Conway spheres will have genus 2 incompressible surfaces. I'd just like to point out that an easy source of knots whose complements contain Conway spheres are pretzel knots, or more generally, Montesinos knots.

Here is a specific example of the pretzel knot $P(5, -7, 5, -4)$ with two incompressible Conway spheres in blue and red.

Form a pair of closed genus two surfaces by tubing along the arcs to join the boundary components pairwise on each Conway sphere. The resulting genus two surfaces each bound the handlebodies on the side indicated by the colors. (In the image, only one handle has been added to each Conway sphere.)

Answer 2

A couple of remarks:

Manifolds with Conway spheres will have genus 2 incompressible surfaces, by Theorem 2.0.3 of the proof of the cyclic surgery theorem. When there is a meridional planar incompressible surface (such as a Conway sphere), then one may tube the boundary components together in pairs to obtain a closed incompressible surface. I suspect that if one has a knot with several Conway spheres, then tubing carefully should yield genus 2 surfaces which intersect non-trivially.

If one has infinitely many genus 2 incompressible surfaces, then the knot must have an essential torus (this may be shown using normal surface theory/incompressible branched surfaces). For example, if one has a satellite knot with a Conway sphere which intersects the satellite torus essentially, then one probably has infinitely many genus 2 incompressible surfaces.

If one has a genus 2 surface in R^3, then it must be compressible to one side or the other (say to the inside; an incompressible surface in a knot complement must be incompressible to the outside). Compress the surface to get a torus. If that torus is incompressible to the inside (otherwise, it bounds a handlebody), then it must compress to the outside, and one has a ball-with-knotted-hole. To reconstruct the genus 2 surface from a ball-with-knotted-hole, add a tube to the outside. In order to make the outside incompressible, this tube must run through the knotted hole in some non-trivial way. In this way, one can manufacture a genus 2 surface which does not bound a handlebody, and is incompressible to one side. Put the knot on the inside so it busts the compressing disk to get the desired example.

Answer 3

This should be a partial construction. Embed $K$ as a knot in a handlebody such that the knot crosses the neck of the handlebody in two places as in the figure ($T_1$ and $T_2$ are meant to represent sufficiently complicated tangles). We may assume this embedding is hyperbolic by Myers' "Simple Knots in Compact, Orientable 3-Manifolds". Then by the methods of Adams and Reid in "Quasifuchsian surfaces in hyperbolic knot complements", the handlebody can be embedded in $S^3$ such that the exterior is incompressible.

The next paragraph is basically a sallow-follow construction. Cutting the handlebody along its neck we get two solid tori $H_1$ and $H_2$ with an arc (coming from the knot) embedded in each one of them. In the knot exterior the boundary of $H_i$ is a twice punctured torus and the annulus that bounds a neighborhood of the arc in it. Let $S_{1,2}$ be the union the twice punctured torus of $\partial H_1$ and the neighborhood of the arc in $H_2$ and let $S_{2,1}$ be the analog with the indices reversed. Then $S_{1,2}$ and $S_{2,1}$ should be incompressible genus 2 surfaces in a knot complement with the desired properties.