How to determine bounds on the extremal length around annuli?

Question

I wish to determine bounds for the sum of moduli of a family of topological annuli in the complex plane. Towards that end I would like to ask a question about the closely related concept of extremal length.

Let a quadrilateral $Q$ be a simply connected region of the complex plane, with Jordan curve boundary, and with two disjoint Jordan arcs $\gamma$ and $\gamma'$ making up part of the boundary. The two curves are each parameterised injectively by the closed unit interval, with the image $\gamma(t)$ (or $\gamma'(t)$) moving anticlockwise as $t$ increases. It is well known that for every quadrilateral there exists a unique $k \in \mathbb{R^+}$ and unique conformal homeomorphism $\phi$ mapping $Q$ onto the interior of a geometrical rectangle with vertices $0$, $1$, $1+ki$, and $ki$, and with $\gamma$ and $\gamma'$ mapped to the top and bottom edges respectively. In such a case the extremal length of curves joining $\gamma$ and $\gamma'$ in $Q$ is $k$. If the quadrilateral $Q$ satisfies the further conditions that $Re(\phi(\gamma(t))) = 1-t$ and $Re(\phi(\gamma'(t))) = t$ we say that $Q$ is a rectangle.

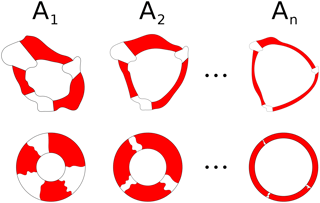
We construct a family of annuli by gluing together a collection of rectangles and generic quadrilaterals. For each $n$ let $(R^n_1, R^n_3, \ldots, R^n_{2m-1})$ be an m-tuple of rectangles, with extremal lengths $k_1n, k_3n, \ldots, k_{2m-1}n$ respectively, where each $k_i$ is a positive scalar fixed for all $n$. Likewise, let $S_2, S_4, \ldots, S_{2m}$ be a sequence of generic quadrilaterals. For each $n$, we form an annulus by taking the first element $R^n_1$ in our sequence of rectangles and identifying the edge $\gamma'_1$ of $R^n_1$ with the edge $\gamma_2$ of $S_2$, identifying $\gamma'_1(t)$ to $\gamma_2(t-1)$, for each $t$. We then identify the edge $\gamma'_2$ of $S_2$ with the edge $\gamma_3$ of $R_3$, again identifying $\gamma'_2(t)$ to $\gamma_3(t-1)$, and so on, finally identifying the edge $\gamma'_{2m}$ of $S_{2m}$ with $\gamma_1$ of $R^n_1$.

Intuitively, it seems to me, as $n$ increases one should expect the sections of the annuli made up of rectangles to predominate over those sections made up of generic quadrilaterals. See the figure below, where the red shaded regions represent images of the rectangles. Thus for $n$ large one would expect the extremal length around the annuli $A_n$ to closely approximate the sum $(k_1 + k_3 + \ldots + k_{2m-1})n$. Could someone confirm that this is indeed the case, and if it is the case suggest a reference or some suggestions as to how one might go about proving it?

     (source)

Answer 1

This is a comment, but it is a bit too long, so I will post it as an answer. At best, this is the restatement of the obvious proof strategy.

Let $A$ be an annulus, and let $\Gamma_1$ be the family of curves homotopic to the generator of the fundamental group of the annulus.
From the definition,

$$Mod(A)^{-1} = {\rm Ext}(\Gamma_1) = \sup_{\rho} \inf_{\gamma \in \Gamma_1} \frac{\ell_\rho(\gamma)^2}{{\rm Area}_\rho(A)}$$,

where the supremum is over the metrics in the conformal class of $A$. This means that if you do the calculation for a specific metric $\rho$, you get a lower bound for ${\rm Ext}(\Gamma_1)$ which gives you an upper bound for ${\rm Mod(A)}$. The picture you draw is suggestive for the possible choices of $\rho$.

To get lower bounds, one way is to use the identity

$$Mod(A) = {\rm Ext}(\Gamma_2) = \sup_{\rho} \inf_{\gamma \in \Gamma_2} \frac{\ell_\rho(\gamma)^2}{{\rm Area}_\rho(A)}$$,

where $\Gamma_2$ is the family of curves connecting the boundaries of the annulus. Now doing the calculation for a specific $\rho$ gives you a lower bound for ${\rm Mod(A)}$.

Perhaps by choosing the metrics to some interpolated versions of the flat metrics on the rectangles you can get reasonable upper and lower bounds.