We are given a disk $D$ in $\mathbb{R}^2$. Let $C$ be its boundary (i.e., the circle bounding $D$ on its plane). Let $P(n,d)$ be a set of $n$ pairs of chords of $C$ such that for each $\{c,c'\}\in P(n,d)$, $c$ and $c'$ are parallel and the distance between them is equal to $0<d<r$, where $r$ is the length of the radius of $C$.
Finally, let $R$ be the set of all connected regions of $D$ obtained by cutting $D$ by all pairs of chords of $P(n,d)$, in such a way that each region of $A\in R$
- does not contain any point belonging to any of such chords,
- is bounded by some of these chords or $C$, and
- there exists a pair of chords $\{c,c'\}\in P(n,d)$ such that all points of $A$ are located between $c$ and $c'$.
Question: What is the maximum number $m(n,d)$ of regions in $R$ over all possible set of $P(n,d)$?
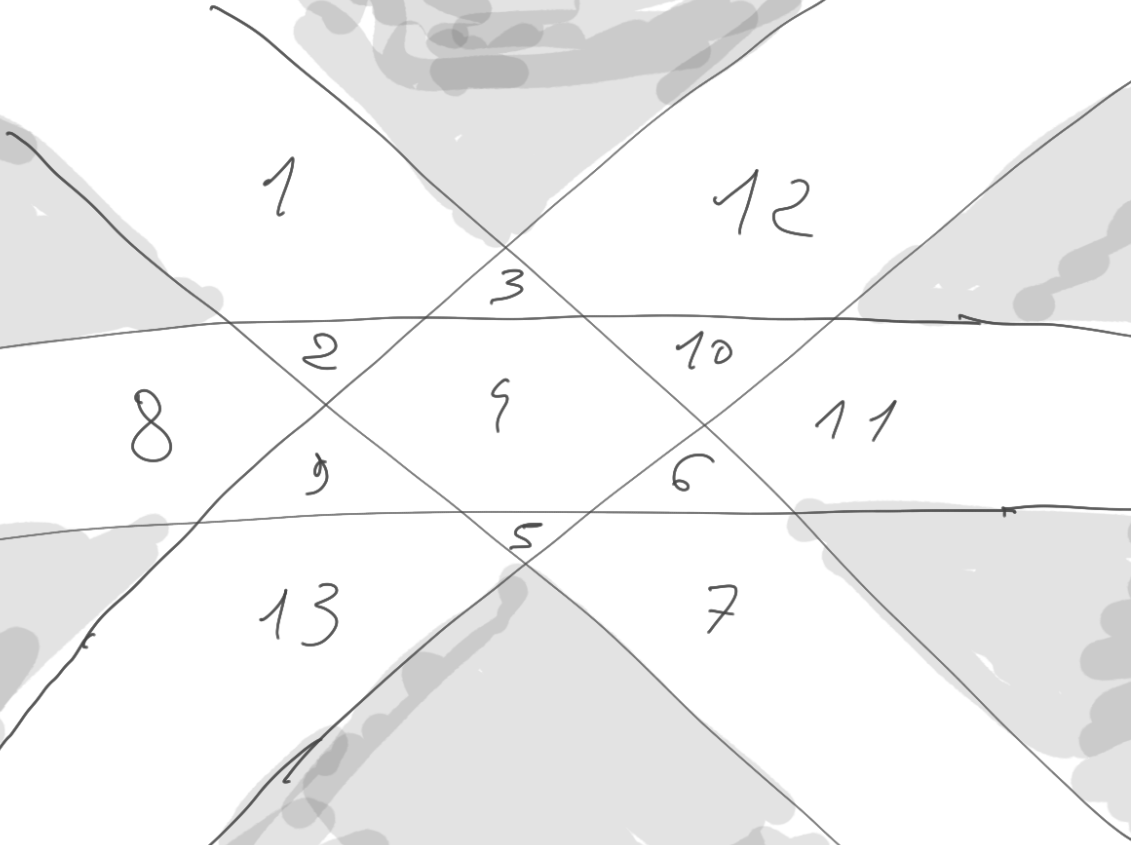 Example: For all $d$, we have $m(1,d)=1$, $m(2,d)=5$, $m(3,d)=13$ - In fact, I actually conjecture that $m$ depends on $n$ solely.
Example: For all $d$, we have $m(1,d)=1$, $m(2,d)=5$, $m(3,d)=13$ - In fact, I actually conjecture that $m$ depends on $n$ solely.
