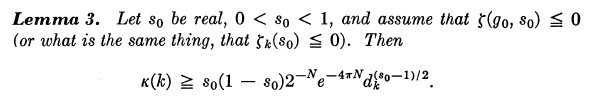3
$\begingroup$

I was reading through chapter 16 of Lang's Algebraic Number Theory book. The chapter is fully devoted to proving the Brauer-Siegel theorem: Let ${(k_n/ \mathbb{Q})}_n$ be a sequence of galois extensions over $\mathbb{Q}$ of degree $N_n$, such that $\frac{N_n}{log(d_n)} \to 0$ then $log(h_n R_n) \sim \frac{1}{2}log(d_n)$, where $d_n, h_n, R_n$ are the absolute value of the disciminant, class number and regulator of $k_n$ respectively. The following is one of the main theorems in the chapter.

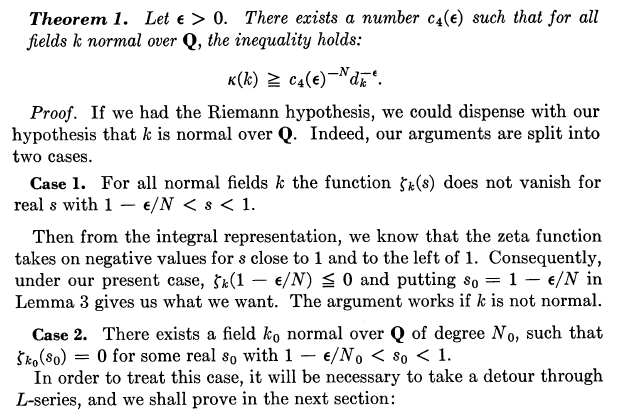
enter image description here

As said in the proof, the theorem easily follows if RH (or rather GRH) is assumed from a previous lemma which is as follows,

enter image description here

As given in the lemma it is only required to assume that the Dedekind zeta function $\zeta_k(s_0) \leq 0$. He treats case 2, separately going through several other lemmas.

Now in case 2 picking $s_0 \in (1 - \frac{\epsilon}{N}, 1)$ a zero of $\zeta_k(s)$ we would still have that $\zeta_k(s_0) \leq 0$ and the result immediately follows from the lemma as in case 1. So why do we have to treat it separately?

Edit:

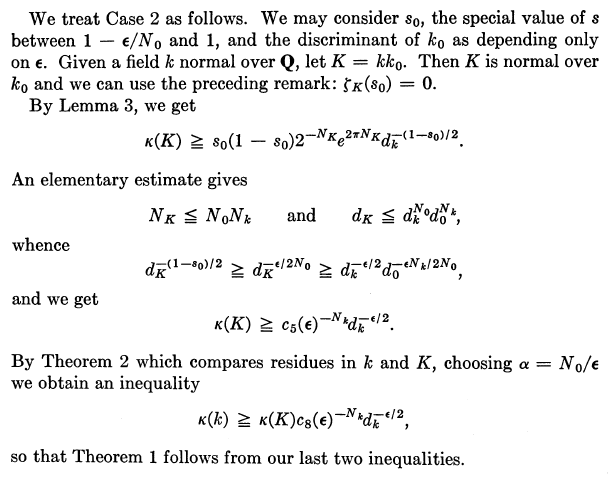
Going through the separate treatment of case 2 shown below, I understand that using the fact that $\zeta_{k_0}(s_0) = 0$, the theorem is proven for other number fields that may have the same pathological behaviour. Let me know if this line of thought is correct. Well, then Theorem 1 should have been "... for all fields normal over $\mathbb{Q}$ except for one possible exception..." since the theorem is left unproven for $k_0$.

enter image description here

Edit: Here $\kappa(k) = \frac{2^{r_1}{(2\pi)}^{r_2}h_k R_k}{\omega {d_k}^{\frac{1}{2}}}$ is the residue of $\zeta_k(s)$ at $s = 1$.

Improve this question
$\endgroup$
7
  • 1
    $\begingroup$ (Because the constant in the inequality, which is supposed to hold for all $k$, will depend on $k_0$! In other words either none of them vanish in the interval, in which case you’re good, or one of them does, in which case you get an inequality that works for every single one of the fields just in terms of that one zero.) $\endgroup$
    – alpoge
    Commented Feb 9, 2021 at 22:54
  • $\begingroup$ @apolge Thanks for the explanation. But why cannot we just forget about this pathological $k_0$ and work with the rest? $\endgroup$
    – Melanka
    Commented Feb 10, 2021 at 13:51
  • $\begingroup$ I will also edit the post to include the resolution of case 2, in which I have some other clarifications. $\endgroup$
    – Melanka
    Commented Feb 10, 2021 at 13:56
  • $\begingroup$ What is $\kappa(k)$, the distance between $1$ and the nearest zero of $\zeta_K(s)$, or something like that? $\endgroup$
    – reuns
    Commented Feb 10, 2021 at 14:29
  • 1
    $\begingroup$ "Well, then Theorem 1 should have been '... for all fields normal over $\mathbb{Q}$ except for one possible exception...'" This is only necessary if you want $c_4(\epsilon)$ to be effective. If one does not care about that (Brauer-Siegel doesn't), then you can allow $c_4(\epsilon)$ to depend ineffectively on $k_0$. This is meaningful in many contexts, but not all. You can't simply "forget about this pathological $k_0$ and work with the rest" unless you are ok with the uncertainty of "am I working with $k_0$?" If $c_4(\epsilon)$ is ineffective, then it doesn't matter if $k=k_0$ or not. $\endgroup$
    – 2734364041
    Commented Feb 10, 2021 at 20:46

0

Reset to default

You must log in to answer this question.