I am reading a paper called A NOTE ON THE RADICAL OF A MODULE CATEGORY by
CLAUDIA CHAIO AND SHIPING LIU. This is Theorem 2.7: 
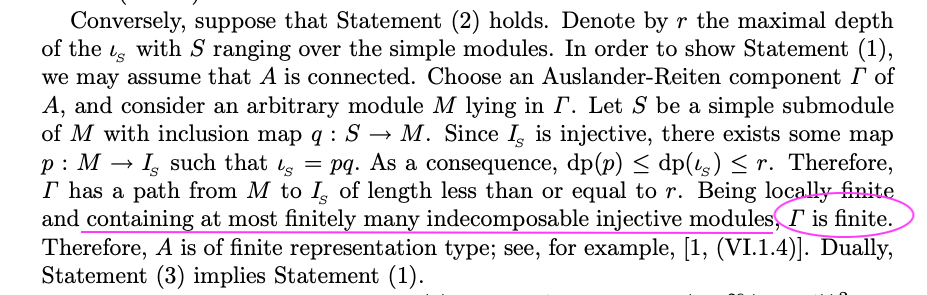
And this is part of it's proof, in which the direction (2) $\Rightarrow $ (1) is shown.
$\iota_S: S \rightarrow I(S)$ denotes the injective envelope and $\pi_S: P(S) \rightarrow S$ denotes the projective cover of a simple module $S$. Also dp$(f)$ denotes the depth of $f$. As far as I know a quiver is locally finite means iff between each two vertices there is only a finite number of arrows between them
I really don't understand why it follows that the Auslander-Reiten component $\Gamma$ is finite. Also why does $\Gamma$ contain at most finitely many indecomposable injective modules?
Can anybody help with his? Any help is highly appreciated!