3
$\begingroup$

Let $S$ be a commutative unital Noetherian ring. Let $D^+(S)$ be the derived category of bounded-below cochain complexes. Given $M^\bullet\in D^+(S)$, define $$\operatorname{Ass}(M^\bullet):= \bigcup_i \operatorname{Ass}(H^i(M^\bullet)).$$ Consider the following (incorrect) statement:

$(\ast)$ Let $M'^\bullet\to M^\bullet \to M''^\bullet \xrightarrow{+1} $ be a distinguished triangle in $D^+(S)$. Then $$\operatorname{Ass}(M^\bullet) \subseteq \operatorname{Ass}(M'^\bullet)\cup \operatorname{Ass}(M''^\bullet).$$

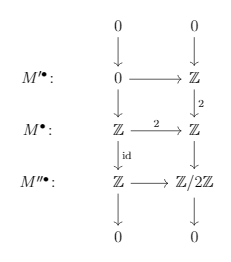
A counterexample to $(*)$ is the following short exact sequences of complexes:

enter image description here

In this situation, $\operatorname{Ass}(M^\bullet)=\operatorname{Ass}(\Bbb Z/2\Bbb Z) = \{(2)\}$, while the other only associated prime of the other two complexes is $(0)$.

My question

Is there a "commonly occurring" (take that to mean what you will) situation in which $(*)$ or something similar does hold?

Improve this question
$\endgroup$
3
  • $\begingroup$ This does not answer your question, but I feel it is worth pointing out that your definition of associated primes for complexes is "wrong". For a correct definition, see mscand.dk/article/view/14336/12333 $\endgroup$
    – the L
    Commented Oct 22, 2020 at 11:28
  • 1
    $\begingroup$ By the way, you can make the counterexample even simpler (and a bit more transparent as to what goes wrong): The short exact sequence $0 \rightarrow 2\mathbb{Z} \rightarrow \mathbb{Z} \rightarrow \mathbb{Z}/2 \rightarrow 0$ sitting as complexes in degree $0$ gives a d.t., but by rotating the triangle you can get the $\mathbb{Z}/2$ into the middle. $\endgroup$
    – Pavel Čoupek
    Commented Oct 22, 2020 at 16:25
  • $\begingroup$ So the issue is that cokernel "may produce new associated points". If you replaced "$\mathrm{Ass}$" with "$\mathrm{Supp}$" everywhere, the analogue of $(*)$ should be true. This is probably not close enough to $(*)$, though. $\endgroup$
    – Pavel Čoupek
    Commented Oct 22, 2020 at 18:16

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .