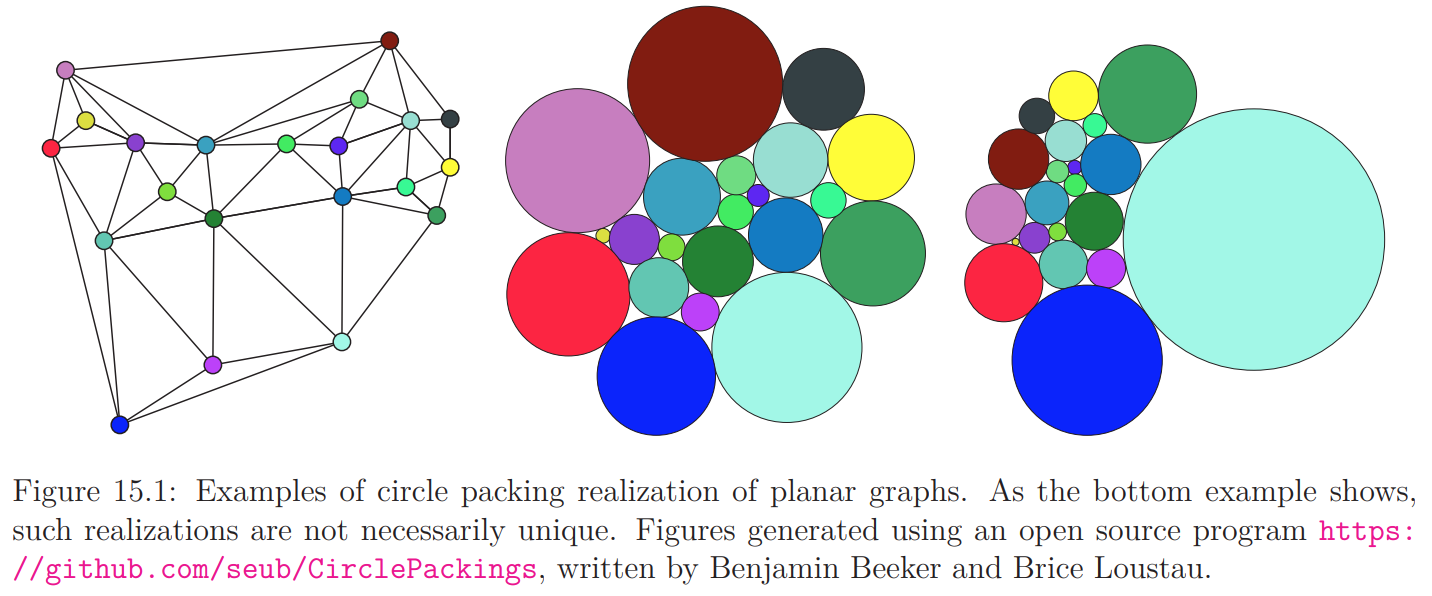
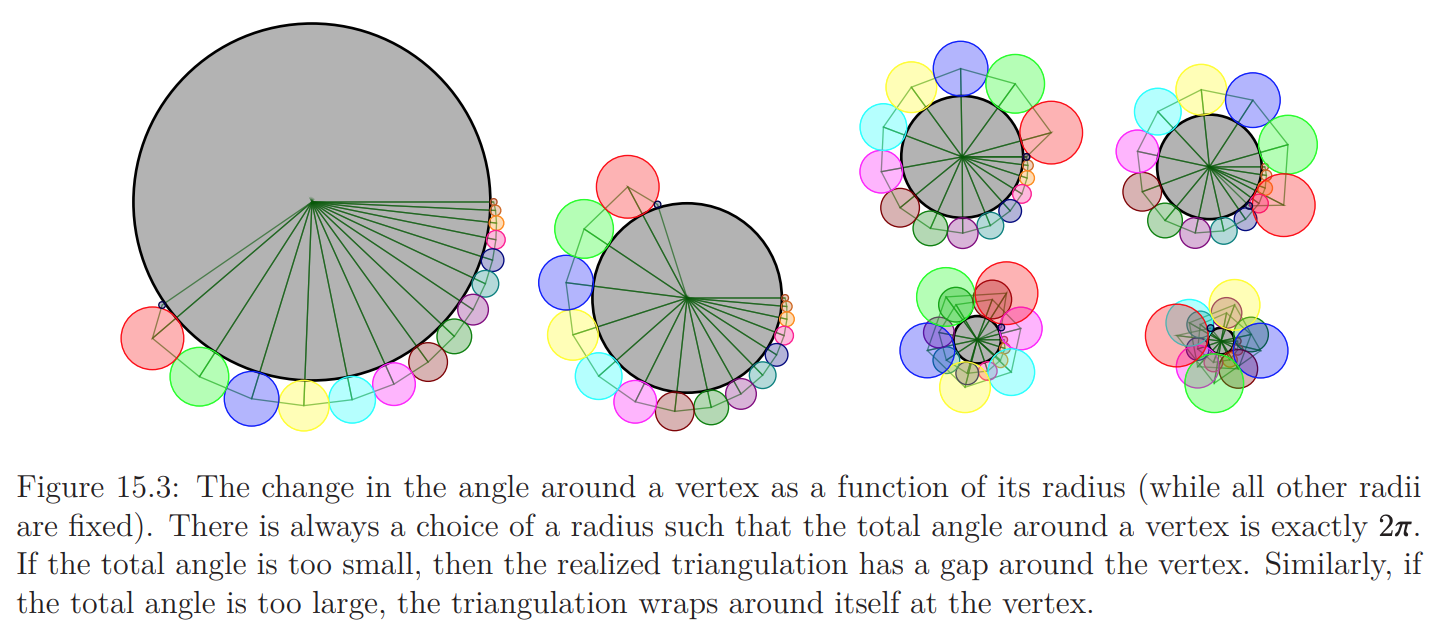
Circle packing theorem is a famous result stating that for every connected simple planar graph $G$ there is a circle packing in the plane whose intersection graph is $G$ https://en.wikipedia.org/wiki/Circle_packing_theorem.
I know that this result has many proofs and I want to read one of them, but don't understand how to start (for quite a while). The article in wiki gives a reference to Thurston notes, but the proof comes only in the last section and I am not sure if this is the simplest approach. I like these notes very much, but was never able to read them till the end. So I wonder if there are some simple proofs of this result nowadays. Can you advise something?