Can projection of convex sets onto convex sets be non-convex yet connected? If so is there any necessary and sufficient conditions?
Can projection of $n$ dimensional convex sets in $\mathbb R^{n'}$ onto $m$ dimensional convex sets in $\mathbb R^{m'}$ with $m<m'<n<n'$ produce non-convex connected sets of dimension smaller than $m$?
$\begingroup$
$\endgroup$
4
-
3$\begingroup$ What happens if you project a line onto a ball? It's not convex right? Or a full cylinder onto a well-aligned circle for your question 2? $\endgroup$– Najib IdrissiCommented Aug 25, 2020 at 15:24
-
3$\begingroup$ The question is not clear. What do you mean by a projection? $\endgroup$– Piotr HajlaszCommented Aug 25, 2020 at 17:38
-
$\begingroup$ Clarify your question and I will try to reopen it, or delete it if you don't really know what the question is about. $\endgroup$– Piotr HajlaszCommented Nov 23, 2022 at 4:57
-
$\begingroup$ @PiotrHajlasz I do not remember what the context of the question was about. Anyway I have accepted an answer then. $\endgroup$– TurboCommented Nov 23, 2022 at 16:11
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
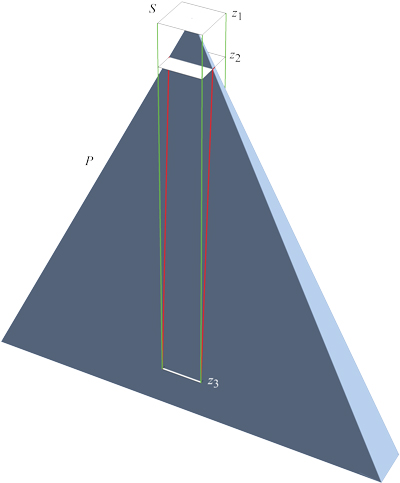
I believe this answers (1). $P$ is the pyramid illustrated. $S$ is a square resting on the apex of $P$, at height $z_1$. Projecting $S$ down (green lines) onto $P$ results in the nonconvex shape outlined in red. The projection only reaches $z_2$ on the thin side faces, but much further down to $z_3$ on the front and back faces. The front/back faces are slanted more steeply than the left/right faces. So, Yes: Projection of a convex set $S$ onto a convex set $P$ can be nonconvex and connected.