Uniform closure of a neighbourhood complex in the tritetragonal tiling

Question

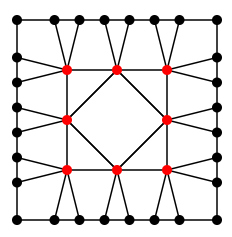
Consider a neighbourhood complex of eight vertices (red) with vertex configuration $(3.4)^3$ which gives rise to the tritetragonal tiling of the hyperbolic plane:

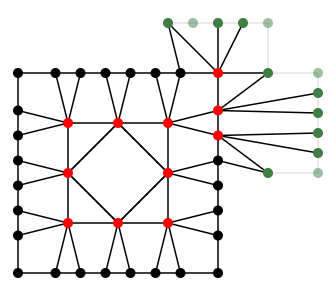
Not knowing if this complex can be uniformly closed – by adding edges such that all vertices in the resulting graph have vertex configuration $(3.4)^3$ – one might start trying to do so. Start "complementing" the first three vertices (from the top right one down). First create the missing faces virtually:

You end up with a sequence of connected virtual vertices which now have to be identified with a free sequence on the border of the complex. ("Free" means that each vertex in the sequence on the border must have degree $d \leq 6-k$ where $k$ is the number of new edges attached to the virtual vertex (black) – except for the dummy vertices (pale green).)

You can continue this process – complementing one vertex with $d < 6$ after the other and identifying the sequence of new virtual vertices with a free sequence – but most probably you will get stuck and not find a free sequence before you are done.

What does this mean?

1. Will this procedure never be successful for this specific neighbourhood complex?

2. If it can be successful: How do I have to choose a correct free sequence in each step? (There may be several.)

3. If it can not be successful: Are there other neighbourhood complexes for which it is?

---

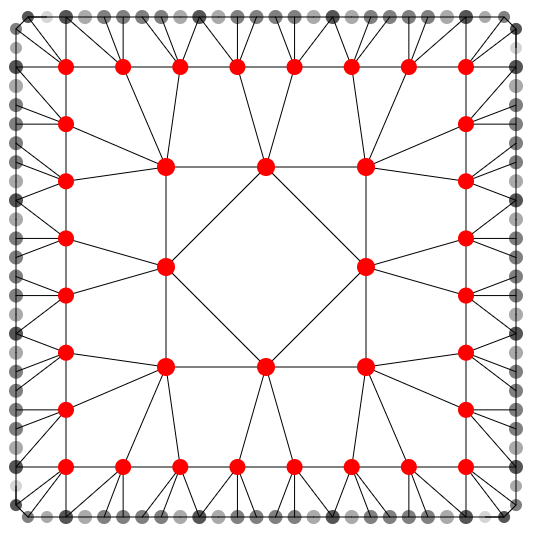
Edit: This is a slightly larger neighbourhood complex containing $36$ vertices with configuration $(3.4)^3$ (exactly the ones in the original complex above).

Answer 1

Just some thoughts.

Suppose you can close a complex $(4.3)^3$ somehow to a finite complex with, say, $v$ vertices, $e$ edges, and $f=f_3+f_4$ faces ($f_3$ triangles and $f_4$ quadrangles), then by double counting

$$3 f_3 = 3 v \implies f_3= v,$$ $$4 f_4 = 3v \implies f_4=3v/4.$$

Also, every vertex has degree six, so $e=3v$. If we assume that this complex has Euler characteristic $\chi$, then by Euler's formula

$$\chi = v-e+f=v(1-3+1+3/4) = -v/4\quad\implies\quad v=-4\chi.$$

So the characteric must be negative and $v$ must be a multiple of $4$. Similarly, we find $f=-7\chi$ and $e=-12\chi$.

For example, if you want to close this complex to a double torus, then $\chi=-2$, $v=8$ and $f=14$. These numbers feel too small. So we need a larger genus (more holes, smaller characteristic). But the vertex count grows only linearly with the number of holes, and right now I have a hard time imagening how this can work out nicely.