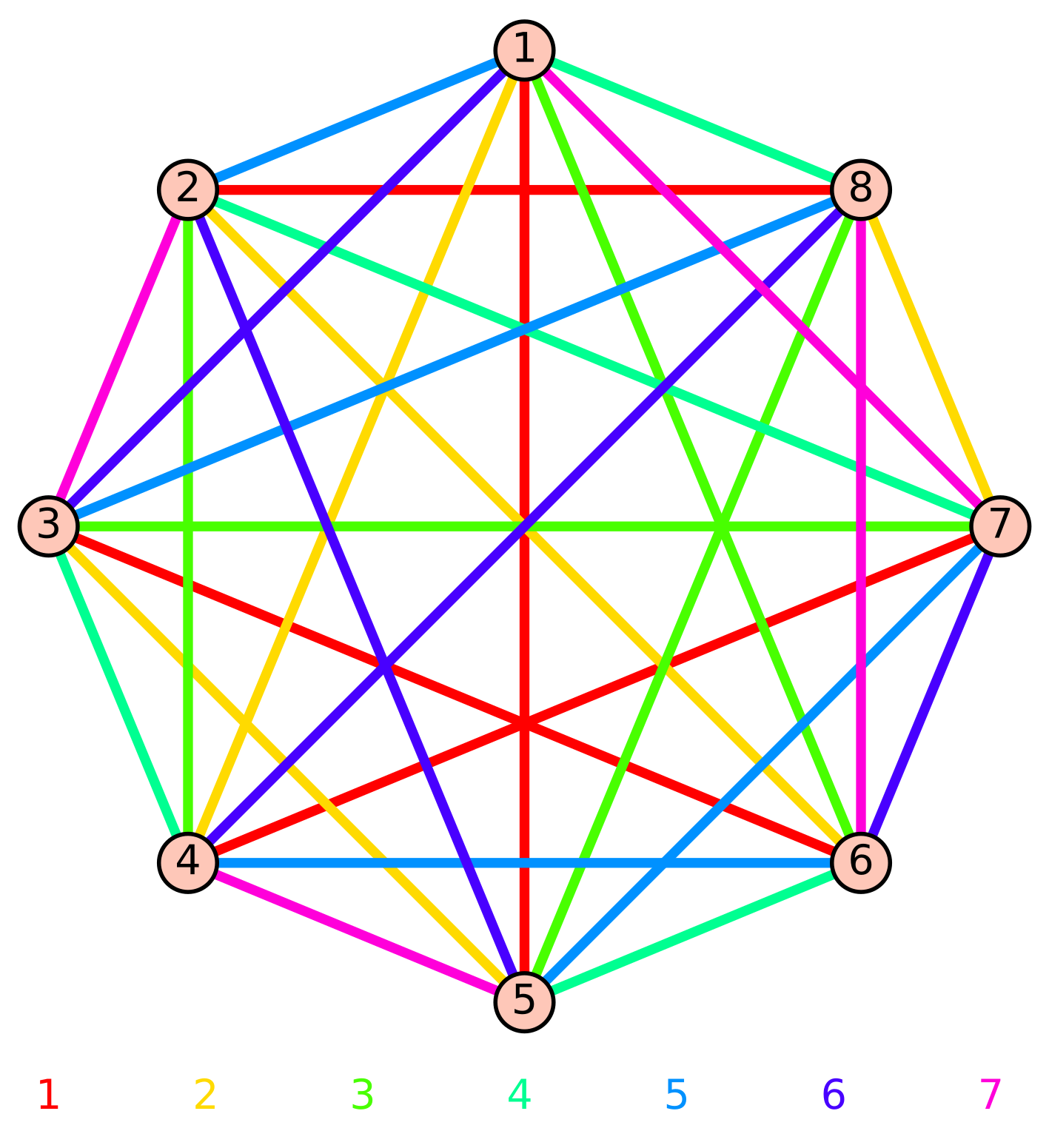
Consider coloring the edges of a complete graph on even order. This can be seen as the completion of an order $n$ symmetric Latin square except the leading diagonal. My question pertains to whether we can always complete the edge coloring in $n-1$ colors given a certain set of colors? The number of colors I fix is exactly equal to $\frac{(k)(k+2)}{2}$, where $k=\frac{n}{2}$ and form $4$ distinct consecutive last four subdiagonals (and, by symmetry, superdiagonals) in the partial Latin square.
For example, in the case of $K_8$, I fix the following colors: \begin{bmatrix}X&&&&1&3&7&4\\&X&&&&2&4&1\\&&X&&&&3&5\\&&&X&&&&6\\1&&&&X&&&\\3&2&&&&X&&\\7&4&3&&&&X&\\4&1&5&6&&&&X\end{bmatrix}
A completion to a proper edge coloring in this case would be:
\begin{bmatrix}X&5&6&2&1&3&7&4\\5&X&7&3&6&2&4&1\\6&7&X&4&2&1&3&5\\2&3&4&X&7&5&1&6\\1&6&2&7&X&4&5&3\\3&2&1&5&4&X&6&7\\7&4&3&1&5&6&X&2\\4&1&5&6&3&7&2&X\end{bmatrix}
Can the above be always done if the colors I fix follow the same pattern for all even order complete graphs? Note that the pattern followed in the precoloring consists of two portions-
i) the last $k-1$ subdiagonals are actually taken from a canonical $n$-edge coloring of the complete graph on $n-1$ vertices, where $n$ is even. By canonical, I mean the commutative idempotent 'anti-circulant' latin square. Like in the example above, the canonical coloring of the complete graph on $7$ vertices is \begin{bmatrix}1&5&2&6&3&7&4\\5&2&6&3&7&4&1\\2&6&3&7&4&1&5\\6&3&7&4&1&5&2\\3&7&4&1&5&2&6\\7&4&1&5&2&6&3\\4&1&5&2&6&3&7\end{bmatrix} ii)The $k$-th subdiagonal just consists of entries in the pattern $1-2-3-$ so on and takes into account the previous entries to create an appropriate entry. Like in the example above the last diagonal I took was $1-2-3-6$. It could also have been $1-2-3-7$.
And, if the completion exists, would the completion be unique? Any hints? Thanks beforehand.