Local Hölder continuity

Question

Assume that $f:[0,1]\to [0,1]$ is Hölder continuous with the constant $1/3$ and assume that for every $s\in [0,1]$ we have $$\limsup_{x\to s}\frac{\lvert f(x)-f(s)\rvert}{\lvert x-s\rvert^{1/2}}<\infty.$$ Does this imply that $$\sup_{x\neq s}\frac{\lvert f(x)-f(s)\rvert}{\lvert x-s\rvert^{1/2}}<\infty?$$

Answer 1

$\newcommand{\de}{\delta}$ $\newcommand{\fl}{\lfloor1/x\rfloor}$ The answer is no. E.g., consider the function $f$ defined by the conditions that $f(0):=0$ and $$f(x)=\frac{(x-x_{n+1})^{1/2}(x_n-x)} {(x_n-x_{n+1})^{7/6}} $$ for each natural $n$ and all $x\in[x_{n+1},x_n]$, where $x_n:=1/n$, so that $n=\fl$.

---

Details: Let \begin{equation} \de_n:=[x_{n+1},x_n],\quad h_n:=x_n-x_{n+1}=\frac1{n(n+1)}, \end{equation} \begin{equation} g(x):=g_n(x):=(x-a)^{1/2}(b-x),\quad a:=a_n:=x_{n+1},\quad b:=b_n:=x_n, \end{equation} $AB\vee CD:=\max(AB,CD)$. Hence, \begin{equation} f(x)=\frac{g_n(x)}{h_n^{7/6}}=\frac{g(x)}{h_n^{7/6}} \end{equation} for each natural $n$ and all $x\in\de_n$. Next, for any $x,y,h$ such that $a\le x\le y=x+h\le b$ we have \begin{align} |g(y)-g(x)|&=|(y-a)^{1/2}(b-x)-(x-a)^{1/2}(b-x) \\ &+(y-a)^{1/2}(b-y)-(y-a)^{1/2}(b-x)| \\ &=\big|(b-x)\big((y-a)^{1/2}-(x-a)^{1/2}\big)-(y-a)^{1/2}h\big| \\ &\le(b-x)\big((y-a)^{1/2}-(x-a)^{1/2}\big)\vee(y-a)^{1/2}h \\ &\le(b-x)h^{1/2}\vee(y-a)^{1/2}h \\ &\le(b-a)h^{1/2}\vee(b-a)^{1/2}h \\ &=(b-a)h^{1/2}=h_n(y-x)^{1/2}, \end{align} so that $f$ is locally $1/2$-Hölder continuous on $(0,1]$ and, moreover, $|f(y)-f(x)|\le h_n^{-1/6}(y-x)^{1/2}\le(y-x)^{1/3}$ and hence \begin{equation} |f(y)-f(x)|\le(y-x)^{1/3}, \tag{*} \end{equation} for any $x,y$ such that $x_{n+1}\le x\le y\le x_n$.

If now $x\in\de_m$ and $y\in\de_n$ for some natural $m$ and $n$ such that $m>n$, then $x_{m+1}\le x\le x_m\le x_{n+1}\le y\le x_n$ and \begin{align} |f(y)-f(x)|&\le f(x)\vee f(y) \\ &=(f(x)-f(x_m))\vee(f(y)-f(x_{n+1})) \\ &\le(x_m-x)^{1/3}\vee(y-x_{n+1})^{1/3}\le(y-x)^{1/3}. \end{align}

So, (*) holds for all $x,y$ such that $0<x\le y\le1$, that is, $f$ is $1/3$-Hölder continuous on $(0,1]$.

Next, for each natural $n$ and all $x\in\de_n$ \begin{equation} 0\le f(x)\le\frac{2(b-a)^{1/3}}{3\sqrt3}\le\frac{(b-a)^{1/3}}2=\frac1{2n^{1/3}(n+1)^{1/3}} \le\frac1{(n+1)^{2/3}}\le x^{2/3}\le x^{1/2}\le x^{1/3}. \end{equation} So, recalling that $f$ is locally $1/2$-Hölder continuous on $(0,1]$ and $1/3$-Hölder continuous on $(0,1]$, we conclude that $f$ is locally $1/2$-Hölder continuous on $[0,1]$ and $1/3$-Hölder continuous on $[0,1]$; moreover, it follows that $0\le f\le1$ on $[0,1]$.

However, $f$ is not $1/2$-Hölder continuous on $[0,1]$, because \begin{equation} \frac{f(a_n+h_n/2)-f(a_n)}{(h_n/2)^{1/2}}=h_n^{-1/6}/2\to\infty \end{equation} as $n\to\infty$, which fully confirms the "no" answer.

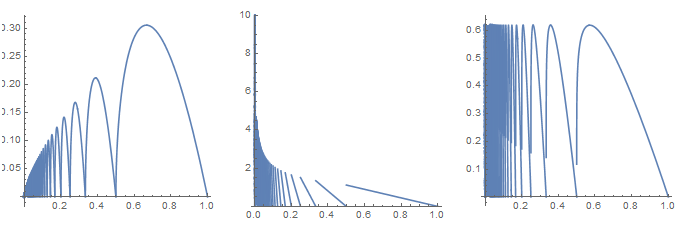
The graphs of the functions $x\mapsto f(x)$, $x\mapsto f(x)/(x-x_{n+1})^{1/2}$, $x\mapsto f(x)/(x-x_{n+1})^{1/3}$ with $n=\fl$ are shown here, left-to-right, respectively: