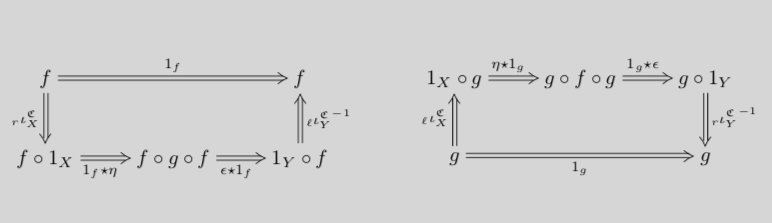4
$\begingroup$

Is the notion of an adjunction well defined in an arbitrary weak $2$-category?

In particular, it seems like we need at least invertible unitors in the ambient $2$-category to write down the triangle identities. For a pair of antiparallel arrows $f:X\leftrightarrows Y:g$ in a $2$-category $\mathfrak{C}$ with unit $\eta:1_X\Rightarrow g\circ f$ and counit $\epsilon:f\circ g\Rightarrow1_Y$ we would like to write the triangle identities as follows

fake triangle identities,

and as seen on the nLab entry for adjunctions, but in a weak $2$-category this diagram is incorrect. We've omitted the units and unitors:

real identities baby

In a partially weak $2$-category with invertible unitors this is okay, but in a fully weak $2$-category the unitors aren't invertible so the above diagrams are still wrong -- can we coherently define adjunctions inside a fully weak $2$-category?

At the $2$-categorical level we know that a fully weak $2$-category is equivalent to a fully strict one, and I suspect this allows for a workaround. At the $3$-categorical level we can only strictify $2$ of $3$ notions among associativity, units and interchange, so if a workaround exists at the $2$-categorical level we can probably strictify units in the equivalent weak $3$-category and repeat the solution one dimension up. At dimension $4$ and beyond we aren't certain about weakness being equivalent to strictness, so a workaround not using the strictification process would apply more generally with current technology.

The above is incorrect, thanks to Todd and Kevin for pointing this out. I do not know if fully weak $2$-categories in the above sense are equivalent to their categories of presheaves under $2$-Yoneda or not.

Improve this question
$\endgroup$
16
  • $\begingroup$ Are you well aware that this "fully weak" or lax notion is quite rarely studied compared to the invertible unitor/associator case? You mention the equivalence with strict 2-categories as if it were very well known, but I think it's rather rarely considered in this generality. $\endgroup$
    – Kevin Carlson
    Commented Oct 5, 2019 at 6:46
  • $\begingroup$ @KevinCarlson I thought it was standard knowledge, my apologies. For any interested readers, the equivalence is still given by the $2$-Yoneda embedding of a weak $2$-category into its $2$-presheaf $2$-category, which is strict since the codomain of $2$-presheaves is the $2$-category of categories which is strict and composition etc. is defined pointwise in the codomain for $2$-functor $2$-categories. $\endgroup$
    – Alec Rhea
    Commented Oct 5, 2019 at 7:28
  • 2
    $\begingroup$ In the argot I know, a weak $n$-category refers to the sequence set, category, bicategory, tricategory, etc., and so a weak 2-category would be a bicategory (although it may be rare these days to hear it referred to as such). There may be lax bicategories, where the one-object case is sometimes called a skew monoidal category. But I thought the Yoneda lemma applied to bicategories (?). Do you have a literature reference for your last paragraph? $\endgroup$
    – Todd Trimble
    Commented Oct 5, 2019 at 12:31
  • $\begingroup$ @ToddTrimble I'm working my way through "Coherence in Three Dimensional Category Theory" by Nick Gurski, and in the "Bicategorical Conventions" section in chapter one uses fully weak $2$-categories in the above sense. $\endgroup$
    – Alec Rhea
    Commented Oct 5, 2019 at 13:47
  • 1
    $\begingroup$ I don't see a problem considering a construction like $yB = \text{LaxBiCat}(B, Cat)$, but the exact features would be something to work out. I'll bet Nick Gurski could tell you more. But I do have a hard time believing that a notion of equivalence by which lax constraints are rendered 'pseudo' would be deserving of the name 'equivalence'. $\endgroup$
    – Todd Trimble
    Commented Oct 5, 2019 at 17:12

0

Reset to default

You must log in to answer this question.